Which two are closest to one another in size ?
Li+andNa+
Be+2andMg+2
Be+2andLi−
Li+andMg+2
The correct Answer is:D
To solve the question of which two ions are closest to one another in size, we can follow these steps:
Step 1: Identify the Ions
We are given four pairs of ions to compare:
1. Li⁺ and Na⁺
2. Be²⁺ and Mg²⁺
3. Be²⁺ and Li⁻
4. Li⁺ and Mg²⁺
Step 2: Understand Ionic Sizes
Ionic size is influenced by several factors, including the charge of the ion and the number of electrons. Generally, cations (positively charged ions) are smaller than their parent atoms because they lose electrons, leading to a decrease in electron-electron repulsion and a greater effective nuclear charge on the remaining electrons.
Step 3: Compare the Sizes of Ions
To determine which ions are closest in size, we can look up or recall the ionic radii of the ions involved:
- Li⁺: Approximately 76 picometers (pm)
- Na⁺: Approximately 102 pm
- Be²⁺: Approximately 31 pm
- Mg²⁺: Approximately 72 pm
- Li⁻: Approximately 152 pm
Step 4: Analyze the Sizes
Now, we can analyze the sizes:
- Li⁺ (76 pm) vs. Na⁺ (102 pm): Difference of 26 pm
- Be²⁺ (31 pm) vs. Mg²⁺ (72 pm): Difference of 41 pm
- Be²⁺ (31 pm) vs. Li⁻ (152 pm): Difference of 121 pm
- Li⁺ (76 pm) vs. Mg²⁺ (72 pm): Difference of 4 pm
Step 5: Conclusion
From the analysis, we see that the closest pair in size is:
- Li⁺ (76 pm) and Mg²⁺ (72 pm) with a difference of only 4 pm.
Thus, the answer to the question is:
Li⁺ and Mg²⁺ are closest to one another in size.
Final Answer
The correct option is: Li⁺ and Mg²⁺.
---
We are given four pairs of ions to compare:
1. Li⁺ and Na⁺
2. Be²⁺ and Mg²⁺
3. Be²⁺ and Li⁻
4. Li⁺ and Mg²⁺
Ionic size is influenced by several factors, including the charge of the ion and the number of electrons. Generally, cations (positively charged ions) are smaller than their parent atoms because they lose electrons, leading to a decrease in electron-electron repulsion and a greater effective nuclear charge on the remaining electrons.
To determine which ions are closest in size, we can look up or recall the ionic radii of the ions involved:
- Li⁺: Approximately 76 picometers (pm)
- Na⁺: Approximately 102 pm
- Be²⁺: Approximately 31 pm
- Mg²⁺: Approximately 72 pm
- Li⁻: Approximately 152 pm
Now, we can analyze the sizes:
- Li⁺ (76 pm) vs. Na⁺ (102 pm): Difference of 26 pm
- Be²⁺ (31 pm) vs. Mg²⁺ (72 pm): Difference of 41 pm
- Be²⁺ (31 pm) vs. Li⁻ (152 pm): Difference of 121 pm
- Li⁺ (76 pm) vs. Mg²⁺ (72 pm): Difference of 4 pm
From the analysis, we see that the closest pair in size is:
- Li⁺ (76 pm) and Mg²⁺ (72 pm) with a difference of only 4 pm.
Li⁺ and Mg²⁺ are closest to one another in size.
The correct option is: Li⁺ and Mg²⁺.
Topper's Solved these Questions
- BOOK - ALLENCHAPTER - INORGANIC CHEMISTRYEXERCISE - PERIODIC TABLE (MATCH THE COLUMN TYPE QUESTIONS)5videos
INORGANIC CHEMISTRY
- BOOK - ALLENCHAPTER - INORGANIC CHEMISTRYEXERCISE - CHEMICAL BONDING119videos
INORGANIC CHEMISTRY
- BOOK - ALLENCHAPTER - HYDROGENEXERCISE - EXERCISE-517videos
HYDROGEN
- BOOK - ALLENCHAPTER - Ionic EquilibriumEXERCISE - All Questions37videos
Ionic Equilibrium
Similar Questions
Knowledge Check
- Question 1 - Select One
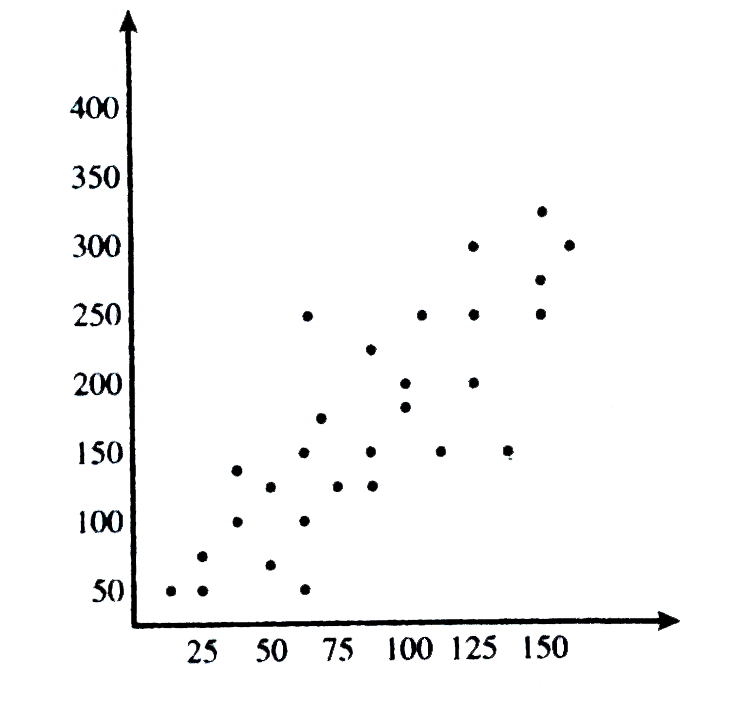
For the scatterplot above , which is closest to the slope of the line of best fit ?A12B1C2D3 - Question 2 - Select One
What is the minimum number of tiles of size 16 by 24 required to form a square by placing the tiles adjacent to one another other?
A6B8C11D16
Vascular bundles in which xylem and phloem surround one another is called
View SolutionThe locus of the point of Interection of two tangents to the hyperbola x2a2−y2b2=1 which a make an angle 60∘ with one another is
View SolutionFind the locus of thepoint of intersection of two normals to a parabolas which are at right angles to one another.
View SolutionFind the locus of thepoint of intersection of two normals to a parabolas which are at right angles to one another.
View SolutionThe radius of which is closest to that of the Li+ ions?
View SolutionShow that if two chords of a circle bisect one another they must be diameters.
View Solution

