How many kinds of gametes can be produced by an individual of genotype AaBbCcDdeeFf
32
16
8
4
The correct Answer is:A
To determine how many kinds of gametes can be produced by an individual with the genotype (A)/(a)(B)/(b)(C)/(c)(D)/(d)(e)/(e)(F)/(f), we can follow these steps:
1. Identify the Genotype: Write down the complete genotype.
- The genotype is: A/a,B/b,C/c,D/d,e/e,F/f.
2. Classify Alleles: Determine which alleles are heterozygous and which are homozygous.
- Heterozygous alleles: A/a,B/b,C/c,D/d,F/f
- Homozygous alleles: e/e (this pair will not contribute to gamete diversity).
3. Count the Heterozygous Alleles: Count the number of heterozygous pairs.
- There are 5 heterozygous pairs: A/a,B/b,C/c,D/d,F/f.
4. Apply the Formula for Gametes: Use the formula 2n, where n is the number of heterozygous pairs.
- Here, n=5.
- Therefore, the number of different gametes is 25.
5. Calculate the Result: Compute 25.
- 25=32.
Final Answer:
The individual can produce 32 different kinds of gametes.
---
- The genotype is: A/a,B/b,C/c,D/d,e/e,F/f.
- Heterozygous alleles: A/a,B/b,C/c,D/d,F/f
- Homozygous alleles: e/e (this pair will not contribute to gamete diversity).
- There are 5 heterozygous pairs: A/a,B/b,C/c,D/d,F/f.
- Here, n=5.
- Therefore, the number of different gametes is 25.
- 25=32.
The individual can produce 32 different kinds of gametes.
Topper's Solved these Questions
Similar Questions
If in two triangles ABC and DEF , ∠A=∠E, ∠B=∠F , then which of the following is not true? BCDF=ACDE (b) ABDE=BCDF (c) ABEF=ACDE (d) BCDF=ABEF
View SolutionIf D,EandF are three points on the sides BC,CAandAB, respectively, of a triangle ABC show that the BDCD=CEAE=AFBF=−1
View SolutionIf ABC and DEF are two triangles such that ABDE=BCEF=CAFD=25 , then Area(ABC):Area(DEF)=
View SolutionIf ABC and DEF are two triangles such that ABDE=BCEF=CAFD=34 , then write Area (ABC):Area(DEF) .
View SolutionIn Figure, ABCDE is a pentagon. A line through B parallel to AC meets DC produced at F. Show that:
(i) ar (ACB)=ar (ACF)
(ii) ar (AEDF)=ar (ABCDE)
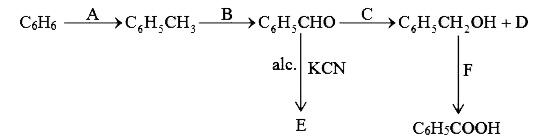
View SolutionIdentify the compounds A, B, C, D, E and F.
View SolutionIf in two triangles ABC and DEF , ABDE=BCFE=CAFD , then FDECAB (b) FDEABC (c) CBAFDE (d) BCAFDE
View SolutionIn Fig. if EF||DC||AB. Prove that AEED=BFFC . (FIGURE)
View SolutionIn Fig. if EF||DC||AB. Prove that AEED=BFFC . (FIGURE)
View SolutionIn Figure, ABCDE is a pentagon. A line through B parallel to AC meets DC produced at F. Show that: (i) ar(△ACB)=ar(△ACF) (ii) ar(quadrilateralAEDF)=ar(ABCDE)
View Solution
