The small particle of mass m is given an initial high velocity in the horizontal plane and winds its cord around the fixed vertical shaft of radius 1m. All motion occurs essentially in the horizontal plane. If the angular velocity of the cord is 0.8 rad/s when the distance from the particle to the tangency point is 5m, determine the angular velocity ω (in rad//s) of the cord after it has turned through an angle 1 rad.
The correct Answer is:1
To solve the problem step by step, we will analyze the motion of the particle as it winds the cord around the vertical shaft.
Step 1: Understand the Initial Conditions
- The initial angular velocity of the cord, ω0=0.8rad/s.
- The initial distance from the particle to the tangency point (radius of the circular path), R=5m.
- The radius of the shaft, a=1m.
- The angle through which the cord has turned, θ=1rad.
Step 2: Relate Linear and Angular Velocity
The linear velocity V of the particle can be expressed in terms of the angular velocity and radius:
V=ω0⋅R
Substituting the known values:
V=0.8rad/s⋅5m=4m/s
Step 3: Determine the New Radius After the Cord Turns
After the cord has turned through an angle θ=1rad, the new radius R′ can be calculated as:
R′=R−a⋅θ
Substituting the known values:
R′=5m−1m⋅1rad=5m−1m=4m
Step 4: Calculate the New Angular Velocity
The new angular velocity ω can be found using the relationship between linear velocity and the new radius:
ω=VR′
Substituting the values we have:
ω=4m/s4m=1rad/s
Final Answer
The angular velocity ω of the cord after it has turned through an angle of 1 radian is:
ω=1rad/s
---
- The initial angular velocity of the cord, ω0=0.8rad/s.
- The initial distance from the particle to the tangency point (radius of the circular path), R=5m.
- The radius of the shaft, a=1m.
- The angle through which the cord has turned, θ=1rad.
The linear velocity V of the particle can be expressed in terms of the angular velocity and radius:
V=ω0⋅R
Substituting the known values:
V=0.8rad/s⋅5m=4m/s
After the cord has turned through an angle θ=1rad, the new radius R′ can be calculated as:
R′=R−a⋅θ
Substituting the known values:
R′=5m−1m⋅1rad=5m−1m=4m
The new angular velocity ω can be found using the relationship between linear velocity and the new radius:
ω=VR′
Substituting the values we have:
ω=4m/s4m=1rad/s
The angular velocity ω of the cord after it has turned through an angle of 1 radian is:
ω=1rad/s
Similar Questions
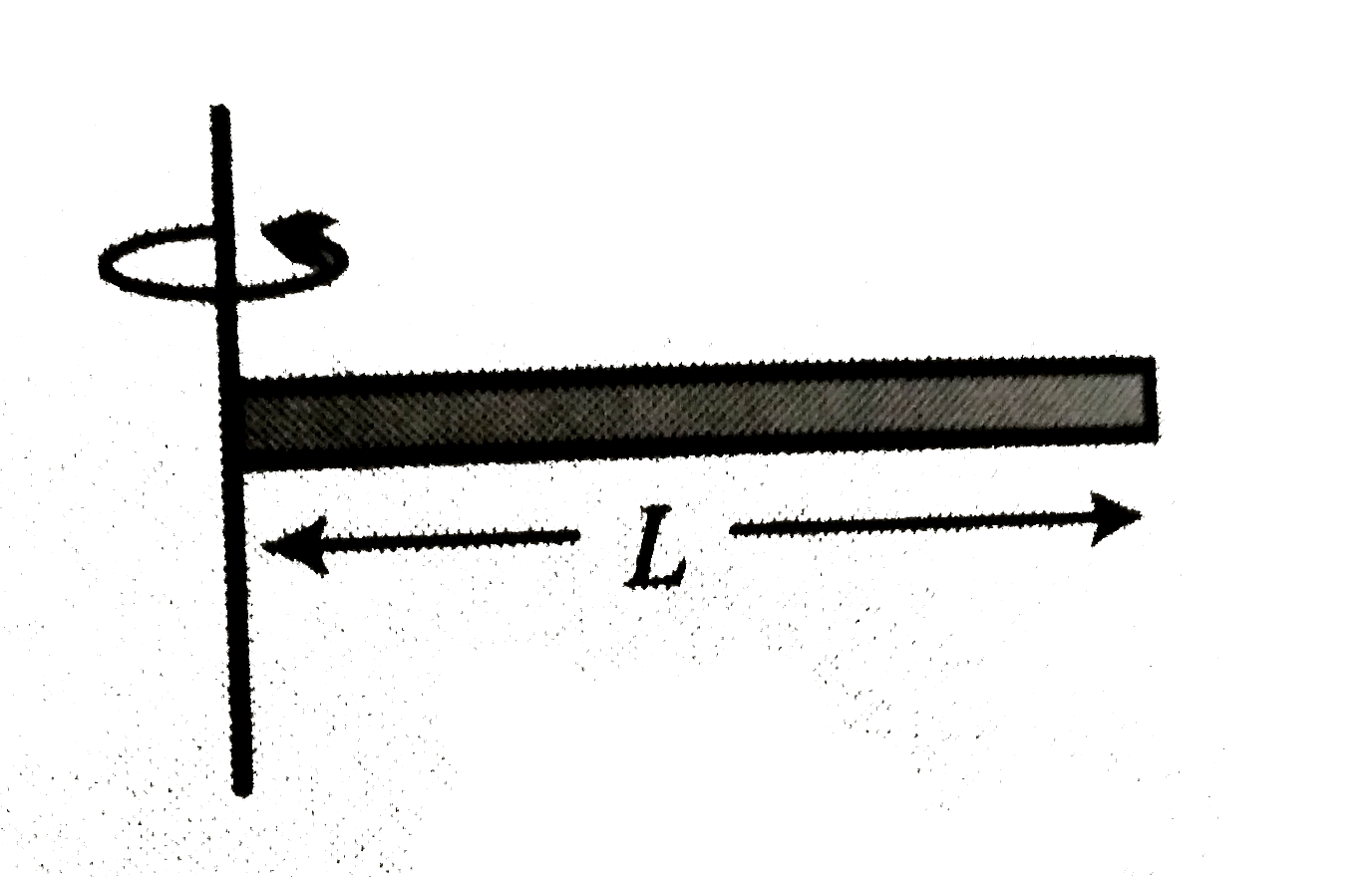
A uniform of mass m and length L is tied to a vertical shaft. It rotates in horizontal plane about the vertical axis at angular velocity ω. How much horizontal force does the shaft exert on the rod?
 View Solution
View SolutionA particle is moving along a circle of radius 20cm, with a linear velocity of 2m//s. Calculate the angular velocity of the particle.
View SolutionA particle is moving along a circle of radius 20cm, with a linear velocity of 2m//s. Calculate the angular velocity of the particle.
View SolutionA uniform rod of mass m and length l rotates in a horizontal plane with an angular velocity ω about a vertical axis passing through one end. The tension in the rod at a distance x from the axis is
View SolutionThe angular momentum of a body with mass (m) moment of inertia (I) and angular velocity (ω) rad/s is equal to
View SolutionA ball is projected from a point in a horizontal plane with a velocity of 20 m/s at 30∘ with the horizontal. Coefficient of restitution is 0.8, then
View SolutionIf 2 kg mass is rotating on a circular path of radius 0.8 m with angular velocity of 44 rad/sec. If radius of the parth becomes 1 m, then what will be the value of angular velocity?
View SolutionA particle of mass m is projected with a velocity v at an angle of θ with horizontal. The angular momentum of the particle at the highest point of its trajectory is equal to :
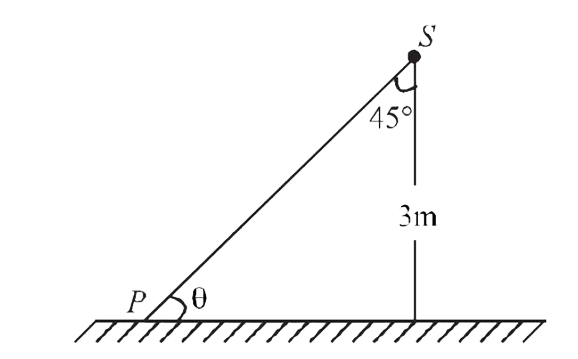
View SolutionA spot light S rotates in a horizontal plane with a constant angular velocity of 0.1rad/s. The spot of light P move along the wall at a disatnce 3m. What is the velocity of the spot P when θ=45∘ ?
View SolutionSpotlight S rotates in a horizontal plane with constant angular velocity of 0.1 rad/s The spot of light P moves along the wall at a distance of 3 m The velocity of the spot P when θ = 45 is m/s (see - fig. ) is ……….. m/s
 View Solution
View Solution

