Question

Graphical soluton of a two body head on collision
A block $A$ of mass $m$ moving with a uniform velocity $v_0$ strikes another identical block $B$ kept at rest on a horizontal smooth surface as shown in the figure (i). We can conserve linear momentum.

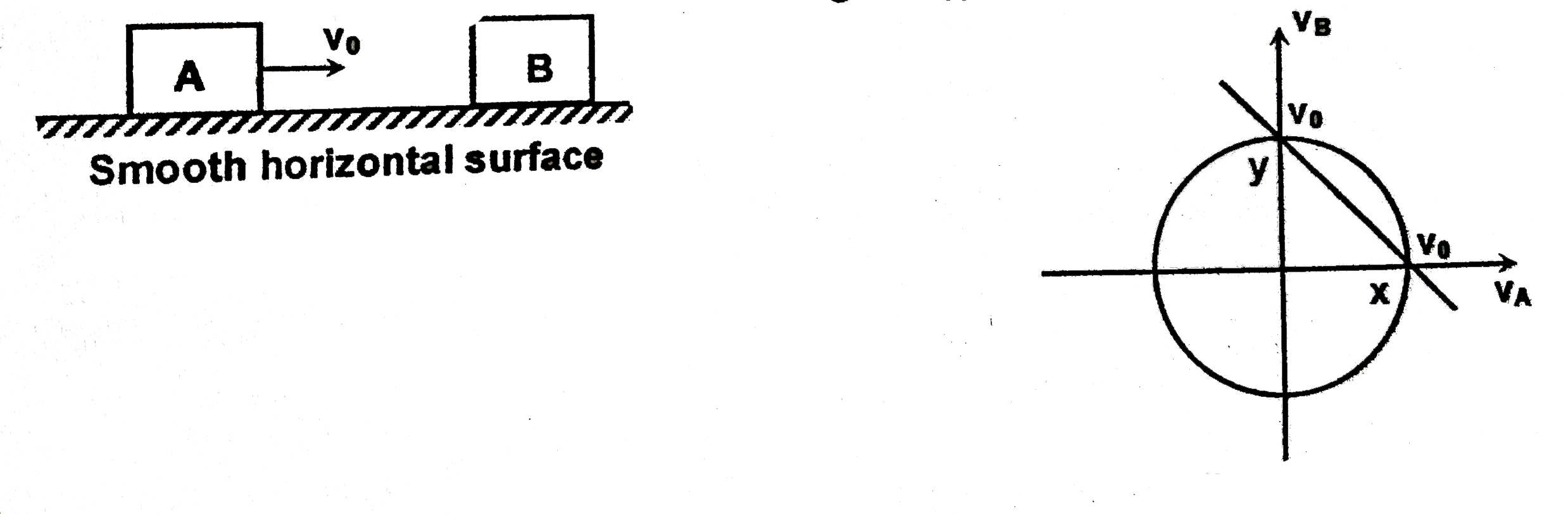
So $m{v}_0=m{v}_{A}m{v}_B$ ($v_A$ and $v_B$ are the velocities of the blocks after collision)
$\therefore v_0=v_A+v_B$.........(i)
If the collision is perfectly elastic
$\frac{1}{2}m{v}_0^2=\frac{1}{2}m{v}_A^2+\frac{1}{2}m{v}_B^2$
$\Rightarrow v_0^2=v_A^2+v_B^2$......(ii)
Both the above equation (i) and (ii) are plotted on $v_A-v_B$ plane as shown in figure (ii). This plot can be used to find the unknowns $v_A$ and $v_B$.
For example the solution of the situation in figure (i) is $v_A=0,v_B=v_0$ (point $y$ in the plot)
Because $v_A=v_0,v_B=0$ (point $x$ in the plot) is not physically possible.
In a situation block $A$ is moving with velocity $2m/s$ an strikes another identical block $B$ kept at rest. The $v_A-v_B$ plot for the situation is shown. $m$ and $l$ are the intersection points whose $v_A,v_B$ coordinaes are given in the figure. The coefficient of restitution of the collision is

Options

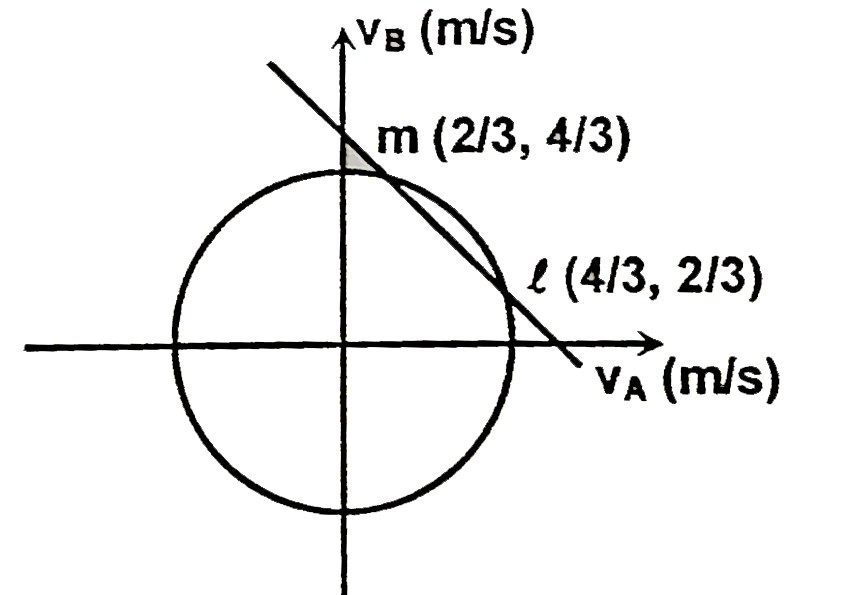
A. `1/2`
B. `1//3`
C. `1`
D. Collision no possible

Answer

B

Explanation

`e=(4/3-2/3)/2=1/3`