A 200cm length of the wire weighs 0.6 gm. If the tension in the wire caused by a 500gm mass hanged from it. If the approximate wavelength of a wave of frequency 400Hz sent down by it is 2n×10−2m. Find the value of n (g=9.8m/s2)
The correct Answer is:5
To solve the problem step by step, we will follow these calculations:
Step 1: Convert the weight of the wire to mass per unit length (μ)
The weight of the wire is given as 0.6 grams, and the length of the wire is 200 cm.
1. Convert the weight to kilograms:
Weight=0.6 g=0.6×10−3 kg
2. Convert the length to meters:
Length=200 cm=2 m
3. Calculate the mass per unit length (μ):
μ=masslength=0.6×10−3 kg2 m=3×10−4 kg/m
Step 2: Calculate the tension (T) in the wire
The tension in the wire is caused by a mass of 500 g hanging from it.
1. Convert the mass to kilograms:
Mass=500 g=0.5 kg
2. Use the formula for tension:
T=mg=0.5 kg×9.8 m/s2=4.9 N
Step 3: Calculate the wave speed (v) in the wire
The wave speed can be calculated using the formula:
v=√Tμ
Substituting the values:
v=√4.9 N3×10−4 kg/m
Calculating this gives:
v=√16333.33≈128 m/s
Step 4: Calculate the wavelength (λ) of the wave
The wavelength can be calculated using the formula:
λ=vf
Where f=400 Hz:
λ=128 m/s400 Hz=0.32 m=32×10−2 m
Step 5: Express the wavelength in the form 2n×10−2
We need to express 32×10−2 in the form 2n×10−2.
1. Recognize that 32=25:
32×10−2=25×10−2
2. Therefore, comparing with 2n×10−2, we find:
n=5
Final Answer
The value of n is 5.
---
The weight of the wire is given as 0.6 grams, and the length of the wire is 200 cm.
Weight=0.6 g=0.6×10−3 kg
Length=200 cm=2 m
μ=masslength=0.6×10−3 kg2 m=3×10−4 kg/m
The tension in the wire is caused by a mass of 500 g hanging from it.
Mass=500 g=0.5 kg
T=mg=0.5 kg×9.8 m/s2=4.9 N
The wave speed can be calculated using the formula:
v=√Tμ
v=√4.9 N3×10−4 kg/m
v=√16333.33≈128 m/s
The wavelength can be calculated using the formula:
λ=vf
λ=128 m/s400 Hz=0.32 m=32×10−2 m
We need to express 32×10−2 in the form 2n×10−2.
32×10−2=25×10−2
n=5
The value of n is 5.
Similar Questions
A steel wire of mass 4.0 g and length 80 cm is fixed at the two ends. The tension in the wire is 50 N. Find the frequency and wavelength of the fourth harmonic of the fundamental.
View SolutionVelocity of a wave in a wire is v when tension in it is 2.06×104N. Find value of tension in wire when velocity of wave become v2.
View Solution
Knowledge Check
- Question 1 - Select One
A 10 m long steel wire has mass 5 g. If the wire is under a tension of 80 N, the speed of transverse waves on the wire is
A100ms−1B200ms−1C400ms−1D500ms−1
Velocity of a wave in a wire is v when tension in it is 2.06×104N. Find value of tension in wire when velocity of wave become v2.
View SolutionA steel wire 100 cm long has a mass of 10 gm. If the wire is under a tension of 400 N, what is the speed of transverse waves in the wire?
View SolutionA steel wire is rigidly fixed at both ends. Its length mass and cross-sectionl area are 1m, 0.1kg and 10−8m2 respectively. Tension in the wire is produced by lowering the temperature by 20∘C. If the transerverse waves are some up by plucking the wire at 0.25m from one end and assuming that the wire viberates with minimum number of loops possble for such a case. The frequency of viberation (in Hz) is found to be. 1.11. Find the value of K. Given α=1.21×10−5.∘C−1.Y−2×1011N/m2
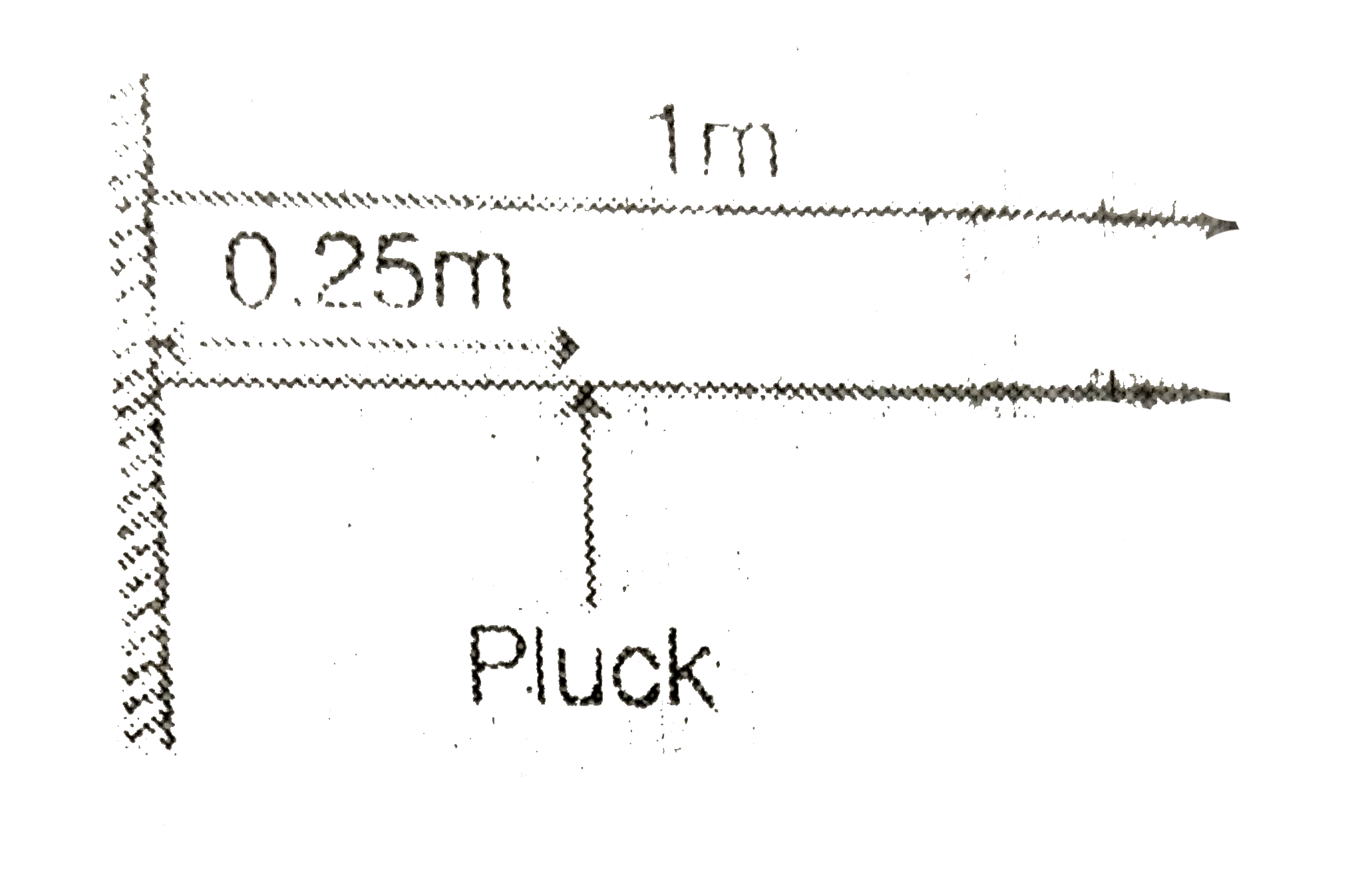 View Solution
View SolutionAn aluminium wire of cross-sectional area (10−6)m2 is joined to a steel wire of the same cross-sectional area. This compound wire is stretched on a sonometer pulled by a weight of 10kg. The total length of the compound wire between the bridges is 1.5m of which the aluminium wire is 0.6m and the rest is steel wire. Transverse vibrations are setup in the wire by using an external source of variable frequency. Find the lowest frequency of excitation for which the standing waves are formed such that the joint in the wire is a node. What is the total number of nodes at this frequency? The density of aluminium is 2.6×(103)kg/m3 and that of steel is 1.04×104kg/m2(g=10m/s2)
View SolutionThe density of the material of a wire used in sonometer is 75×10−2kg/m3. If the stress on the wire is 3.0×104N/m2, the speed of transverse wave in the wire will be
View SolutionA wire of mass 5 gram is kept stretched by a force of 400 N. When plucked at a point, transverse waves travel along the wire with a speed of 400 m/s. The length of the wire is
View SolutionA child weighing 25 kg slides down a rope hanging from a branch of a tall tree. If the force of friction acting against him is 200 N, the acceleration of child is (g=10m/s2)
View Solution

