If an electron enters a space between the plates of a parallel plate capacitor at an angle θ1 with the plates and leaves at an angle θ2 to the plates, the ratio of its kinetic energy while entering the capacitor to that while leaving will be
cos2θ2cos2θ1
cos2θ1cos2θ2
sin2θ2sin2θ1
sin2θ1sin2θ2
To solve the problem of finding the ratio of the kinetic energy of an electron while entering and leaving a parallel plate capacitor, we can follow these steps:
1. Understand the Scenario:
- An electron enters the space between the plates of a parallel plate capacitor at an angle θ1 and exits at an angle θ2.
- We need to find the ratio of its kinetic energy while entering the capacitor to that while leaving.
2. Identify Components of Velocity:
- Let v1 be the velocity of the electron when it enters the capacitor and v2 be the velocity when it exits.
- The horizontal component of the velocity remains constant because there is no horizontal force acting on the electron.
- Therefore, we can write:
v1x=v1cos(θ1)
v2x=v2cos(θ2)
- Since v1x=v2x, we have:
v1cos(θ1)=v2cos(θ2)
3. Derive the Ratio of Velocities:
- Rearranging the equation gives:
v1v2=cos(θ2)cos(θ1)
4. Kinetic Energy Formula:
- The kinetic energy KE is given by:
KE=12mv2
- Let KE1 be the kinetic energy while entering and KE2 be the kinetic energy while leaving the capacitor.
5. Write the Ratio of Kinetic Energies:
- The ratio of the kinetic energies can be expressed as:
KE1KE2=12mv2112mv22=v21v22
6. Substituting the Velocity Ratio:
- From the previous step, we substitute v1v2:
KE1KE2=(v1v2)2=(cos(θ2)cos(θ1))2
7. Final Result:
- Thus, the ratio of the kinetic energy while entering the capacitor to that while leaving is:
KE1KE2=cos2(θ2)cos2(θ1)
Conclusion:
The ratio of the kinetic energy of the electron while entering the capacitor to that while leaving is given by:
KE1KE2=cos2(θ2)cos2(θ1)
- An electron enters the space between the plates of a parallel plate capacitor at an angle θ1 and exits at an angle θ2.
- We need to find the ratio of its kinetic energy while entering the capacitor to that while leaving.
- Let v1 be the velocity of the electron when it enters the capacitor and v2 be the velocity when it exits.
- The horizontal component of the velocity remains constant because there is no horizontal force acting on the electron.
- Therefore, we can write:
v1x=v1cos(θ1)
v2x=v2cos(θ2)
- Since v1x=v2x, we have:
v1cos(θ1)=v2cos(θ2)
- Rearranging the equation gives:
v1v2=cos(θ2)cos(θ1)
- The kinetic energy KE is given by:
KE=12mv2
- Let KE1 be the kinetic energy while entering and KE2 be the kinetic energy while leaving the capacitor.
- The ratio of the kinetic energies can be expressed as:
KE1KE2=12mv2112mv22=v21v22
- From the previous step, we substitute v1v2:
KE1KE2=(v1v2)2=(cos(θ2)cos(θ1))2
- Thus, the ratio of the kinetic energy while entering the capacitor to that while leaving is:
KE1KE2=cos2(θ2)cos2(θ1)
The ratio of the kinetic energy of the electron while entering the capacitor to that while leaving is given by:
KE1KE2=cos2(θ2)cos2(θ1)
Similar Questions
IF an electron enters into a space between the plates of a parallel plate capacitor at an an angle α with the plates an leaves at an angle β to the plates find the ratio of its kinetic energy while entering the capacitor of that while leaving.
View SolutionForce of attraction between the plates of a parallel plate capacitor is
View Solution
Knowledge Check
- Question 1 - Select One
As the distance between the plates of a parallel plate capacitor decreased
AIf both assertion and reason are ture and reason is the correct explanation of assertion.BIf both assertin and reason are ture but reason is not the correct explanation of assertion .CIf assertion is true but reason is false.DIf both assertion and reason are false. - Question 2 - Select One
In a parallel plate capacitor , the capacity increases if
Aarea of the plate is decreasedBdistance between the plates increasesCarea of the plate is increasedDdielectric constant decreases.
If the plates of a parallel plate capacitor are not equal in area, then
View SolutionAn electron enters into the space of uniform field of finite width making an angle 60∘ to the field lines and leaves at angle 30∘ to the field lines. The ratio of its kinetic energy while entering the electric field to the while leave will be :
 View Solution
View SolutionTo reduce the capacitance of parallel plate capacitor, the space between the plate is
View SolutionAn electron enters the region between the plates of a parallel plate capacitor at an angle θ to the plates. The plate width is l, the plate separation is d. The electron follows the path shown just missing the upper plate. Neglect gravity. Then,
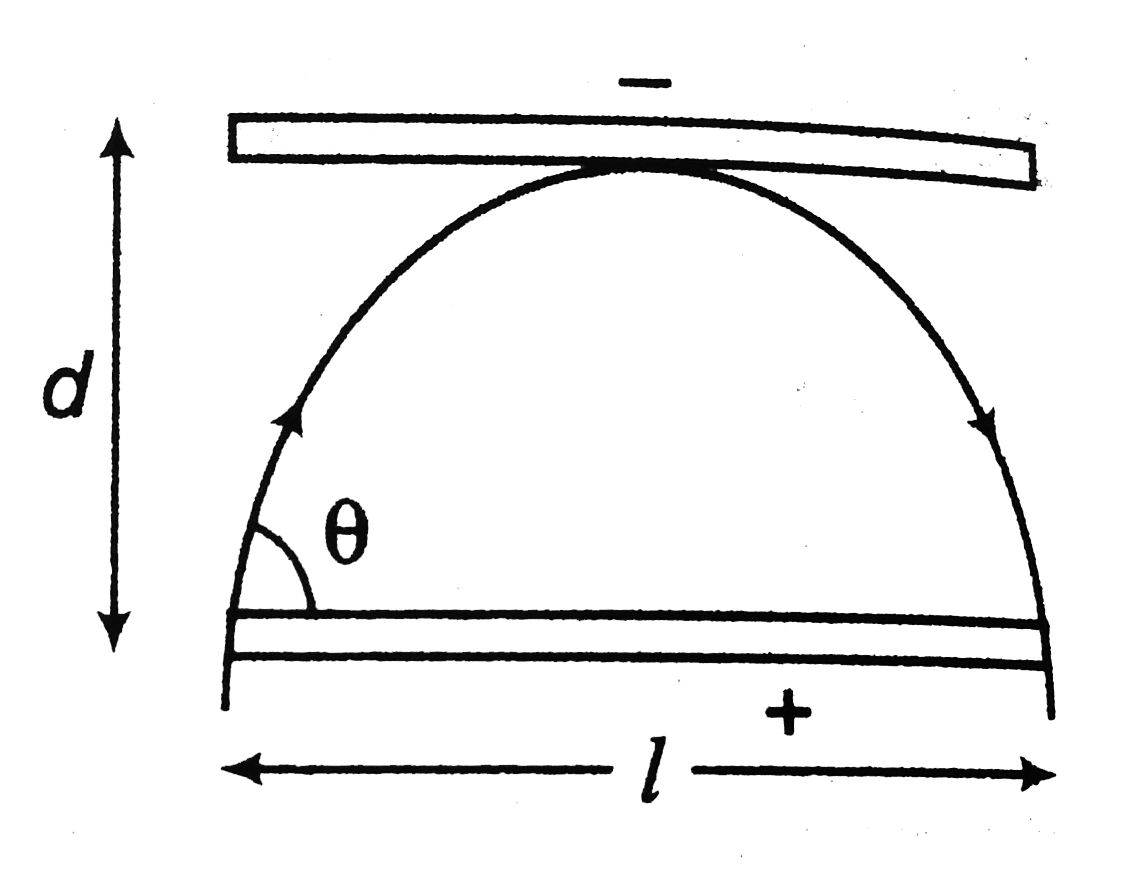 View Solution
View SolutionThe separation between the plates of a charged parallel-plate capacitor is increased. The force between the plates
View SolutionA parallel plate capacitor is charged. If the plates are pulled apart
View Solution

