An ideal choke takes a current of 8A when connected to an a.c. source of 100 volt and 50Hz. A pure resistor under the same conditions takes a current of 10A. If two are connected in series to an a.c. supply of 100V and 40Hz, then the current in the series combination of above resistor and inductor is
10A
8A
5√2amp
10√2amp
To solve the problem step by step, we will follow these calculations:
Step 1: Calculate the Inductive Reactance (XL) at 50 Hz
Given:
- Voltage (V) = 100 V
- Current through inductor (IL) = 8 A
Using the formula for inductive reactance:
XL=VIL
Substituting the values:
XL=1008=12.5Ω
Step 2: Calculate the Resistance (R) of the Resistor
Given:
- Current through resistor (IR) = 10 A
Using Ohm's law:
R=VIR
Substituting the values:
R=10010=10Ω
Step 3: Calculate the Inductance (L) of the Choke
Using the formula for inductive reactance:
XL=2πfL
Where f=50Hz. Rearranging for L:
L=XL2πf
Substituting the values:
L=12.52π×50=12.5100π=18πH
Step 4: Calculate the Inductive Reactance (XL) at 40 Hz
Now, we need to calculate XL at the new frequency f=40Hz:
XL=2πfL
Substituting the values:
XL=2π×40×18π
This simplifies to:
XL=10Ω
Step 5: Calculate the Total Impedance (Z) in Series
The total impedance in the series circuit is given by:
Z=√R2+X2L
Substituting the values:
Z=√102+102=√100+100=√200=10√2Ω
Step 6: Calculate the Current (I) in the Series Combination
Using Ohm's law, the current in the series circuit can be calculated as:
I=VZ
Substituting the values:
I=10010√2=10√2=5√2A
Final Answer
The current in the series combination of the resistor and inductor is:
5√2A
Given:
- Voltage (V) = 100 V
- Current through inductor (IL) = 8 A
XL=VIL
Substituting the values:
XL=1008=12.5Ω
Given:
- Current through resistor (IR) = 10 A
R=VIR
Substituting the values:
R=10010=10Ω
Using the formula for inductive reactance:
XL=2πfL
Where f=50Hz. Rearranging for L:
L=XL2πf
Substituting the values:
L=12.52π×50=12.5100π=18πH
Now, we need to calculate XL at the new frequency f=40Hz:
XL=2πfL
Substituting the values:
XL=2π×40×18π
This simplifies to:
XL=10Ω
The total impedance in the series circuit is given by:
Z=√R2+X2L
Substituting the values:
Z=√102+102=√100+100=√200=10√2Ω
Using Ohm's law, the current in the series circuit can be calculated as:
I=VZ
Substituting the values:
I=10010√2=10√2=5√2A
The current in the series combination of the resistor and inductor is:
5√2A
Similar Questions
An ideal choke takes a current of 8A when connectd to an AC source of 100V and 50 Hz. A pure resistor under the same condition strikes a current of 10A. If two are connected in series to an AC supply of 100V and 40Hz, then the current in the series combination of above resistor and inductor √10xA. Find value of x
View SolutionAn ideal choke takes a current of 10 A when connected to an ac supply of 125 V and 50 Hz. A pure resistor under the same conditions takes a current of 12.5 A. If the two are connected to an ac supply of 100 V and 40 Hz, then the current in series combination of above resistor and inductor is
View SolutionA resistor and an inductor are connected to an ac supply of 120 V and 50 Hz, the current in the circuit is 3 A. If the power consumed in the circuit is 108 W, then the resistance in the circuit is
View SolutionA tube light of 60V,60W rating is connected across an AC source of 100V and 50Hz frequency. Then,
View SolutionFigure below shows a capacitor C, an inductor L and a resistor R, connected in series to an a.c. supply of 220 V.
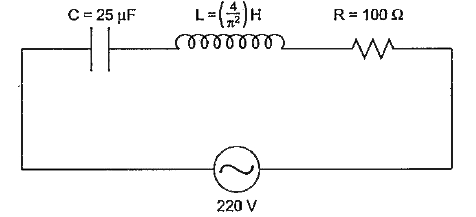
Current flowing through the circuit.
View SolutionA 0.21 H inductor and a 12Ω resistance are connected in series to a 220 V, 50 Hz ac source.
The current in the circuit is
View SolutionFigure below shows a capacitor C, an inductor L and a resistor R, connected in series to an a.c. supply of 220 V.
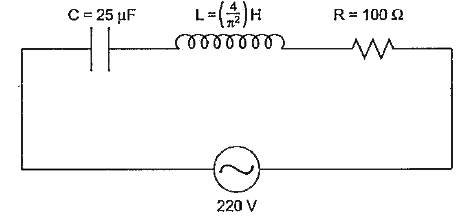
Average power consumed by the circuit.
View SolutionA 100W bulb is connected to an AC source of 220V, 50Hz. Then the current flowing through the bulb is
View SolutionA 12 Ω resistor and a 0.21 H inductor are connected in series to an a.c. source operating at V, 50 cycle second. The phase angle between current and source vottage is
View SolutionA 12 Ω resistor and a 0.21 H inductor are connected in series to an a.c. source operating at V, 50 cycle second. The phase angle between current and source vottage is
View Solution
