is small angled prism of angle made of a material of refractive index . A ray of light is incident normally to the mirror as shown in figure. is a plane mirror. The angle of deviation for the ray reflected from the mirror with respect to the incident ray is :
A.
B.
C.
D.
To solve the problem, we need to find the angle of deviation for a ray of light that passes through a small angled prism and reflects off a plane mirror. Here are the steps to find the solution:
Step 1: Understand the given values
- The angle of the prism A=3∘
- The refractive index of the prism material μ=1.5
Step 2: Calculate the angle of deviation due to the prism
The formula for the angle of deviation DP for a small angle prism is given by:
DP=(μ−1)⋅A
Substituting the known values:
DP=(1.5−1)⋅3∘=0.5⋅3∘=1.5∘
Step 3: Determine the angle of incidence at the mirror
Since the ray is incident normally to the prism, the angle of incidence at the mirror IM is equal to the angle of deviation caused by the prism:
IM=DP=1.5∘
Step 4: Calculate the angle of deviation due to the mirror
The angle of deviation DM for a plane mirror is given by:
DM=180∘−2IM
Substituting the value of IM:
DM=180∘−2⋅1.5∘=180∘−3∘=177∘
Step 5: Calculate the total angle of deviation
The total angle of deviation D is the sum of the deviation caused by the prism and the deviation caused by the mirror:
D=DP+DM=1.5∘+177∘=178.5∘
Final Answer
The angle of deviation for the ray reflected from the mirror M with respect to the incident ray is:
178.5∘
- The angle of the prism A=3∘
- The refractive index of the prism material μ=1.5
The formula for the angle of deviation DP for a small angle prism is given by:
DP=(μ−1)⋅A
DP=(1.5−1)⋅3∘=0.5⋅3∘=1.5∘
Since the ray is incident normally to the prism, the angle of incidence at the mirror IM is equal to the angle of deviation caused by the prism:
IM=DP=1.5∘
The angle of deviation DM for a plane mirror is given by:
DM=180∘−2IM
DM=180∘−2⋅1.5∘=180∘−3∘=177∘
The total angle of deviation D is the sum of the deviation caused by the prism and the deviation caused by the mirror:
D=DP+DM=1.5∘+177∘=178.5∘
The angle of deviation for the ray reflected from the mirror M with respect to the incident ray is:
178.5∘
Similar Questions
Knowledge Check
- Question 1 - Select One
The angle between the incident ray and the ray reflected from the plane mirror is 70∘. The angle of incidence will be:
A70∘B30∘C35∘D90∘
A light ray is incident normally on a plane mirror.
What is the angle of incidence?View SolutionA light ray is incident normally on a plane mirror.
What is its angle of incidence?View SolutionA ray of light is incident normally on one of the faces of a prism of apex angle 30 degree and refractive index √2. The angle of deviation of the ray in degrees is
View SolutionA prism having an apex angle 4∘ and refractive index 1.5 is located in front of a vertical plane mirror as shown in figure. Through what total angle is the ray is deviated after reflection from the mirror ?
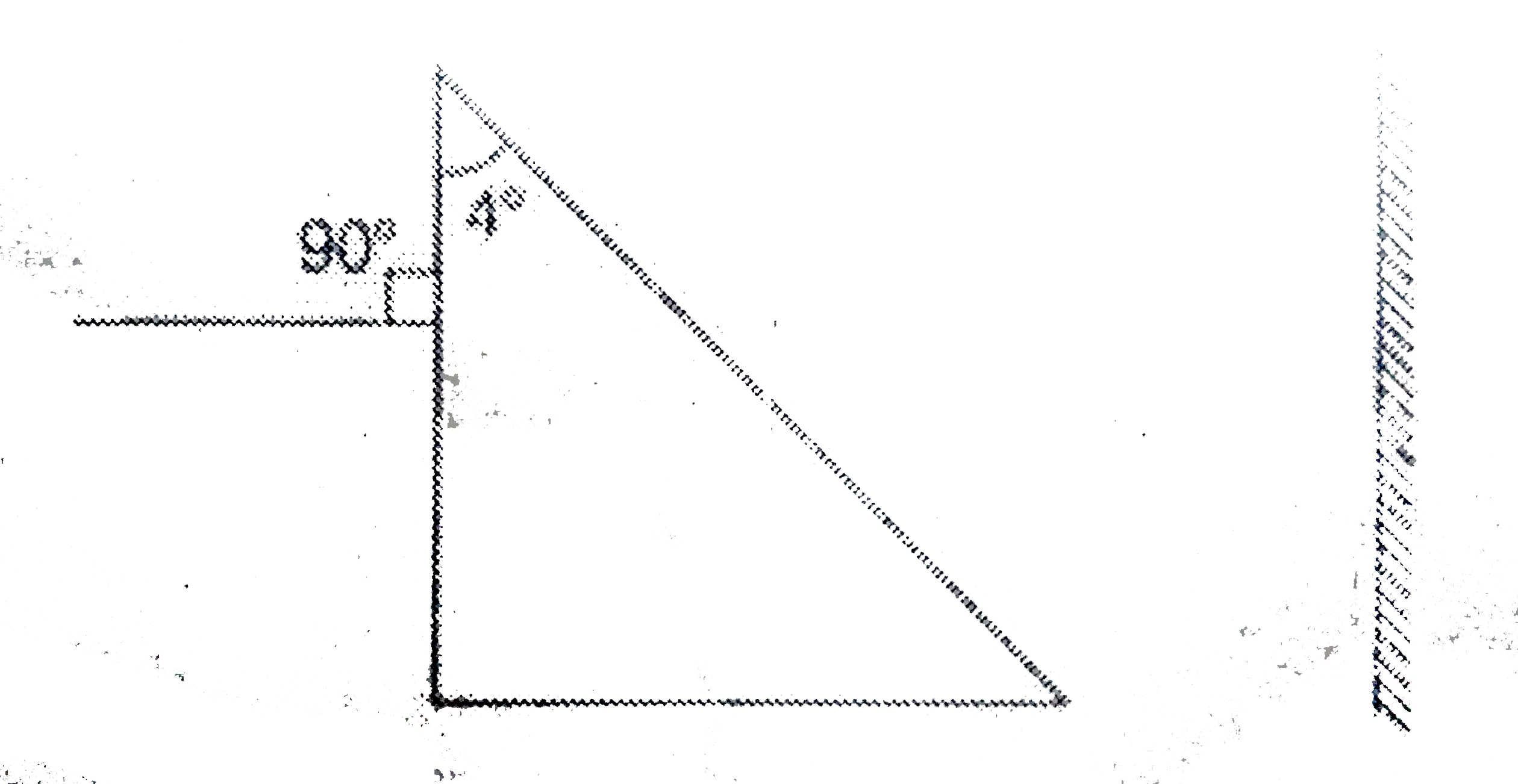 View Solution
View SolutionDraw a diagram to show the reflection of a light ray incident normally on a plane mirror.
View SolutionA ray of light falls normally on a plane mirror. What is the angle of incidence ?
View SolutionA ray of light is incident on a plane mirror at an angle of incidence of 30∘. The deviation produced by the mirror is
View Solution

