A class has 30 students. The followign prizes are to be awarded to the students of the class- first and second in mathematics, first and second in Physics, first in Chemistry and first Biology. If N denote the number of ways in which this can be done, then
N is divisible by 400
N is divisivble by 600
N is divisivble by 8100
N is divisible by 4 distinct prime No.
The correct Answer is:a,b,c,d
To solve the problem of awarding prizes to students in a class of 30, we need to calculate the total number of ways to award the prizes as specified.
1. First Prize in Mathematics:
- There are 30 students eligible for the first prize in Mathematics.
- Therefore, the number of ways to choose the first prize winner in Mathematics = 30.
2. Second Prize in Mathematics:
- After awarding the first prize, 29 students remain eligible for the second prize in Mathematics.
- Therefore, the number of ways to choose the second prize winner in Mathematics = 29.
3. First Prize in Physics:
- Again, there are 30 students eligible for the first prize in Physics.
- Therefore, the number of ways to choose the first prize winner in Physics = 30.
4. Second Prize in Physics:
- After awarding the first prize, 29 students remain eligible for the second prize in Physics.
- Therefore, the number of ways to choose the second prize winner in Physics = 29.
5. First Prize in Chemistry:
- There are 30 students eligible for the first prize in Chemistry.
- Therefore, the number of ways to choose the first prize winner in Chemistry = 30.
6. First Prize in Biology:
- There are also 30 students eligible for the first prize in Biology.
- Therefore, the number of ways to choose the first prize winner in Biology = 30.
7. Total Number of Ways (N):
- Now, we can calculate the total number of ways to award all the prizes:
N=(30 ways for Math 1st)×(29 ways for Math 2nd)×(30 ways for Physics 1st)×(29 ways for Physics 2nd)×(30 ways for Chemistry)×(30 ways for Biology)
N=30×29×30×29×30×30
8. Final Calculation:
- We can simplify this expression:
N=(304)×(292)
Conclusion:
The total number of ways to award the prizes is:
N=304×292
- There are 30 students eligible for the first prize in Mathematics.
- Therefore, the number of ways to choose the first prize winner in Mathematics = 30.
- After awarding the first prize, 29 students remain eligible for the second prize in Mathematics.
- Therefore, the number of ways to choose the second prize winner in Mathematics = 29.
- Again, there are 30 students eligible for the first prize in Physics.
- Therefore, the number of ways to choose the first prize winner in Physics = 30.
- After awarding the first prize, 29 students remain eligible for the second prize in Physics.
- Therefore, the number of ways to choose the second prize winner in Physics = 29.
- There are 30 students eligible for the first prize in Chemistry.
- Therefore, the number of ways to choose the first prize winner in Chemistry = 30.
- There are also 30 students eligible for the first prize in Biology.
- Therefore, the number of ways to choose the first prize winner in Biology = 30.
- Now, we can calculate the total number of ways to award all the prizes:
N=(30 ways for Math 1st)×(29 ways for Math 2nd)×(30 ways for Physics 1st)×(29 ways for Physics 2nd)×(30 ways for Chemistry)×(30 ways for Biology)
N=30×29×30×29×30×30
- We can simplify this expression:
N=(304)×(292)
The total number of ways to award the prizes is:
N=304×292
Similar Questions
In how many different ways the following 5 prizes be distributed among 10 students? First and second in Mathematics; first and second in physics and first in Hindi.
View SolutionIn how many ways first and second rank in mathematics, first and second rank in physics, first rank in chemistry, and first rank in English be given away to a class of 30 students.
View SolutionIn how many ways first and second rank in mathematics, first and second rank in physics, first rank in chemistry, and first rank in English be given away to a class of 30 students.
View SolutionA group of 2n students consisting of n boys and n girls are to be arranged in a row such that adjacent members are of opposite sex. The number of ways in which this can be done is
View SolutionFirst class lever and Second class lever
View SolutionA class has 175 students. The following table shows the number of students studying one or more of the following subjects in this case.
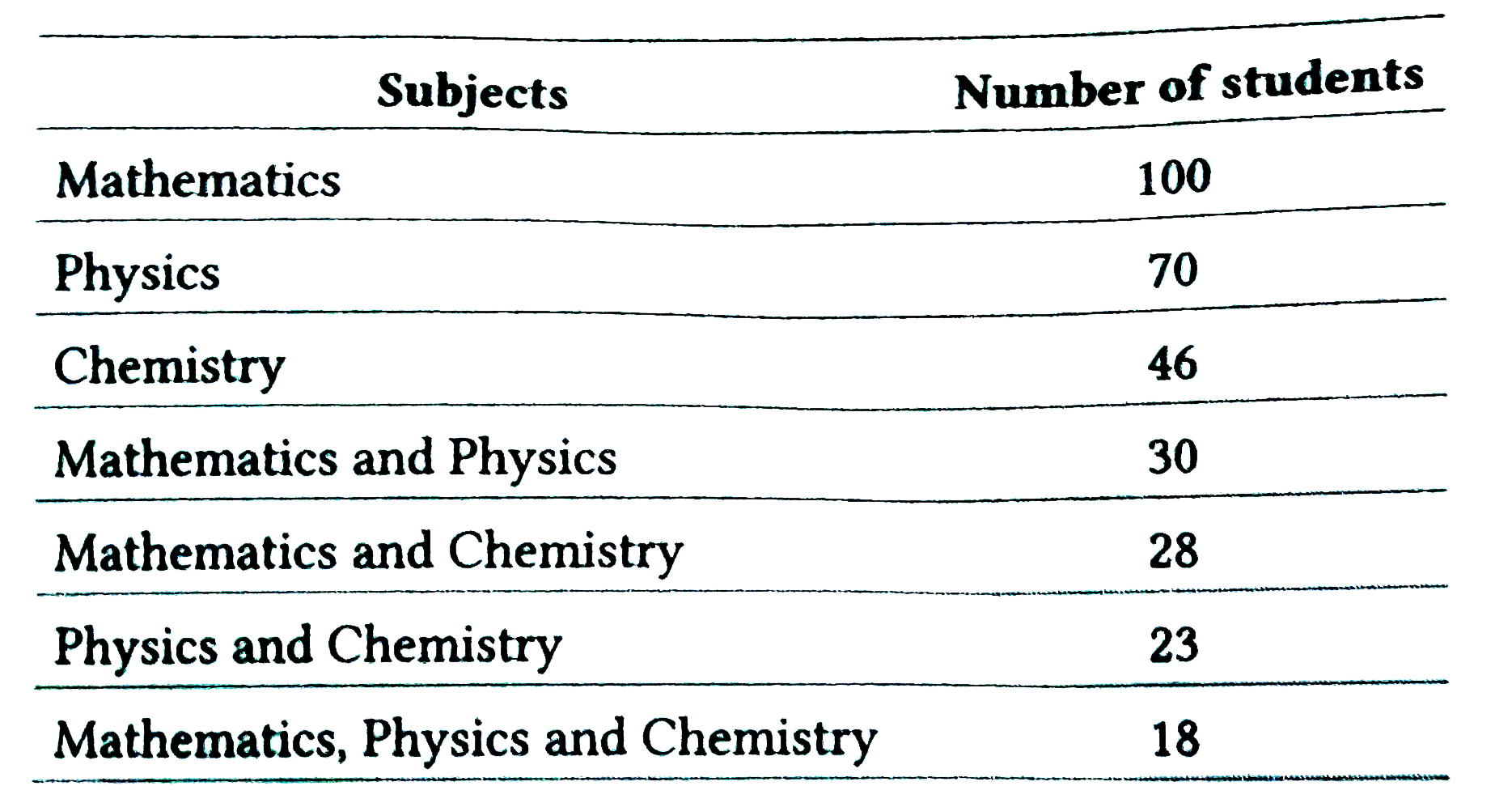
How many students are enrolled in Mathematics alone, Physics alone and Chemistry alone? Are there students who have not offered any one of these subjects?
View SolutionIn which of the following second ion is more stable than the first:
View SolutionA class has 175 students. The following data shows the number of students obtaining one or more subjects. Mathematics 100, Physics 70, Chemistry 40, Mathematics and Physics 30, Mathematics and Chemistry 28, Physics and Chemistry 23, Mathematics, Physics and Chemistry 18. How many students have offered Mathematics alone (a) 35 (c) 60 (b) 48 (d) 22
View SolutionIn which case first has lower melting point than second ?
View SolutionIn a class of 175 students the following data shows the number of students opting one or more subjects Mathematics 100, physics 70, chemistry 28, physics and Chemistry 23, mathematics and chemistry 28 , mathematics and physics 30 , mathematics physics and chemistry 18. how many students have offered mathematics alone ?
View Solution
