What is the fractional error in g calculated from ? Given that fractional error in and are and respectively.
The correct Answer is:
To find the fractional error in g calculated from the equation T=2π√lg, we will follow these steps:
Step 1: Understand the relationship
The relationship between the period T, length l, and acceleration due to gravity g is given by:
T=2π√lg
Step 2: Differentiate the equation
To find the fractional error in g, we need to differentiate the equation with respect to T and l. The formula for the propagation of errors in a function is:
ΔTT=12Δll+12Δgg
Here, ΔT, Δl, and Δg represent the absolute errors in T, l, and g, respectively.
Step 3: Rearranging for Δg
We can rearrange the equation to solve for the fractional error in g:
Δgg=2ΔTT−12Δll
Step 4: Substitute the given errors
We are given:
- The fractional error in T is ±3% or ΔTT=3100=0.03
- The fractional error in l is ±6% or Δll=6100=0.06
Substituting these values into the equation:
Δgg=2(0.03)−12(0.06)
Step 5: Calculate the fractional error in g
Calculating the right side:
Δgg=0.06−0.03=0.03
Step 6: Convert to percentage
To express this as a percentage, we multiply by 100:
Δgg×100=0.03×100=3%
Final Result
Thus, the fractional error in g is ±3%.
---
The relationship between the period T, length l, and acceleration due to gravity g is given by:
T=2π√lg
To find the fractional error in g, we need to differentiate the equation with respect to T and l. The formula for the propagation of errors in a function is:
ΔTT=12Δll+12Δgg
Here, ΔT, Δl, and Δg represent the absolute errors in T, l, and g, respectively.
We can rearrange the equation to solve for the fractional error in g:
Δgg=2ΔTT−12Δll
We are given:
- The fractional error in T is ±3% or ΔTT=3100=0.03
- The fractional error in l is ±6% or Δll=6100=0.06
Δgg=2(0.03)−12(0.06)
Calculating the right side:
Δgg=0.06−0.03=0.03
To express this as a percentage, we multiply by 100:
Δgg×100=0.03×100=3%
Thus, the fractional error in g is ±3%.
Similar Questions
Knowledge Check
An experiment measured quantity a, b, c and then x is calculated from x=ab2/c3. If the percentage error in a, b, c are ± 1%, ± 3% and ± 2% respectively, the percentage error in x can be,
AA) ± 13%BB) ± 7%CC) ± 4%DD) ± 1%
If T=2π√18, then relative errors in T and l are in the ratio
View SolutionWhat is the percentage error in the measurement of time period of a pendulum if maximum errors in the measurement of l ands g are 1% and 6% respectively?
View SolutionWhat is the percentage error in the measurement of time period of a pendulum if maximum errors in the measurement of l ands g are 2% and 4% respectively?
View SolutionWhat fraction is?
250 mL of 2L
View SolutionConsider the following arrangement and choose the correct options
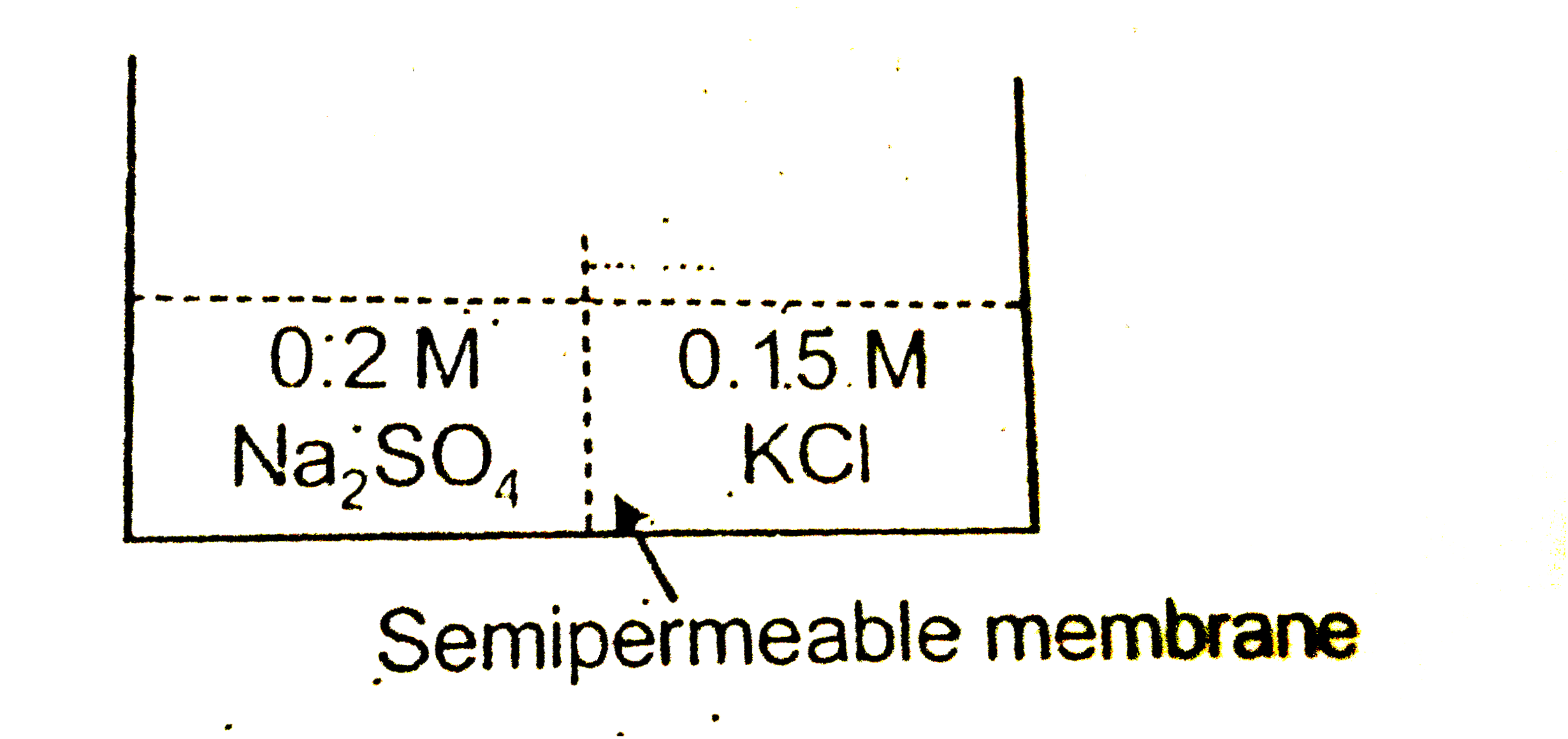
View SolutionAn experiment measure quantities x,y,z and then t is in calculate from the data as t=xy2z3 if perecentage error in x,y,z and are respectively 1%,3%,2% then percentage error in t is
View SolutionA physical quantity x is calculated from x=ab2/√c. Calculate the percentage error in measuring x when the percentage errors in measuring a , b , and c are 4 , 2 , and 3%, respectively .
View Solution
