Consider a radioactive material of half-life 1.0 minute. If one of the nuclei decays now, the next one will decay
The correct Answer is:at any time
To solve the question, we need to understand the concept of half-life and the nature of radioactive decay.
1. Understanding Half-Life: The half-life of a radioactive material is the time required for half of the radioactive nuclei in a sample to decay. In this case, the half-life is given as 1.0minute.
2. Radioactive Decay Process: Radioactive decay is a random process. This means that while we can predict the average behavior of a large number of nuclei, we cannot predict when a specific nucleus will decay.
3. Decay of One Nucleus: If one nucleus decays now, it does not imply that the next nucleus will decay after a fixed time. The decay of each nucleus is independent of the others.
4. Expected Time for Next Decay: Although we cannot predict the exact time for the next decay, we can calculate the average time between decays for a large number of nuclei. The average time between decays is related to the decay constant (λ) and can be derived from the half-life (T₁/₂) using the formula:
\(
T_{avg} = \frac{1}{\lambda}
\)
where \( \lambda = \frac{\ln(2)}{T_{1/2}} \).
5. Calculating the Decay Constant: Using the half-life:
\(
\lambda = \frac{\ln(2)}{1 \text{ minute}} \approx 0.693 \text{ min}^{-1}
\)
6. Calculating Average Time Between Decays:
\(
T_{avg} = \frac{1}{\lambda} = \frac{1}{0.693} \approx 1.44 \text{ minutes}
\)
This means that on average, we can expect the next nucleus to decay approximately 1.44 minutes after the first one.
7. Conclusion: Therefore, while we cannot determine the exact time for the next decay, we can say that on average, it will occur approximately 1.44 minutes after the first decay.
\(
T_{avg} = \frac{1}{\lambda}
\)
where \( \lambda = \frac{\ln(2)}{T_{1/2}} \).
\(
\lambda = \frac{\ln(2)}{1 \text{ minute}} \approx 0.693 \text{ min}^{-1}
\)
\(
T_{avg} = \frac{1}{\lambda} = \frac{1}{0.693} \approx 1.44 \text{ minutes}
\)
This means that on average, we can expect the next nucleus to decay approximately 1.44 minutes after the first one.
Similar Questions
For a radioactive material, half-life is 10 minutes. If initially there are 600 number of nuclei, the time taken (in minutes) for the disintegration of 450 nuclei is.
View SolutionFor a radioactive material, half-life is 10 minutes. If initially there are 600 number of nuclei, the time taken (in minutes) for the disintegration of 450 nuclei is.
View Solution
Knowledge Check
In a sample of radioactive material, what percentage of the initial number of active nuclei will decay during one mean life ?
A63%B69.3%C37%D50%In a sample of radioactive material , what fraction of the initial number of active nuclei will remain undisintegrated after half of the half life of the sample ?
A14B12√2C1√2D√2−1
Four thousand active nuclei of a radioactive material are present at t=0. After 60 minutes 500 active nuclei are left in the sample. The decay constant of the sample is
View SolutionA radioactive sample is decayed 20% in one day. After next two days, the undecayed percentage of nuclei will be
View SolutionA certain radioactive element has half-life of 4 days. The fraction of material that decays in 2days is
View SolutionComplete the following table for a radioactive element whose half life is 5 minutes. Assume that you have 32 8 of this element at start, i.e., at t=0.
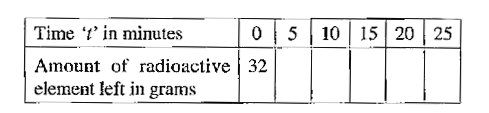
Now, using this data, plot the "decay curve"
View SolutionThe half-life period of a radioactive substance is 5min. The amount of substance decayed in 20min will be
View SolutionIn a sample of radioactive material, what fraction of initial number of active nuclei will remain undistintegrated after half of a half0life of the sample?
View Solution
