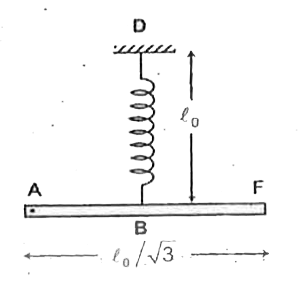
In the figure shown one end of a light spring of natural length l0=√58m(≈0.8m) is fixed at point D and other end is attached to the centre B of a uniform rod AF of length l0/√3 and mass 10kg. The rod is free to rotate in a vertical plane about a fixed horizontal axis passing through the end A of the rod. The rod is held at rest in horizontal position and the spring is in relaxed state. It is found that, when the rod is released to move it makes an angle of 60∘ with the horizontal when it comes to rest for the first time. Find the
(a) the maximum elogation in the spring . (Approximately)
(b) the spring constant. (Approximately)
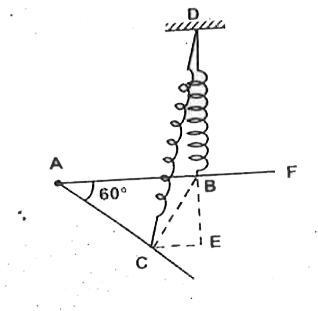
In triangle ABC
AB=AC
From geometry in ΔABC
∴BC=l02√3
So, ∠ABC=∠ACB=60∘
Also in triangle BCD,∠DBC=150∘
Applying cosine law
DC2=DB2+BC2−2DB.BCcos150∘
=58+112×58+2√58.12√3×√58×√32
DC≈1m
∴ Expansion in the spring is x=1−0.8≈0.2m
Vertical displacement of centre of mass of the rod
BE=ACsin60∘=l02√3(√32)=0.2m
Using conservation of mechanical energy
Mg(BE)=12kx2
10×10×0.2=12k(0.2)2
⇒K=103N/m
⇒k≈103N/m
Similar Questions
A uniform rod of mass m and length L is free to rotate in the vertical plane about a horizontal axis passing through its end. The rod initially in horizontal position is released. The initial angular acceleration of the rod is:

View Solution
A uniform rod of length L is free to rotate in a vertical plane about a fixed horizontal axis through B. The rod begins rotating from rest. The angular velocity ω at angle θ is given as
View SolutionA uniform rod of length l and mass m is free to rotate in a vertical plane about A as shown in Fig. The rod initially in horizontal position is released. The initial angular acceleration of the rod is

View Solution
A uniform rod of length L and mass m is free to rotate about a frictionless pivot at one end as shown in figure. The rod is held at rest in the horizontal position and a coin of mass m is placed at the free end. Now the rod is released The reaction on the coin immediately after the rod starts falling is
View SolutionA L shaped rod of mass M is free to rotate in a vertical plane about axis A A as shown in figure. Maximum angular acceleration of rod is

View SolutionA uniform rod AB of length l and mass m is free to rotate about an Axis passing through A and perpendicular to length of the rod. The rod is released from rest as shown in figure. Given that the moment of inertia of the rod about A is ml23 The initial angular acceleration of the rod will be
View SolutionFind the moment of inertia A of a spherical ball of mass m and radius r attached at the end of a straight rod of mass M and length l, if this system is free to rotate about an axis passing through the end of the rod (end of the rod opposite to sphere).

View SolutionA thin uniform copper rod of length l and mass m rotates uniformly with an angular velocity ω in a horizontal plane about a vertical axis passing through one of its ends. Determine the tension in the rod as a function of the distance r from the rotation axis. Find the elongation of the rod.
View SolutionA charge of 1 coulumb is placed is one end of a non-conducting rod of length 0.6m.The rod is rotated in a vertical plane about a horizontal axis passing through the other end of the rod with an angular velocity 104πrad/sec.Find the magnetic field at a point on the axis of rotation of a distance of 0.8m from the centre of the path is Nπ×10−4T,then find value of N.
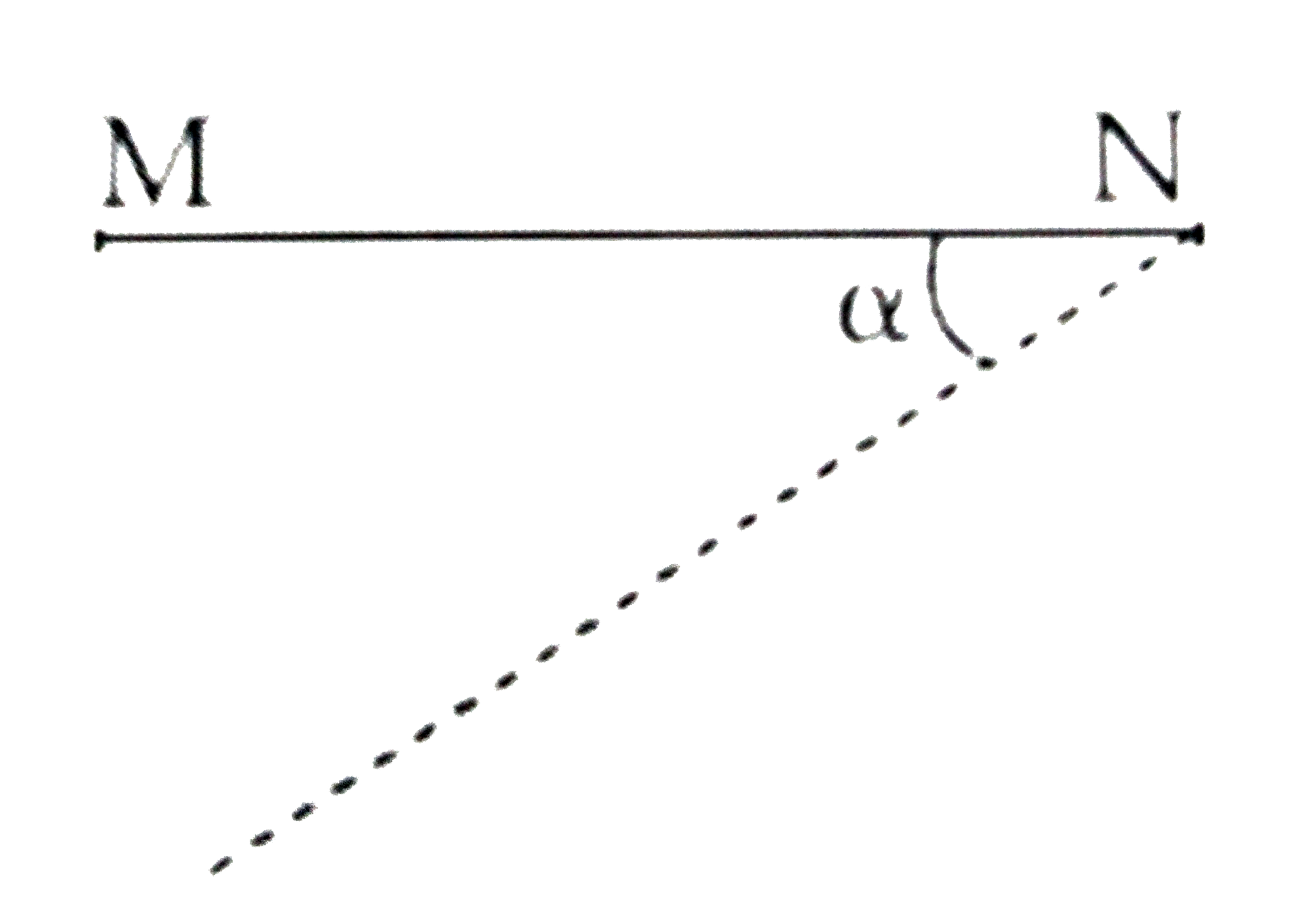
View SolutionA thin rod MN free to rotation in the vertical plane about the fixed end N is held horizontal when the end M is released the speed of this end when the rod makes an angle α with the horizontal will be proportional to : (see figure)
View Solution
