The minimum number of communication satellites (Geostationary) required to link the communication network of entire earth is
1
2
3
4
To determine the minimum number of geostationary communication satellites required to cover the entire Earth, we can follow these steps:
Step 1: Understand the Geostationary Orbit
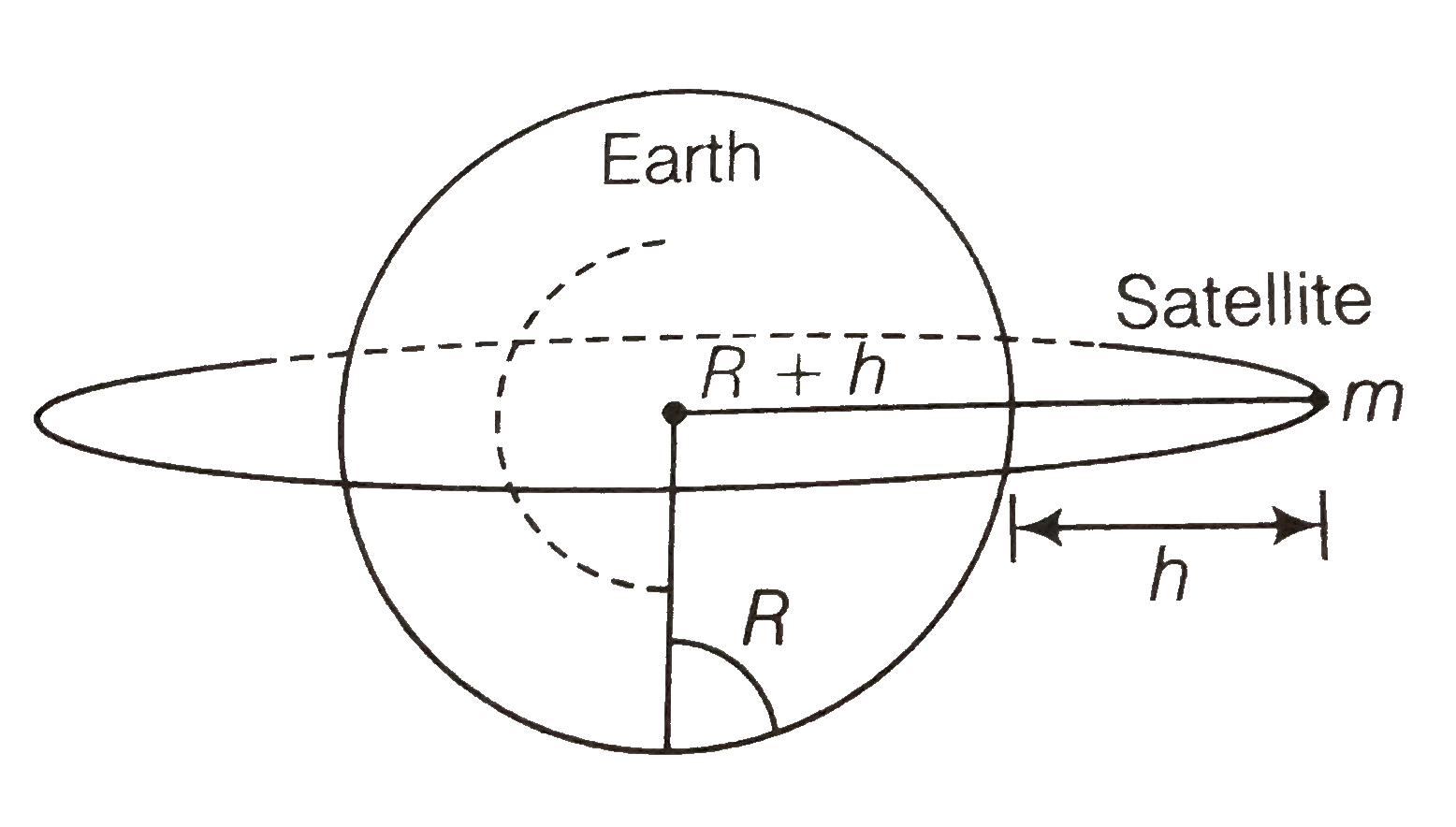
A geostationary satellite orbits the Earth at a specific altitude where it appears to be stationary relative to a point on the Earth's surface. The radius of orbit (R) from the center of the Earth is approximately 42,400 km, which includes the Earth's radius (about 6,400 km) and the height (h) above the Earth's surface (approximately 36,000 km).
Step 2: Calculate the Area Covered by One Satellite
The area covered by one geostationary satellite can be calculated using the formula for the area of a circle:
A=π(R+h)2
Substituting the values:
- R + h = 42,400 km
- Therefore, A=π(42,400)2
Calculating this:
A≈π×(42,400)2≈π×1.79856×109≈5.64×109 km2
Step 3: Calculate the Total Area of the Earth
The total surface area of the Earth (AE) can be calculated using the formula for the surface area of a sphere:
AE=4πR2E
Where RE is the radius of the Earth (approximately 6,400 km):
AE≈4π(6,400)2≈4π×40,960,000≈5.1×108 km2
Step 4: Determine the Coverage Percentage
To find out how many satellites are needed, we need to find out how much area one satellite covers in relation to the total area of the Earth:
Coverage Percentage=AAE
Substituting the values:
Coverage Percentage=5.64×1095.1×108≈11.06
This means that one satellite can cover approximately 11 times the area of the Earth.
Step 5: Calculate the Minimum Number of Satellites
Since one satellite can cover a significant portion of the Earth, we can determine the minimum number of satellites needed by taking the ceiling of the ratio of the total area of the Earth to the area covered by one satellite:
N=AEA
Since one satellite covers 37% of the Earth, we can conclude that:
N≈10.37≈3
Thus, the minimum number of geostationary satellites required to cover the entire Earth is 3.
Final Answer
The minimum number of communication satellites (Geostationary) required to link the communication network of the entire Earth is 3.
---
A geostationary satellite orbits the Earth at a specific altitude where it appears to be stationary relative to a point on the Earth's surface. The radius of orbit (R) from the center of the Earth is approximately 42,400 km, which includes the Earth's radius (about 6,400 km) and the height (h) above the Earth's surface (approximately 36,000 km).
The area covered by one geostationary satellite can be calculated using the formula for the area of a circle:
A=π(R+h)2
Substituting the values:
- R + h = 42,400 km
- Therefore, A=π(42,400)2
A≈π×(42,400)2≈π×1.79856×109≈5.64×109 km2
The total surface area of the Earth (AE) can be calculated using the formula for the surface area of a sphere:
AE=4πR2E
Where RE is the radius of the Earth (approximately 6,400 km):
AE≈4π(6,400)2≈4π×40,960,000≈5.1×108 km2
To find out how many satellites are needed, we need to find out how much area one satellite covers in relation to the total area of the Earth:
Coverage Percentage=AAE
Substituting the values:
Coverage Percentage=5.64×1095.1×108≈11.06
Since one satellite can cover a significant portion of the Earth, we can determine the minimum number of satellites needed by taking the ceiling of the ratio of the total area of the Earth to the area covered by one satellite:
N=AEA
Since one satellite covers 37% of the Earth, we can conclude that:
N≈10.37≈3
The minimum number of communication satellites (Geostationary) required to link the communication network of the entire Earth is 3.
Similar Questions
Knowledge Check
A satellite is to be placed in equatorial geostationary orbit around the earth for communication.
(a) Calculate height of such a satellite
(b) Find out the minimum number of satellites that are needed to cover entire earth, so that atleast one satellite is visible from any point on the equator.
[M=6×1024kg,R=6400km,T=24h,G=6.67×10−11 SI unit ]A3.57×105mB3.57×106mC3.57×107mD3.57×108mFor base station to mobile communication, the required frequency band is
A540-1600 KHzB200-325MHzC5.9-6.42GHzD840-935 MHzFor base station to mobile communication, the required frequency band is
A540-1600 KHzB200-325MHzC5.9-6.42GHzD840-935 MHz
In the satellite communication, the up linking frequency range is
View SolutionA satellite is to be placed in equatorial geostationary orbit around earth for communication.
(a) Calculate height of such a satellite.
(b) Find out the minimum number of satellites that are needed to cover entire earth so that at least one satellites is visible from any point on the equator.
[M=6×1024kg,R=6400km,T=24h,G=6.67×10−11SIunits]
View SolutionPerhaps, the most commonly used mode of communication involves use of free space as a communication channel between transmitter and receiver. The various possible modes of using of free space have been described below
Ground wave communication : This mode is used for low frequency as at high frequency, losses become large. This is useful for distance of the order of 1 km.
Space wave communication: The signals are transmitted directly from transmitter to receiver. There is no limit to upper frequency, but its range is limited to few hundred kilometers. The range depends on the height of transmitting antenna
Sky wave communication : The signals are sent towards sky They are reflected back towards earth by ionosphere. Its range can be above 500 km. But, very high frequencies (above 10 MHz) are not reflected back. Satellite communication: The signals that cannot be reflected back by ionosphere are reflected back by satellite. Moon is a passive satellite of earth.
The frequency range allotted to FM radio broadcast is 88 MHz to 108 MHz. Which of the following communication channels can be used for its transmission?
A. Sky wave communication only
B. Space wave communication only
C. Satellite communication only
D. Either space wave communication or satellite communication
View SolutionOrbital radius of a satellite S of earth is four times that s communication satellite C. Period of revolution of S is :-
View SolutionThe ratio of earth's surface area as covered by two communication satellite moving synchronously in equatorial plane with orbital speed of 1√2 times and √35 times that of required orbital speed near earths surface is
View Solution
