STATEMENT-1 : A particle is projected with a velocity 0.5√gRe from the surface of the earth at an angle of 30∘ with the vertical (at that point ) its velocity at the highest point will be 0.25√gRe
because
STATEMENT-2 : Angular momentum of the particle earth system remains constant.
Statement-1 is True, Statement-2 is True, Statement-2 is a correct explanation for Statement-1
Statement-1 is True, Statement-2 is True, Statement-2 is NOT a correct explanation for Statement-1
Statement-1 is True , Statement-2 is False
Statement-1 is False , Statement-2 is True
The correct Answer is:D
To solve the problem, we need to analyze the two statements provided:
Statement 1: A particle is projected with a velocity 0.5√gRe from the surface of the Earth at an angle of 30∘ with the vertical. Its velocity at the highest point will be 0.25√gRe.
Statement 2: The angular momentum of the particle-Earth system remains constant.
1. Identify the Initial Velocity Components:
The initial velocity u of the particle is given as:
u=0.5√gRe
The angle of projection with the vertical is 30∘. Therefore, we can find the horizontal and vertical components of the initial velocity:
- Horizontal component (ux):
ux=usin(30∘)=0.5√gRe⋅sin(30∘)=0.5√gRe⋅12=0.25√gRe
- Vertical component (uy):
uy=ucos(30∘)=0.5√gRe⋅cos(30∘)=0.5√gRe⋅√32=√34√gRe
2. Determine the Maximum Height:
At the highest point of the projectile's motion, the vertical component of the velocity becomes zero. The time taken to reach the highest point can be calculated using the vertical motion equations:
vy=uy−gt
Setting vy=0 at the highest point gives:
0=√34√gRe−gt⟹t=√34g√gRe=√3Re4
3. Calculate the Horizontal Distance:
The horizontal component of the velocity remains constant throughout the motion. Therefore, at the highest point, the horizontal velocity is:
vx=ux=0.25√gRe
4. Final Velocity at the Highest Point:
Since the vertical component of the velocity is zero at the highest point, the total velocity at that point is simply the horizontal component:
v=vx=0.25√gRe
5. Conclusion for Statement 1:
Thus, the velocity at the highest point is indeed 0.25√gRe, confirming that Statement 1 is true.
6. Conclusion for Statement 2:
The angular momentum of the particle-Earth system remains constant due to the conservation of angular momentum in the absence of external torques. However, it does not provide a direct explanation for the velocity at the highest point. Therefore, while Statement 2 is true, it is not a correct explanation for Statement 1.
Final Answer:
- Statement 1 is true.
- Statement 2 is true but does not explain Statement 1.
The initial velocity u of the particle is given as:
u=0.5√gRe
The angle of projection with the vertical is 30∘. Therefore, we can find the horizontal and vertical components of the initial velocity:
- Horizontal component (ux):
ux=usin(30∘)=0.5√gRe⋅sin(30∘)=0.5√gRe⋅12=0.25√gRe
- Vertical component (uy):
uy=ucos(30∘)=0.5√gRe⋅cos(30∘)=0.5√gRe⋅√32=√34√gRe
At the highest point of the projectile's motion, the vertical component of the velocity becomes zero. The time taken to reach the highest point can be calculated using the vertical motion equations:
vy=uy−gt
Setting vy=0 at the highest point gives:
0=√34√gRe−gt⟹t=√34g√gRe=√3Re4
The horizontal component of the velocity remains constant throughout the motion. Therefore, at the highest point, the horizontal velocity is:
vx=ux=0.25√gRe
Since the vertical component of the velocity is zero at the highest point, the total velocity at that point is simply the horizontal component:
v=vx=0.25√gRe
Thus, the velocity at the highest point is indeed 0.25√gRe, confirming that Statement 1 is true.
The angular momentum of the particle-Earth system remains constant due to the conservation of angular momentum in the absence of external torques. However, it does not provide a direct explanation for the velocity at the highest point. Therefore, while Statement 2 is true, it is not a correct explanation for Statement 1.
- Statement 1 is true.
- Statement 2 is true but does not explain Statement 1.
Similar Questions
A particle is thrown with escape velocity ve from the surface of earth. Calculate its velocity at height 3 R :-
View SolutionA particle of mass m is projected with a velocity v at an angle of θ with horizontal. The angular momentum of the particle at the highest point of its trajectory is equal to :
View SolutionA particle is projected vertically upward with with velocity √23GMR from the surface of Earth. The height attained by it is (G, M, R have usual meanings)
View SolutionA particle is projected with a velocity 10 m//s at an angle 37∘ to the horizontal. Find the location at which the particle is at a height 1 m from point of projection.
View SolutionSTATEMENT-1: A body is projected from the ground with kinetic energy K at an angle of 60° with the horizontal. If air resistance is neglected, its K.E. When it is at the highest point of its trajectory will be K/4
because
STATEMENT-2: At the highest point of the trajectory, the direction of velocity and acceleration of the body are perpendicular to each other
View SolutionThe particle of mass 1 kg is projected with velocity 20√2m/s at 45∘ with ground . When , the particle is at highest point (g=10m/s2),
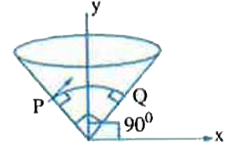
View SolutionA particle is projected from point P with velocity 5√2ms−1 prependicular to the surface of a hollow right angle cone whose axis is vertical . It collides at Q normally. The time of the flight of the particle is .
View SolutionA particle of mass m is projected with velocity v at an angle θ with the horizontal. Find its angular momentum about the point of projection when it is at the highest point of its trajectory.
View SolutionA particle is projected vertically upwards the surface of the earth (radius Re) with a speed equal to one fourth of escape velocity what is the maximum height attained by it from the surface of the earth?
View SolutionA particle of mass m is projected form ground with velocity u making angle θ with the vertical. The de Broglie wavelength of the particle at the highest point is
View Solution
