A disc is rotating about vertical axis OA, which is tangent to the circumference and perpendicular to the plane of disc. Its rotational energy is given as E. Find the time period of rotation.

Moment of inertia about OA=12mR2+mR2=32mR2
E=12IOAω2=1232mR2ω2
43EmR2=ω2
⇒ ω=√43EmR2
Time period =2πω=2π√3mR24E=π√3mR2E
Similar Questions
A quarter disc of radius R and mass m is rotating about the axis OO' (perpendicular to the plane of the disc) as shown. Rotational kinetic energy of the quarter disc is
 View Solution
View SolutionA disc is free to rotate about an axis passing through its centre and perpendicular to its plane. The moment of inertia of the disc about its rotation axis is I. A light ribbon is tightly wrapped over it in multiple layers. The end of the ribbon is pulled out at a constant speed of u. Let the radius of the ribboned disc be R at any time and thickness of the ribbon be d(<<R). Find the force (F) required to pull the ribbon as a function of radius R.
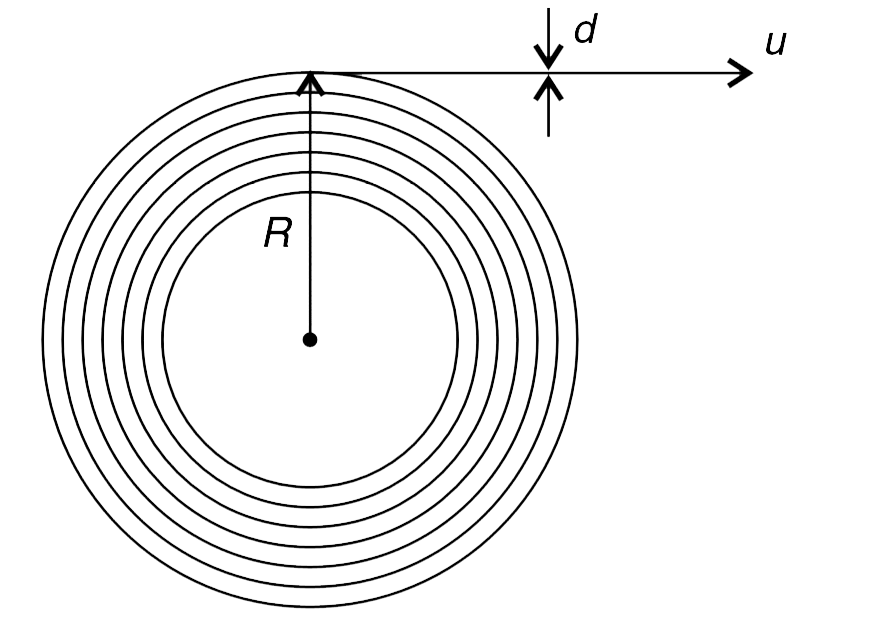 View Solution
View SolutionThe moment of inertia of a copper disc, rotating about an axis passing through its centre and perpendicular to its plane
View SolutionA disc of radius R is pivoted at its rim. The period for small oscillations about an axis perpendicular to the plane of disc is
View SolutionSuppose a disc is rotating counter clockwise in the plane of the paper then
View SolutionTwo discs of moment of inertia I1 and I2 and angular speeds ω1 and ω2 are rotating along the collinear axes passing through their center of mass and perpendicular to their plane. If the two are made to rotate combindly along the same axis the rotational K.E. of system will be
View SolutionTwo discs of same moment of inertia rotating about their regular axis passing through centre and perpendicular to the plane of disc with angular velocities ω1 and ω2. They are brought into contact face to face coinciding the axis of rotation. The expression for loss of energy during this process is
View SolutionOn a disc of radius R a concentric circle of radius R/2 is drawn. The disc is free to rotate about a frictionless fixed axis through its center and perpendicular to plane of the disc. All three forces ( in plane of the disc ) shown in figure are exerted tangent to their respective circular periphery. The magnitude of the net torque ( about centre of disc ) acting on the disc is :
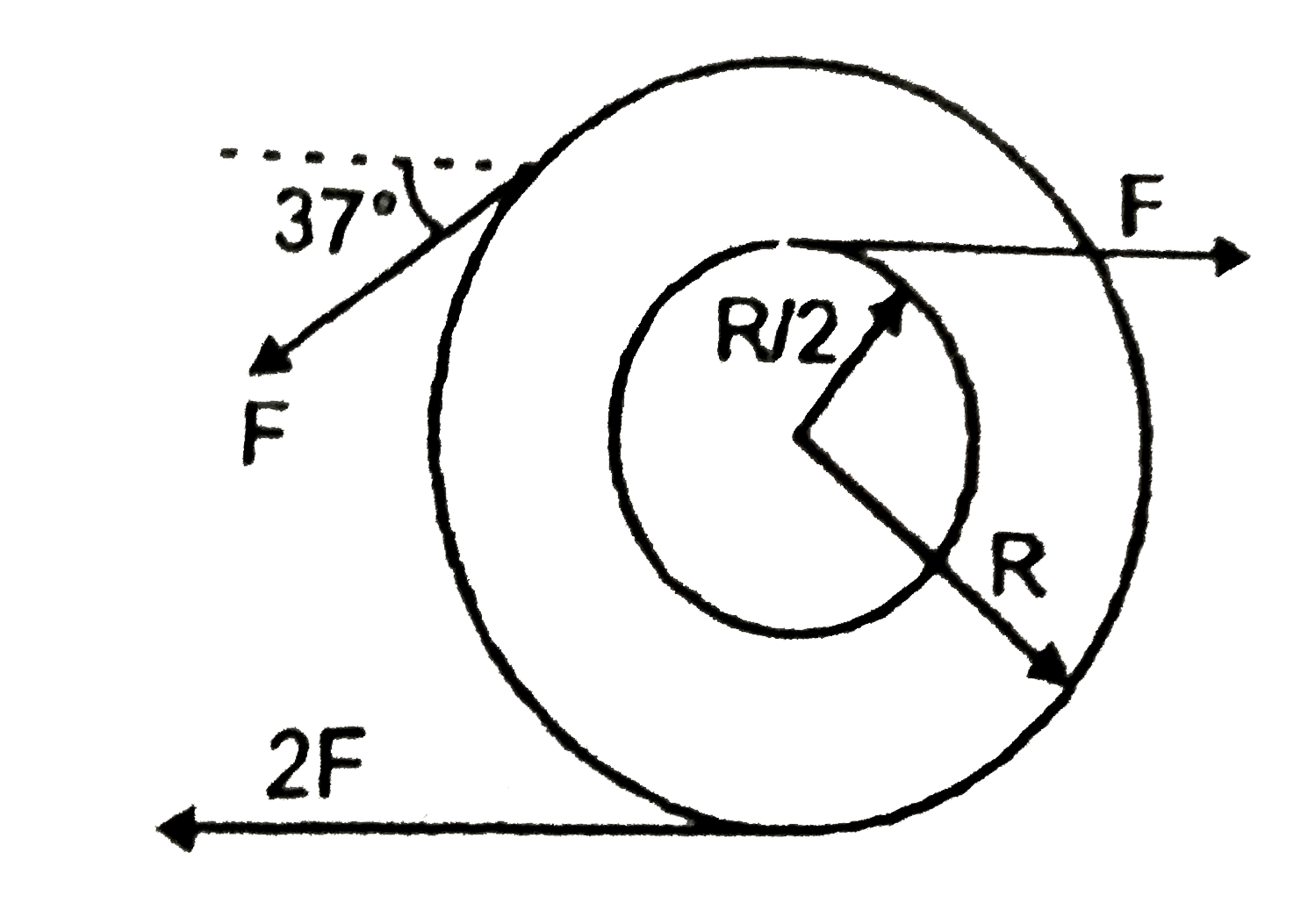 View Solution
View SolutionOn a disc of radius R a concentric circle of radius R/2 is drawn. The disc is free to rotate about a frictionless fixed axis through its center and perpendicular to plane of the disc. All three forces ( in plane of the disc ) shown in figure are exerted tangent to their respective circular periphery. The magnitude of the net torque ( about centre of disc ) acting on the disc is :
 View Solution
View SolutionA ring of mass m and radius r rotates about an axis passing through its centre and perpendicular to its plane with angular velocity ω. Its kinetic energy is
View Solution

