Sixty five percent of children in a sport club play football, 70 percent play volley ball and 75 per-cent play basket ball. What is the smallest percentage of children playing all the three games?
To find the smallest percentage of children playing all three games (football, volleyball, and basketball), we can use the principle of inclusion-exclusion. Here’s a step-by-step solution:
Step 1: Define the total number of children
Let’s assume the total number of children in the sport club is 100.
Step 2: Determine the number of children playing each sport
- Number of children playing football = 65% of 100 = 65 children
- Number of children playing volleyball = 70% of 100 = 70 children
- Number of children playing basketball = 75% of 100 = 75 children
Step 3: Calculate the number of children not playing each sport
- Number of children not playing football = 100 - 65 = 35 children
- Number of children not playing volleyball = 100 - 70 = 30 children
- Number of children not playing basketball = 100 - 75 = 25 children
Step 4: Calculate the total number of children not playing at least one game
To find the maximum number of children who do not play at least one game, we sum the number of children not playing each sport:
- Total not playing any game = 35 (not playing football) + 30 (not playing volleyball) + 25 (not playing basketball) = 90 children
Step 5: Determine the minimum number of children playing all three games
Since there are 100 children in total, the minimum number of children who play all three games can be calculated as:
- Minimum number playing all three games = Total children - Total not playing any game
- Minimum number playing all three games = 100 - 90 = 10 children
Step 6: Calculate the percentage of children playing all three games
- Percentage of children playing all three games = (Number of children playing all three games / Total number of children) × 100
- Percentage of children playing all three games = (10 / 100) × 100 = 10%
Final Answer
The smallest percentage of children playing all three games is 10%.
---
Let’s assume the total number of children in the sport club is 100.
- Number of children playing football = 65% of 100 = 65 children
- Number of children playing volleyball = 70% of 100 = 70 children
- Number of children playing basketball = 75% of 100 = 75 children
- Number of children not playing football = 100 - 65 = 35 children
- Number of children not playing volleyball = 100 - 70 = 30 children
- Number of children not playing basketball = 100 - 75 = 25 children
To find the maximum number of children who do not play at least one game, we sum the number of children not playing each sport:
- Total not playing any game = 35 (not playing football) + 30 (not playing volleyball) + 25 (not playing basketball) = 90 children
Since there are 100 children in total, the minimum number of children who play all three games can be calculated as:
- Minimum number playing all three games = Total children - Total not playing any game
- Minimum number playing all three games = 100 - 90 = 10 children
- Percentage of children playing all three games = (Number of children playing all three games / Total number of children) × 100
- Percentage of children playing all three games = (10 / 100) × 100 = 10%
The smallest percentage of children playing all three games is 10%.
Similar Questions
In a class, 45% students play football and the rest play cricket. What per cent of the students play cricket?
View SolutionIn a group of children, 35 play football out of which 20 play football only, 22 play hockey, 25 play cricket out of which 11 play cricket only. Out of these 7 play cricket and football but not hockey, 3 play football and hockey but not cricket and 12 play football and cricket both.
How many play all the three games ? How many play cricket and hockey but not football, how many play hockey only? What is the total number of children in the group?
View Solution
Knowledge Check
A recent survey reported that 60 percent of the students at a high school are girls and 65 percent of girls at this school play a sport. If a student at this high school were selected at random, what is the probability that the student is a girl who plays a sport?
A0.1B0.21C0.32D0.42Mare understanding needs to be cultivated of the social process of organizations and, in particular, the role of one's own behaviour in the production and reproduction of organisational life. For it is true that management is too potent in its effects upon the lies of employees, consumers and citizens to be guided by an instrumental form of rationality. MBA programs strongly advise students on the importance of safeguarding their careers. Almost everything and everyone is seen as a stepping stone or an obstacle in realizing this end. In education and educational matters it helps to explicitly discuss the tendency people have of avoiding emotions or discussions on power problems and underlying dynamic processes. The thing that makes experimental learning so very valuable is not possible until there is no escape route left. Students and other participants in the programme will initially be opposed to expressing their emotions and will also feel ill at ease, but getting over this helps and facilitates their learning. Things do not have to be what they seem at first glance. Let me illustrate this. Two children are playing and they both fall into the mud. One of the children didn't get a dirty face and when he sees the soiled face of his friend, it is understandable that he should run inside to wash. The other, the one who actually is dirty and could do with a good wash, stays sitting there quietly. He didn't get a fright at all, after all, his friend looked the same as always. However, it is far less understandable if it is managers who become the unwitting prisoner of supposed Minstrumental" perception. The technical view of management practice can be criticized from both inside and outside). In the latter case, it is the attempt to dominate others which is criticized. (Looking at the instrumental apparatus from the inside, there is absolutely no proof that control techniques actually lead to the desired control. Drucker has already recognized that "control weakens control." In other words, even within one's own frame of reference, purely technical viewson management practice are not particularly moral or effective. An education plan which emphasizes that a manager will always be a tangible, approachable person have to leam to deal with the insecurity, doubts and mysteries. The lecture hall will often be a safer place than one's place of work of thinking, experimenting, testing, and questioning. It is good place to practice and to learn to be alert in everyday work situations. The role of management education is therefore inclusive, and is also concerned with accepting daily dilemmas, both moral and instrumental, and no longer believing that a technical approach can be neutral, even though it seems to be taught that way. The past 20 years, which have been characterized in the West by a modern neo-capitalistic political climate, have increasingly validated the usefulness of management education. On the one hand, education is considered as socially competitive. On the other hand, within the framework of an individualistic consumer culture, education is considered personally valuable in so far as it enhances the economic position and career of the individual. Business studies and management education score well on both criteria. However, this will remain true only if people continue to feel there is a positive relationship between management education, results in management practice, and managers' individual achievements.
Facilitates:AencourageBadvanceCpromotesDall of themMare understanding needs to be cultivated of the social process of organizations and, in particular, the role of one's own behaviour in the production and reproduction of organisational life. For it is true that management is too potent in its effects upon the lies of employees, consumers and citizens to be guided by an instrumental form of rationality. MBA programs strongly advise students on the importance of safeguarding their careers. Almost everything and everyone is seen as a stepping stone or an obstacle in realizing this end. In education and educational matters it helps to explicitly discuss the tendency people have of avoiding emotions or discussions on power problems and underlying dynamic processes. The thing that makes experimental learning so very valuable is not possible until there is no escape route left. Students and other participants in the programme will initially be opposed to expressing their emotions and will also feel ill at ease, but getting over this helps and facilitates their learning. Things do not have to be what they seem at first glance. Let me illustrate this. Two children are playing and they both fall into the mud. One of the children didn't get a dirty face and when he sees the soiled face of his friend, it is understandable that he should run inside to wash. The other, the one who actually is dirty and could do with a good wash, stays sitting there quietly. He didn't get a fright at all, after all, his friend looked the same as always. However, it is far less understandable if it is managers who become the unwitting prisoner of supposed Minstrumental" perception. The technical view of management practice can be criticized from both inside and outside). In the latter case, it is the attempt to dominate others which is criticized. (Looking at the instrumental apparatus from the inside, there is absolutely no proof that control techniques actually lead to the desired control. Drucker has already recognized that "control weakens control." In other words, even within one's own frame of reference, purely technical viewson management practice are not particularly moral or effective. An education plan which emphasizes that a manager will always be a tangible, approachable person have to leam to deal with the insecurity, doubts and mysteries. The lecture hall will often be a safer place than one's place of work of thinking, experimenting, testing, and questioning. It is good place to practice and to learn to be alert in everyday work situations. The role of management education is therefore inclusive, and is also concerned with accepting daily dilemmas, both moral and instrumental, and no longer believing that a technical approach can be neutral, even though it seems to be taught that way. The past 20 years, which have been characterized in the West by a modern neo-capitalistic political climate, have increasingly validated the usefulness of management education. On the one hand, education is considered as socially competitive. On the other hand, within the framework of an individualistic consumer culture, education is considered personally valuable in so far as it enhances the economic position and career of the individual. Business studies and management education score well on both criteria. However, this will remain true only if people continue to feel there is a positive relationship between management education, results in management practice, and managers' individual achievements.
EconomicAFreedom cannot exist apart from some amount of economic liberty.BThis graph shows political, economical and moral culture.CWe face severe disruptions, when we transit from one set of economic realities to another.DThe economic class in railways is divided into five styles.
In a group of children, one-fifth play football, one-fourth play basketball, two-fifths play cricket, and the remaining 30 play other games. Find the total number of children in the group.
View SolutionIn the International Oxford School, all students play at least one of the two games rugby and baseball . 40% of all students play both rugby and baseball. If 20% of the students who play baseball do not play rugby , then what is the percentage of all students who plya baseball ?
View SolutionA survey of 40 children showed that 25% liked playing football. Howmany children liked playing football
View SolutionOf the members of three athletic teams in a certain school, 21 are in the basketball team, 26 in hockey team and 29 in the football team. 14 play hockey and basket ball, 15 play hockey and football, 12 play football and basketball and 8 play all the three games bow many members are there in all?
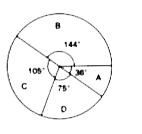
View SolutionThe adjoining diagram shows a pie-chart with central angles for number of players playing four games A, B, C and D. If each player takes part in only one game and there are 480 players in all, find :
(i) how many players play the game D?
(ii) how many players do not play game B?
(iii) what is the ratio of players playing game A to the players playing game C ?
(iv) what percentage of players are playing game B?
View Solution
