In one dimentional collision between two idential particles 'A' and 'B'. 'B' is stationary and 'A' has momentum 54 kg-m/ sec before impact. During impact , 'B' gives an impulse 3kg-m/sec to 'A' . The coefficient of restitution between A and B is
2/5
1/5
1/2
8/9
The correct Answer is:B
To find the coefficient of restitution between two identical particles A and B during a one-dimensional collision, we can follow these steps:
Step 1: Understand the initial conditions
- Particle A has an initial momentum PA=54kg m/s.
- Particle B is stationary, so its initial momentum PB=0kg m/s.
Step 2: Determine the impulse given to A
- During the collision, particle B gives an impulse of J=3kg m/s to particle A.
Step 3: Calculate the final momentum of particle A
- The change in momentum for particle A due to the impulse is given by:
P′A=PA−J
Substituting the values:
P′A=54−3=51kg m/s
Step 4: Calculate the final momentum of particle B
- Since the impulse given to A is equal and opposite to the impulse received by B, the momentum of particle B after the collision is:
P′B=J=3kg m/s
Step 5: Determine the velocities of A and B after the collision
- To find the velocities, we can use the relationship between momentum and velocity:
v′A=P′Amandv′B=P′Bm
Since both particles are identical, we can denote their mass as m. Thus:
v′A=51mandv′B=3m
Step 6: Calculate the coefficient of restitution
- The coefficient of restitution e is defined as:
e=relative velocity of separationrelative velocity of approach
- The relative velocity of separation is:
vseparation=v′A−v′B=51m−3m=48m
- The relative velocity of approach (before the collision) is:
vapproach=vA−vB=54m−0=54m
- Therefore, the coefficient of restitution can be calculated as:
e=48m54m=4854=89
Final Answer
The coefficient of restitution between particles A and B is 89.
---
- Particle A has an initial momentum PA=54kg m/s.
- Particle B is stationary, so its initial momentum PB=0kg m/s.
- During the collision, particle B gives an impulse of J=3kg m/s to particle A.
- The change in momentum for particle A due to the impulse is given by:
P′A=PA−J
Substituting the values:
P′A=54−3=51kg m/s
- Since the impulse given to A is equal and opposite to the impulse received by B, the momentum of particle B after the collision is:
P′B=J=3kg m/s
- To find the velocities, we can use the relationship between momentum and velocity:
v′A=P′Amandv′B=P′Bm
Since both particles are identical, we can denote their mass as m. Thus:
v′A=51mandv′B=3m
- The coefficient of restitution e is defined as:
e=relative velocity of separationrelative velocity of approach
- The relative velocity of separation is:
vseparation=v′A−v′B=51m−3m=48m
- The relative velocity of approach (before the collision) is:
vapproach=vA−vB=54m−0=54m
- Therefore, the coefficient of restitution can be calculated as:
e=48m54m=4854=89
The coefficient of restitution between particles A and B is 89.
Similar Questions
In a one dimensional collision between two identical particles A and B, B is stationary and A has momentum p before impact. During impact, B gives impulse J to A.
View SolutionIn a one-dimensional collision between two identical particles. A and B, B is stationary and A has momentum p before impact. During impact, B gives an impulse J to A. Find the coefficient of restitution between A and B?
View SolutionIn a one-dimensional collision between two identical particles. A and B, B is stationary and A has momentum p before impact. During impact, B gives an impulse J to A. Find the coefficient of restitution between A and B?
View SolutionIn a one-dimensional collision between two identical particles. A and B, B is stationary and A has momentum p before impact. During impact, B gives an impulse J to A. Find the coefficient of restitution between A and B?
View SolutionIn a head on collision between two identical particles A and B moving on a horizontal surface, B is stationary and A has momentum P0 before impact. Due to the impact, A recrieves an impulse I. Then coefficient of restitution between the two is :
View SolutionA body X with a momentum p collides with another identical stationary body Y one dimensionally. During the collision, Y gives an impulse J to body X. Then coefficient of restitution is
View SolutionA sphere A of mass m, travelling with speed v, collides directly with a stationary sphere B. If A is brought to rest and B is given a speed V, find (a) the mass of B (b) the coefficient of restitution between A and B?
View SolutionBlock A has mass 40kg and B has 15kg and F is 500N parallel to smooth inclined plane.The system is moving together
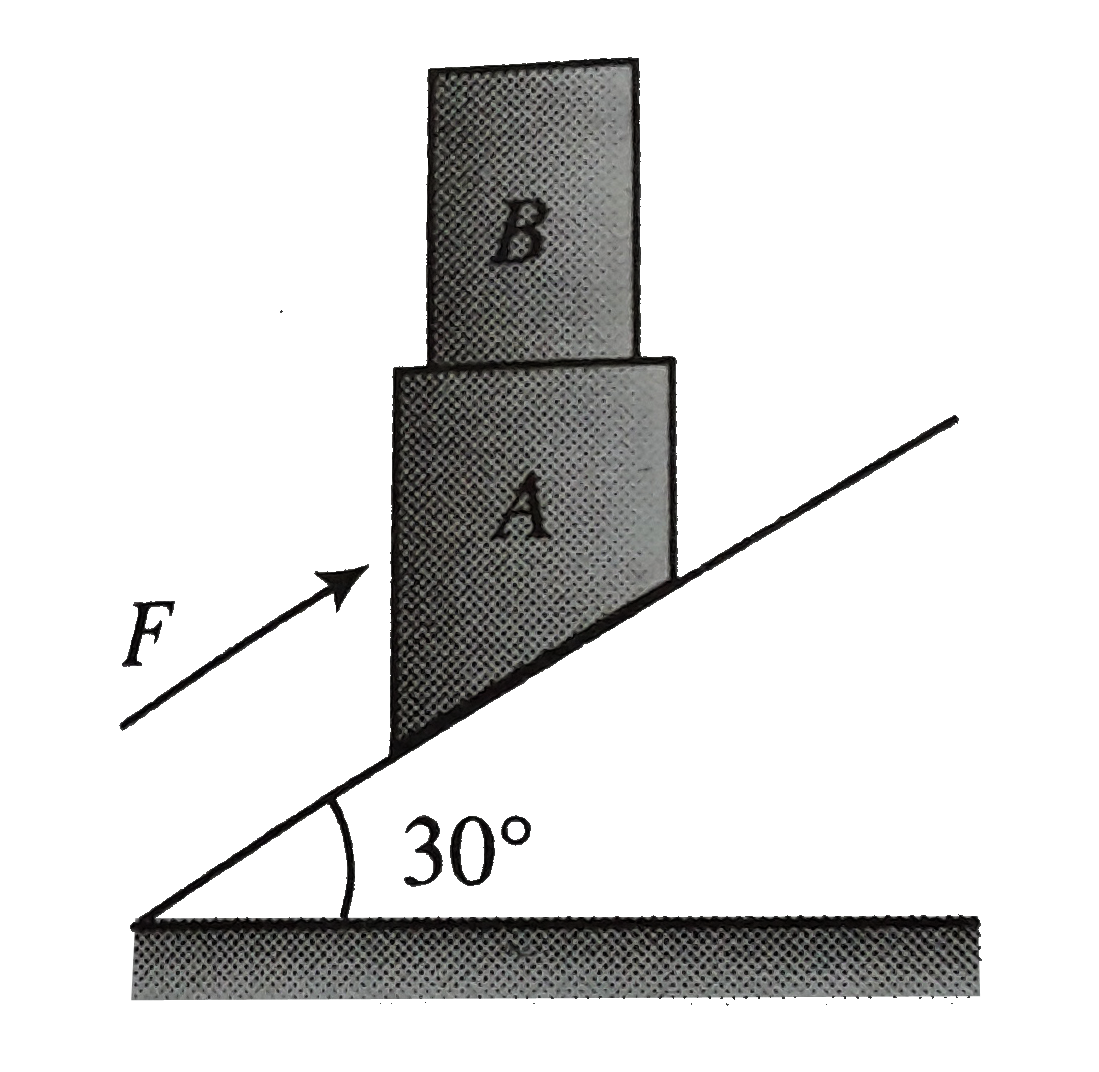
The least coefficient of friction between A and B is
View SolutionTwo equal spheres B and C, each of mass m, are in contact on a smooth horizontal table. A third sphere A of same size as that of B or C but mass m/2 impinges symmetrically on them with a velocity u and is itself brought to rest.
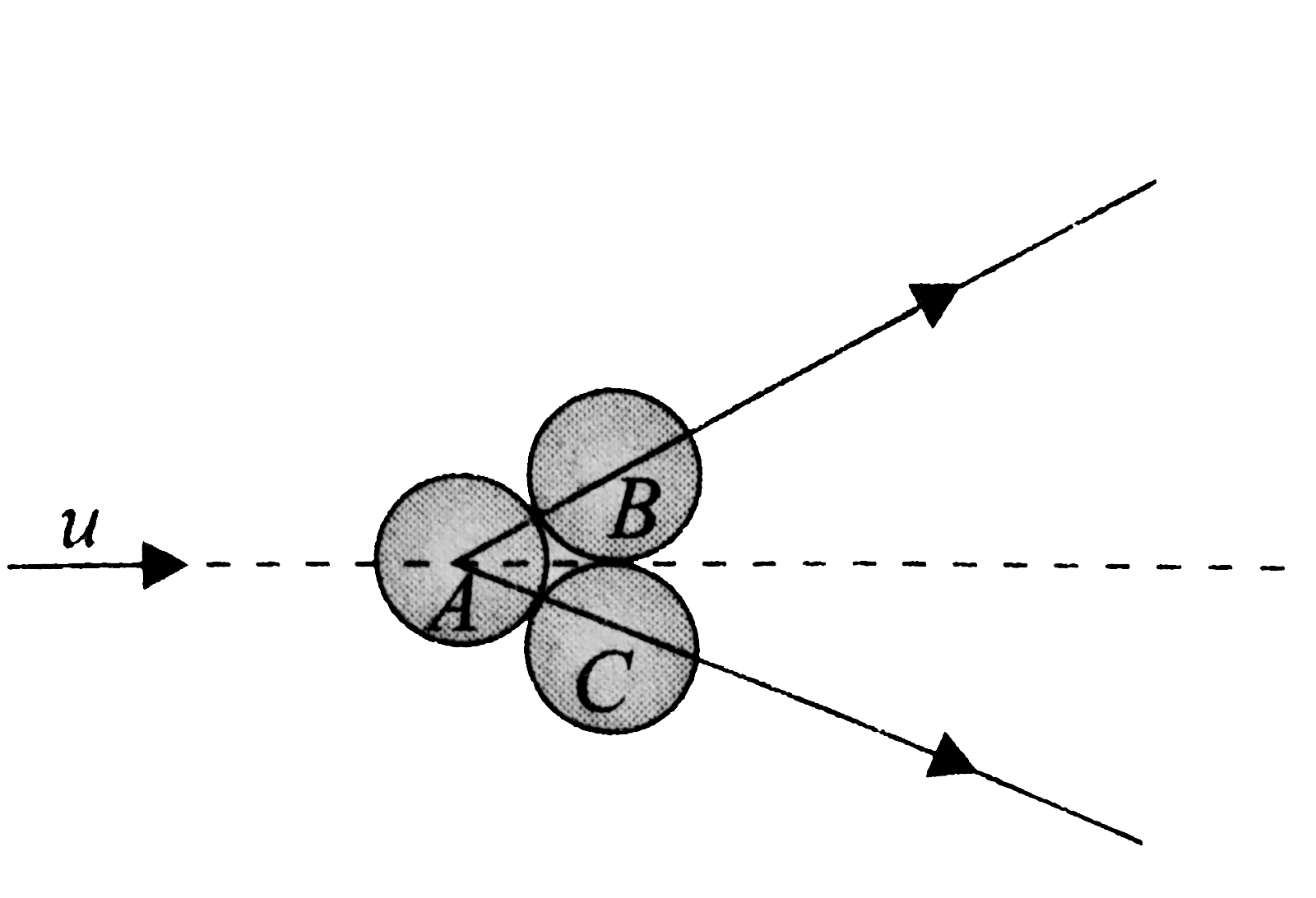
The coefficient of restitution between the two spheres A and B (or between A and C) is
View SolutionTwo smooth spheres. A and B. having equal radii, lie on a horizontal table. A is of mass m and B is of mass 3m. The spheres are projected towards each other with velocity vector 5ˆi+2ˆj and 2ˆi−ˆj, respectively, and when they collide the lige joining their centres is parallel to the vector ˆi. If the coefficient of restitution between A and B is 1/3, find the velocities after impact and the loss in kinetic energy caused by the collision. Find also the magnitude of the impulses that act at the instant of impact.
View Solution
