Consider a uniform square plate shown in the figure. I1,I2, I3 and I4 are moment of inertia of the plate about the axes 1, 2, 3 and 4 respectively. Axes 1 and 2 are diagonals and 3 and 4 are lines passing through centre parallel to sides of the square. The moment of inertia of the plate about an axis passing through centre and perpendicular to the plane of the figure is equal to which of the followings.
A. I3 + I4
B. I1 + I3
C. I2 + I3
D. 12 (I1+I2+I3+I4)
I3 + I4
I1 + I3
I2 + I3
1/2(I1+I2+I3+I4)
The correct Answer is:3
To solve the problem, we need to find the moment of inertia of a uniform square plate about an axis passing through its center and perpendicular to its plane. We will use the perpendicular axis theorem, which states that for a flat plate lying in the XY plane, the moment of inertia about an axis perpendicular to the plane (Iz) is equal to the sum of the moments of inertia about two perpendicular axes lying in the plane (Ix and Iy).
1. Identify the Axes and Moments of Inertia:
- Let I1 and I2 be the moments of inertia about the diagonals of the square plate.
- Let I3 and I4 be the moments of inertia about the axes parallel to the sides of the square plate.
2. Apply the Perpendicular Axis Theorem:
According to the perpendicular axis theorem:
Iz=Ix+Iy
where Iz is the moment of inertia about the axis perpendicular to the plane, and Ix and Iy are the moments of inertia about two perpendicular axes in the plane.
3. Relate the Moments of Inertia:
Since the square plate is uniform, we have:
I1=I2=I3=I4
This means that all the moments of inertia about the axes are equal.
4. Combine the Moments of Inertia:
From the previous step, we can express Iz in terms of I1 and I3:
Iz=I1+I2=I1+I1=2I1
Similarly, we can express it using I3 and I4:
Iz=I3+I4=I3+I3=2I3
5. Calculate Iz:
Now, if we add all four moments of inertia:
I1+I2+I3+I4=4I1
Thus, we can relate Iz to the total moment of inertia:
Iz=12(I1+I2+I3+I4)
6. Conclusion:
Therefore, the moment of inertia of the plate about an axis passing through the center and perpendicular to the plane is:
Iz=12(I1+I2+I3+I4)
This corresponds to option D.
Final Answer:
The moment of inertia of the plate about an axis passing through the center and perpendicular to the plane of the figure is equal to:
D. 12(I1+I2+I3+I4)
- Let I1 and I2 be the moments of inertia about the diagonals of the square plate.
- Let I3 and I4 be the moments of inertia about the axes parallel to the sides of the square plate.
According to the perpendicular axis theorem:
Iz=Ix+Iy
where Iz is the moment of inertia about the axis perpendicular to the plane, and Ix and Iy are the moments of inertia about two perpendicular axes in the plane.
Since the square plate is uniform, we have:
I1=I2=I3=I4
This means that all the moments of inertia about the axes are equal.
From the previous step, we can express Iz in terms of I1 and I3:
Iz=I1+I2=I1+I1=2I1
Similarly, we can express it using I3 and I4:
Iz=I3+I4=I3+I3=2I3
Now, if we add all four moments of inertia:
I1+I2+I3+I4=4I1
Thus, we can relate Iz to the total moment of inertia:
Iz=12(I1+I2+I3+I4)
Therefore, the moment of inertia of the plate about an axis passing through the center and perpendicular to the plane is:
Iz=12(I1+I2+I3+I4)
This corresponds to option D.
The moment of inertia of the plate about an axis passing through the center and perpendicular to the plane of the figure is equal to:
D. 12(I1+I2+I3+I4)
Similar Questions
The moment of inertia of a copper disc, rotating about an axis passing through its centre and perpendicular to its plane
View SolutionMoment of inertia of a ring of mass M and radius R about an axis passing through the centre and perpendicular to the plane is
View SolutionThe moment of inertia of ring about an axis passing through its diameter is I. Then moment of inertia of that ring about an axis passing through its centre and perpendicular to its plane is
View SolutionThe moment of inertia of a thin square plate ABCD of uniform thickness about an axis passing through the centre O and perpendicular to plate is
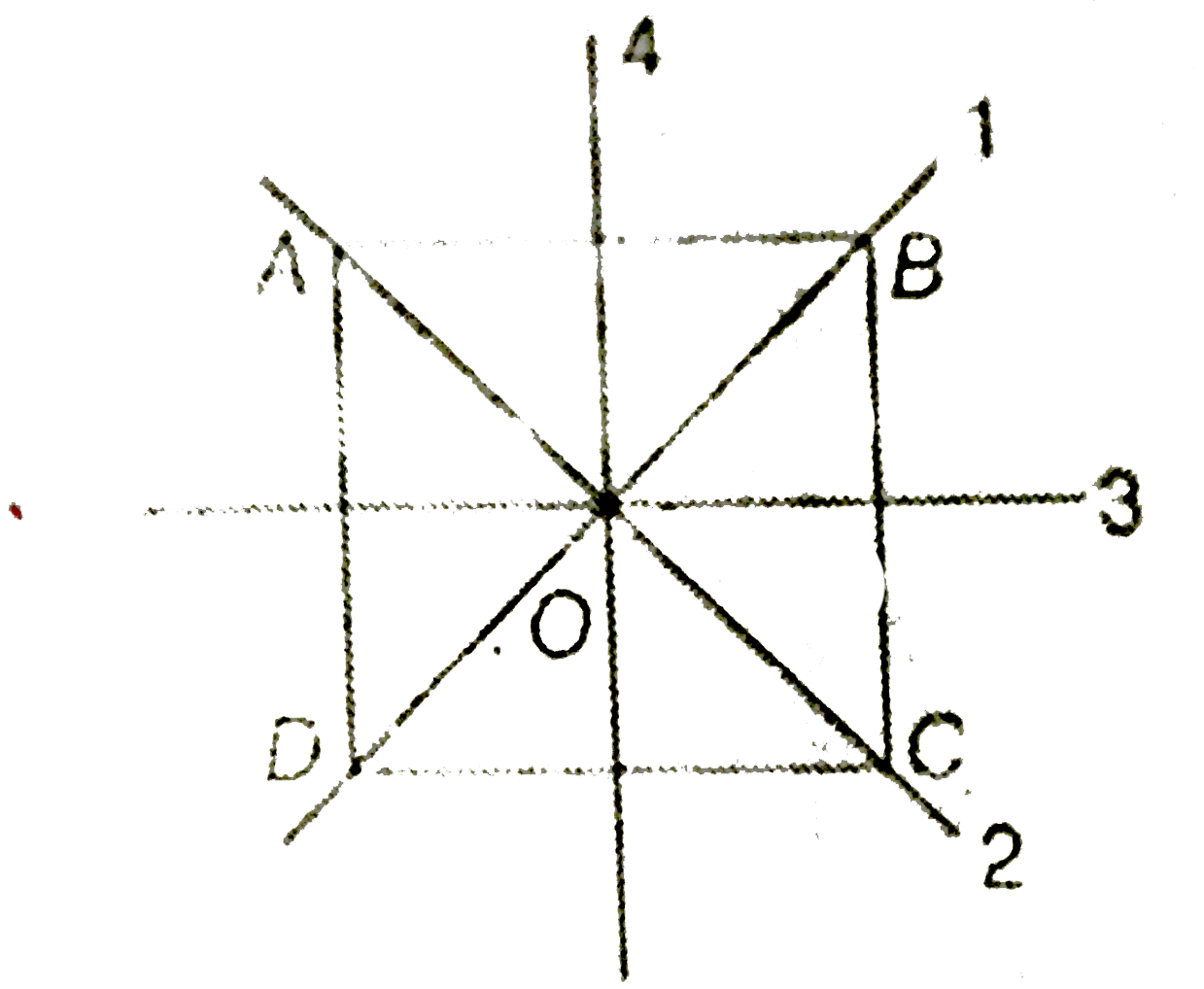
View SolutionIn the figure shown ABCD is a lamina of mass 12kg. The moment of inertia of the given body about an axis passing through D, and perpendicular to the plane of the body is
View SolutionFor the structure shown in figure, moment of inertia about an axis passing through yy' is
_PHY_213_Q01.png)
View SolutionFor the structure shown in figure, moment of inertia about an Axis passing through yy' is
_PHY_E05_238_Q01.png)
View SolutionFor the same total mass, which of the following will have the largest moment of inertia about an axis passing through the centre of mass and perpendicular to the plane of the body
View SolutionA hole of radius R/2 is removed from a thin circular plate of radius R and mass M. The moment of inertia of the remaining plate about an axis through centre O and perpendicular to the plane of the plate is

View SolutionThe moment of inertia of a circular ring of mass 1 kg about an axis passing through its centre and perpendicular to its plane is 4 kg m2. The diameter of the ring is
View Solution
