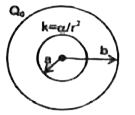
An ice ball of radius a and mass m is placed at $0^{\circ} C$ inside a thick hollow sphere of inner and outer radii a and b whose thermal conductivity varies as $k = \frac{α}{r^{2}}$ , where $α$ is a positive constant and r is the distance from centre. The temperature of surrounding is $θ_{0}$ and latent heat of fusion for ice is $L_{f}$ . Then , time required to completely melt the ice ball is (Neglect the contraction in volume when ice melts into water and any change in thermal resistance due to melting of ice of the system.)

$\frac{m L_{f} ln (\frac{b}{a})}{4 π α θ_{0}}$

$\frac{m L_{f (b - a)}}{2 π α θ_{0}}$

$\frac{m L_{f (b - a)}}{π α θ_{0}}$

$\frac{m L_{f (b - a)}}{4 π α θ_{0}}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

Updated on:21/07/2023

Answer

Step by step video & image solution for An ice ball of radius a and mass m is placed at 0^@C inside a thick hollow sphere of inner and outer radii a and b whose thermal conductivity varies as k=alpha/r^2, where alpha is a positive constant and r is the distance from centre. The temperature of surrounding is theta_0 and latent heat of fusion for ice is L_f. Then , time required to completely melt the ice ball is (Neglect the contraction in volume when ice melts into water and any change in thermal resistance due to melting of ice of the system.) by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 PHYSICS QUESTION BANK

Knowledge Check

A system consits of a uniformly charged sphere of radius R and a surrounding medium filled by a charge with the volume density $ρ = \frac{α}{r}$ , where $α$ is a positive constant and r is the distance from the centre of the sphere. Find the charge of the sphere for which the electric field intensity E outside the sphere is independent of R.
A $\frac{α}{2 ε_{0}}$
B $\frac{2}{α ε_{0}}$
C $2 π α R^{2}$
DNone of these

The correct Answer is:D

Similar Questions

Knowledge Check

Recommended Questions