A dielectric sphere of radius 10 cm has a charge of 3 mC distributed uniformly throughout its volume. The dielectric constant of the material of the sphere is 1. A bullet having charge 3 mc and mass 10 g is fired from the surface of the sphere along the diameter, directly towards its centre. Assuming the sphere to be fixed and neglecting any forces other than electrostatic force, the minimum velocity of the bulge so that it can emerge from the other side of the sphere is
3×103ms−1
3×106ms−1
9×106ms−1
9×103ms−1
The correct Answer is:D
To solve the problem, we need to find the minimum velocity of a charged bullet so that it can emerge from the other side of a uniformly charged dielectric sphere. Here are the steps to derive the solution:
Step 1: Understand the Problem
We have a dielectric sphere with a radius R=10cm=0.1m and a total charge Q=3mC=3×10−3C. A bullet with charge q=3mC=3×10−3C and mass m=10g=10×10−3kg is fired from the surface of the sphere towards its center.
Step 2: Calculate the Electric Field Inside the Sphere
Using Gauss's law, the electric field E inside the sphere at a distance r from the center is given by:
E=Q⋅r4πϵ0R3
where ϵ0 is the permittivity of free space, approximately 8.85×10−12C2/N⋅m2.
Step 3: Calculate the Force on the Bullet
The force F experienced by the bullet due to the electric field is:
F=q⋅E=q⋅Q⋅r4πϵ0R3
Step 4: Work Done Against the Electric Force
The work done W against the electric force as the bullet moves from the surface to the center of the sphere is given by:
W=∫0RFdr=∫0R(q⋅Q⋅r4πϵ0R3)dr
Substituting the expression for F:
W=qQ4πϵ0R3∫0Rrdr
Calculating the integral:
∫rdr=r22∣∣0R=0−R22=−R22
Thus,
W=−qQ4πϵ0R3⋅(−R22)=qQR28πϵ0R3=qQ8πϵ0R
Step 5: Set the Work Done Equal to Kinetic Energy
The initial kinetic energy KE of the bullet is given by:
KE=12mv2
Setting the work done equal to the kinetic energy:
12mv2=qQ8πϵ0R
Step 6: Solve for Velocity v
Rearranging the equation to solve for v2:
v2=qQ4πϵ0mR
Taking the square root gives:
v=√qQ4πϵ0mR
Step 7: Substitute the Values
Substituting the known values:
- q=3×10−3C
- Q=3×10−3C
- m=10×10−3kg
- R=0.1m
- ϵ0=8.85×10−12C2/N⋅m2
Calculating v:
v=
⎷(3×10−3)(3×10−3)4π(8.85×10−12)(10×10−3)(0.1)
Calculating the value gives:
v≈9×103m/s
Final Answer
The minimum velocity of the bullet so that it can emerge from the other side of the sphere is approximately:
v≈9000m/s
We have a dielectric sphere with a radius R=10cm=0.1m and a total charge Q=3mC=3×10−3C. A bullet with charge q=3mC=3×10−3C and mass m=10g=10×10−3kg is fired from the surface of the sphere towards its center.
Using Gauss's law, the electric field E inside the sphere at a distance r from the center is given by:
E=Q⋅r4πϵ0R3
where ϵ0 is the permittivity of free space, approximately 8.85×10−12C2/N⋅m2.
The force F experienced by the bullet due to the electric field is:
F=q⋅E=q⋅Q⋅r4πϵ0R3
The work done W against the electric force as the bullet moves from the surface to the center of the sphere is given by:
W=∫0RFdr=∫0R(q⋅Q⋅r4πϵ0R3)dr
Substituting the expression for F:
W=qQ4πϵ0R3∫0Rrdr
Calculating the integral:
∫rdr=r22∣∣0R=0−R22=−R22
Thus,
W=−qQ4πϵ0R3⋅(−R22)=qQR28πϵ0R3=qQ8πϵ0R
The initial kinetic energy KE of the bullet is given by:
KE=12mv2
Setting the work done equal to the kinetic energy:
12mv2=qQ8πϵ0R
Rearranging the equation to solve for v2:
v2=qQ4πϵ0mR
Taking the square root gives:
v=√qQ4πϵ0mR
Substituting the known values:
- q=3×10−3C
- Q=3×10−3C
- m=10×10−3kg
- R=0.1m
- ϵ0=8.85×10−12C2/N⋅m2
v=
⎷(3×10−3)(3×10−3)4π(8.85×10−12)(10×10−3)(0.1)
Calculating the value gives:
v≈9×103m/s
The minimum velocity of the bullet so that it can emerge from the other side of the sphere is approximately:
v≈9000m/s
Similar Questions
A spherical conductor of radius 10 cm has a charge of 3.2 x 10^-7 C distributed uniformly. What is magnitude of electric field at point 15 cm from centre of sphere?
View SolutionA non-conducting sphere of radius R is given a charge Q. Charge is uniformly distributed in its volume. The electric potential at centre and at the surface of sphere respectively are
View SolutionA solid sphere of radius R is charged uniformly through out the volume. At what distance from its surface is the electric potential 1/4 of the potential at the centre?
View SolutionA spherical conductor of radius 12 cm has a charge of 1.6×10−7C distributed uniformly on its surface. What is the electric field (a) inside the sphere (b) just outside the sphere (c ) at a point 18 cm from the center of the sphere ?
View SolutionFind the electric work done in bringing a charge q from A to B in a sphere of charge Q distributed uniformly throughout its volume.
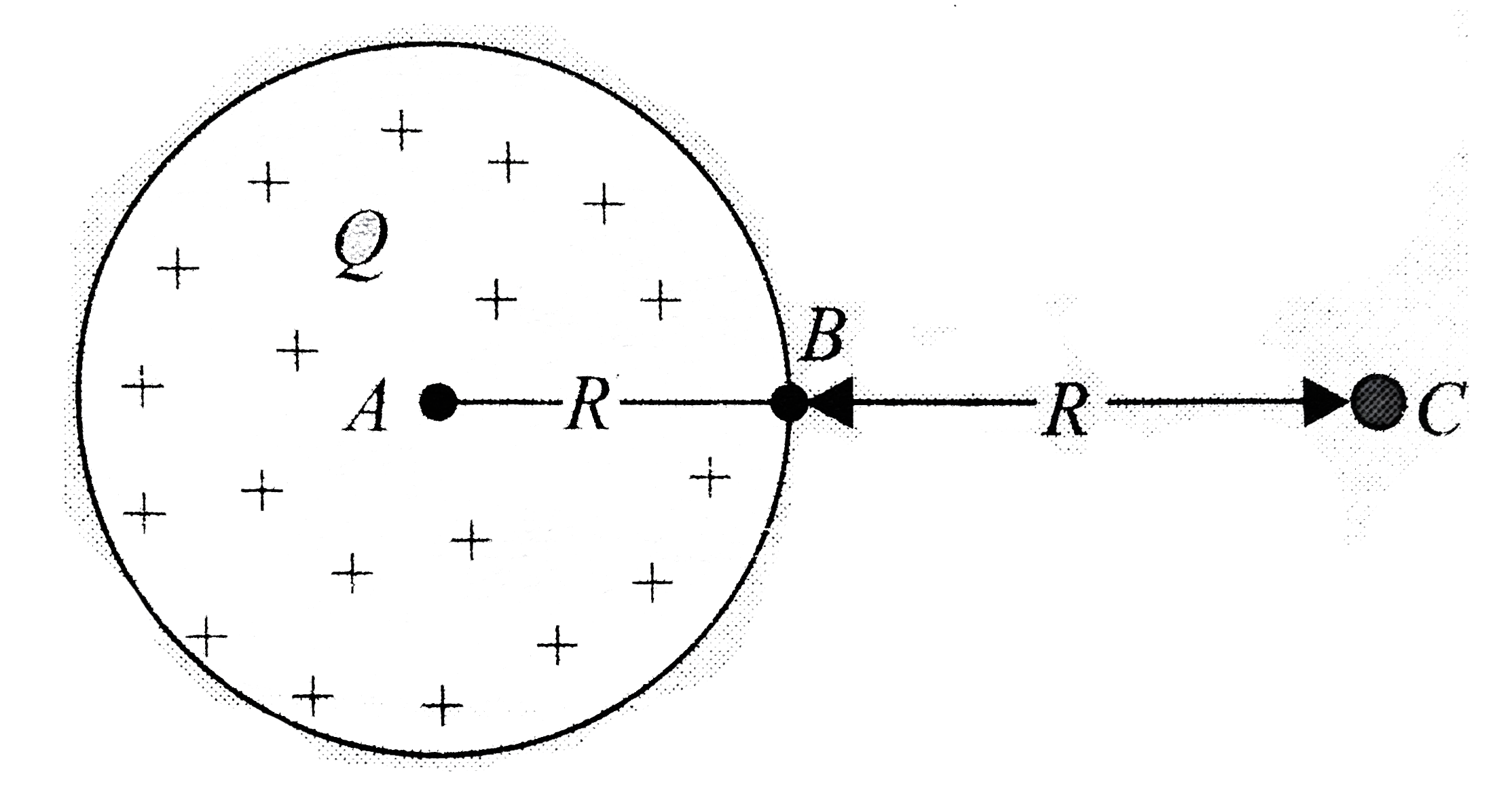 .
.
View SolutionA solid sphere of radius R has charge q uniformly distributed over its volume. The distance from it surfce at which the electrostatic potential is equal to half of the potential at the centre is
View SolutionA solid sphere of radius R has charge q uniformly distributed over its volume. The distance from it surfce at which the electrostatic potential is equal to half of the potential at the centre is
View SolutionA hollow metal sphere of radius 5 cm is charged such that the potential on its surface is 10V. The potential at the distance 3cm from the centre of the sphere is:
View SolutionA hollow metal sphere of radius 10 cm is charged such that the potential on its surface is 5 V. What is the potential at the centre of the sphere?
View SolutionA hollow metal sphere of radius 10 cm is charged such that the potential on its surface is 5 V. What is the potential at the centre of the sphere?
View Solution
