A ball is projected from the floor of a cabin of height 7m with speed 20 m/s at an angle of 37∘ with the floor of cabin. It makes a successive collision with the wall of cabin and then return again to its floor. Assume all collisions are perfectly elastic. Find time taken (in sec) by the ball to reach the floor after collisions with the ceiling of cabin.
The correct Answer is:1
To solve the problem step by step, we will break down the motion of the ball and analyze the time taken for it to reach the floor after colliding with the ceiling of the cabin.
Step 1: Determine the initial velocity components
The ball is projected with an initial speed u=20m/s at an angle θ=37∘ with respect to the horizontal. We need to find the horizontal and vertical components of the initial velocity.
- The vertical component uy is given by:
uy=usinθ=20sin(37∘)
- The horizontal component ux is given by:
ux=ucosθ=20cos(37∘)
Using sin(37∘)≈0.6 and cos(37∘)≈0.8:
uy=20×0.6=12m/s
ux=20×0.8=16m/s
Step 2: Calculate the time to reach the ceiling
The height of the cabin is h=7m. We need to find the time t1 it takes for the ball to reach the ceiling using the following kinematic equation:
s=uyt+12at2
Here, s=7m, uy=12m/s, and a=−g=−10m/s2 (acceleration due to gravity).
Substituting the values:
7=12t−5t2
Rearranging gives:
5t2−12t+7=0
Step 3: Solve the quadratic equation
Using the quadratic formula t=−b±√b2−4ac2a:
t=12±√(−12)2−4⋅5⋅72⋅5
Calculating the discriminant:
=√144−140=√4=2
Thus, the time values are:
t=12±210
Calculating both possible times:
1. t1=1410=1.4s
2. t2=1010=1.0s
Step 4: Determine the time taken after collision with the ceiling
After reaching the ceiling, the ball will reverse its vertical velocity and fall back down. The velocity just before hitting the ceiling is vy=uy−gt1:
vy=12−10⋅1.4=−1m/s
Now, we need to find the time t3 it takes to fall back to the floor from the ceiling. The ball will fall a distance of 7m with an initial velocity of 1m/s (downwards).
Using the same kinematic equation:
s=vyt+12gt2
Substituting s=7m, vy=−1m/s, and g=10m/s2:
7=−1t+5t2
Rearranging gives:
5t2+t−7=0
Step 5: Solve the new quadratic equation
Using the quadratic formula again:
t=−1±√12−4⋅5⋅(−7)2⋅5
Calculating the discriminant:
=√1+140=√141
Thus, the time values are:
t=−1±√14110
Calculating the positive root gives:
t≈−1+11.8310≈0.983s
Final Step: Total time after collision with the ceiling
The total time taken by the ball to reach the floor after colliding with the ceiling is:
ttotal=t1+t3≈1.4+0.983≈2.383s
Final Answer
The time taken by the ball to reach the floor after the collision with the ceiling is approximately 2.38s.
The ball is projected with an initial speed u=20m/s at an angle θ=37∘ with respect to the horizontal. We need to find the horizontal and vertical components of the initial velocity.
uy=usinθ=20sin(37∘)
- The horizontal component ux is given by:
ux=ucosθ=20cos(37∘)
uy=20×0.6=12m/s
ux=20×0.8=16m/s
The height of the cabin is h=7m. We need to find the time t1 it takes for the ball to reach the ceiling using the following kinematic equation:
s=uyt+12at2
Here, s=7m, uy=12m/s, and a=−g=−10m/s2 (acceleration due to gravity).
7=12t−5t2
Rearranging gives:
5t2−12t+7=0
Using the quadratic formula t=−b±√b2−4ac2a:
t=12±√(−12)2−4⋅5⋅72⋅5
Calculating the discriminant:
=√144−140=√4=2
Thus, the time values are:
t=12±210
Calculating both possible times:
1. t1=1410=1.4s
2. t2=1010=1.0s
After reaching the ceiling, the ball will reverse its vertical velocity and fall back down. The velocity just before hitting the ceiling is vy=uy−gt1:
vy=12−10⋅1.4=−1m/s
Now, we need to find the time t3 it takes to fall back to the floor from the ceiling. The ball will fall a distance of 7m with an initial velocity of 1m/s (downwards).
s=vyt+12gt2
Substituting s=7m, vy=−1m/s, and g=10m/s2:
7=−1t+5t2
Rearranging gives:
5t2+t−7=0
Using the quadratic formula again:
t=−1±√12−4⋅5⋅(−7)2⋅5
Calculating the discriminant:
=√1+140=√141
Thus, the time values are:
t=−1±√14110
Calculating the positive root gives:
t≈−1+11.8310≈0.983s
The total time taken by the ball to reach the floor after colliding with the ceiling is:
ttotal=t1+t3≈1.4+0.983≈2.383s
The time taken by the ball to reach the floor after the collision with the ceiling is approximately 2.38s.
Similar Questions
A particle is projected from a horizontal floor with speed 10m/s at an angle 30∘ with the floor and striking the floor after sometime. State which is correct.
View SolutionA particle is projected from a horizontal floor with speed 10ms at an angle 30∘ with the floor and striking the floor after sometime. State which is correct.
View SolutionA ball is shot in a long hall having a roof at a height of 10m with 25m/s at an angle of 37∘ with the floor. The ball lands on the floor at a distance of ………………….. from the point of projection. ( Assume elastic collisions if any )
 View Solution
View SolutionA ball of mass m moving horizontally at a speed v collides with the bob of a simple pendulum at rest. The mass of the bob is also m. If the collision is perfectly elastic and both balls sticks, the height to which the two balls rise after the collision will be given by:
View SolutionA ball is projected from the point O with velocity 20 m/s at an angle of 60∘ with horizontal as shown in figure. At highest point of its trajectory it strikes a smooth plane of inclination 30∘ at point A. The collision is perfectly inelastic. The maximum height from the ground attained by the ball is (g=10m/s2)
 View Solution
View SolutionA particle is projected from the ground with speed u at angle 60∘ from horizontal. It collides with a second particle of same mass moving with horizontal speed u in same direction at highest point of its trajectory. If collision is perfectly inelastic then find horizontal distance travelled by them after collision when they reached at ground
View SolutionA ball is projected on a very long floor. There may be two conditions (i) floor is smooth &(ii) the collision is elastic
If both the considered then the path of ball is as follows.
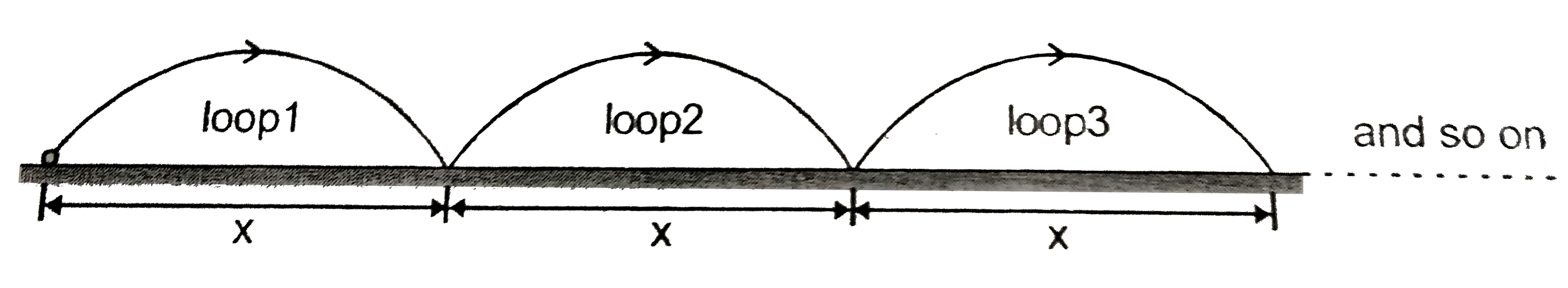
Now if collision is inelastic and surface is rough then the path is as follows.
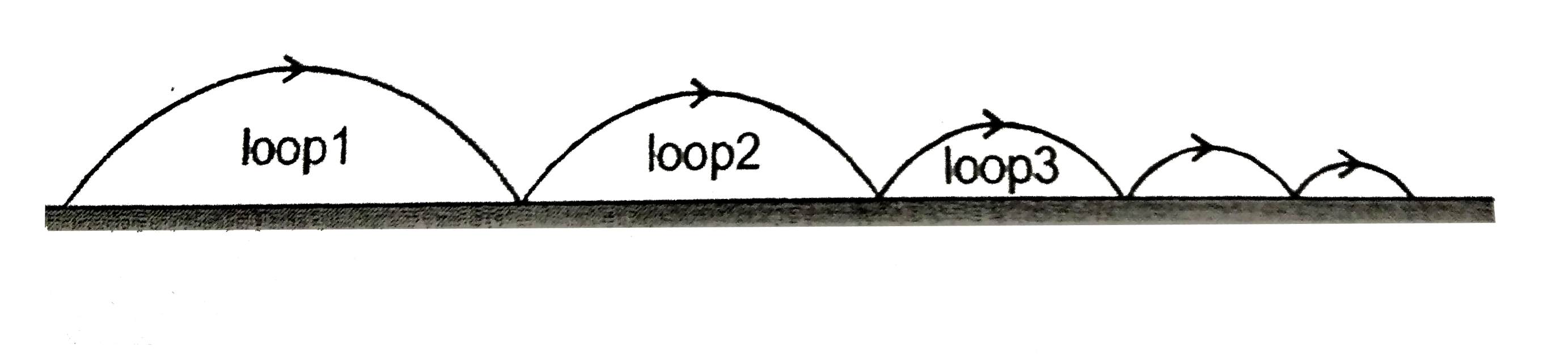
Successive range is decreasing .
Roughness of surface decreases the horizontal component of ball during collision and inelastic nature of collision decreases the vertical component of velocity of ball. In first case both components remain unchanged in magnitude and in second case both the components of the velocity will change.
Let us consider a third case here surface is rough but the collision of ball with floor is elastic. A ball is projected withe speed u at an angle 30∘ with horizontal and it is known that after collision with the floor its speed becomes u√3 . Then answer the following questions.
If the ball after first collision with the floor has rebounded vertically then the speed of the ball just after the collision with the floor would have been :View SolutionThe two balls shown in figure are identical the first moving at a speed v towards right and the second staying at rest. The wall at the extreme right is fixed. Assume all collisions to be elastic. Show that the speeds of the balls remain unchanged after all the collisions have taken place.
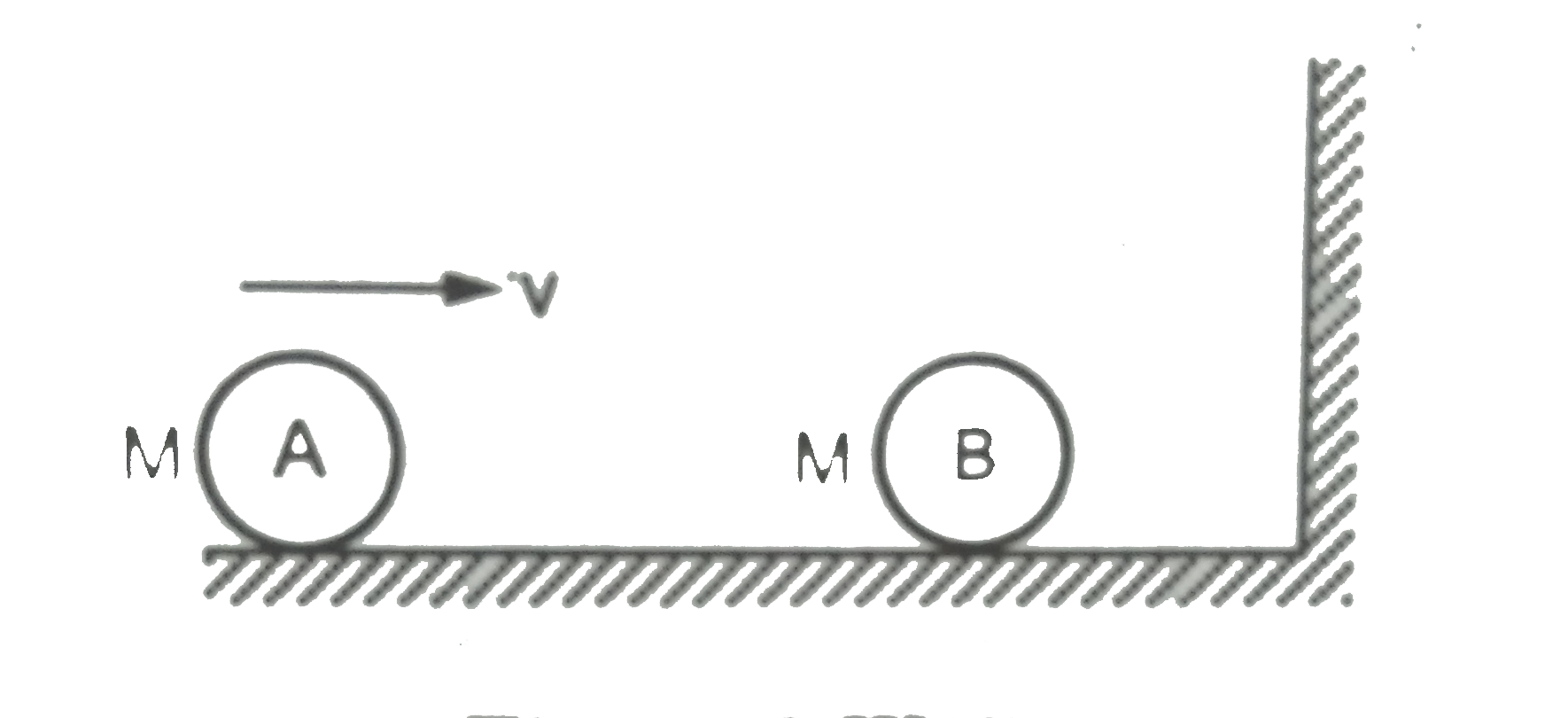 View Solution
View SolutionThe two balls shwon in figure are indentical the first moving at a speed v towards right and the second staying at rest. The wall at the extreme right is fixed. Assume all collisions to be elastic. Show that the speeds of the balls remain unchanged after all the collisions have taken place.
 View Solution
View SolutionA massive vertical wall is approaching a man at a speed μ. When it is at a distance of 10m, the man throws a ball with speed 10 m/s at an angle of 37∘, which after having a completely elastic collision with the wall, reaches back directly into the hands of the man. The velocity of the wall is
View Solution

