Current I A flowing in a circular coil of radius r. then the magnetic induction at the centre is B. If the current is doubled then the magnetic induction will be
To solve the problem, we need to understand how the magnetic induction (magnetic field) at the center of a circular coil changes when the current flowing through it is doubled.
1. Understanding the Formula:
The magnetic induction B at the center of a circular coil is given by the formula:
B=μ0I2R
where:
- B is the magnetic induction,
- μ0 is the permeability of free space,
- I is the current flowing through the coil,
- R is the radius of the coil.
2. Initial Condition:
Initially, the current I is given as 1 A. Plugging this into the formula, we have:
B=μ0⋅12R=μ02R
3. Doubling the Current:
Now, if the current is doubled, the new current I′ becomes:
I′=2I=2⋅1=2 A
4. Calculating New Magnetic Induction:
We will now calculate the new magnetic induction B′ using the doubled current:
B′=μ0I′2R=μ0⋅22R
5. Simplifying the Expression:
Simplifying the above expression, we find:
B′=2μ02R=μ0R
6. Relating New Magnetic Induction to Initial Induction:
We can relate B′ to the initial magnetic induction B:
B′=2⋅μ02R=2B
7. Conclusion:
Therefore, when the current is doubled, the magnetic induction at the center of the coil also doubles. Thus, the final answer is:
B′=2B
The magnetic induction B at the center of a circular coil is given by the formula:
B=μ0I2R
where:
- B is the magnetic induction,
- μ0 is the permeability of free space,
- I is the current flowing through the coil,
- R is the radius of the coil.
Initially, the current I is given as 1 A. Plugging this into the formula, we have:
B=μ0⋅12R=μ02R
Now, if the current is doubled, the new current I′ becomes:
I′=2I=2⋅1=2 A
We will now calculate the new magnetic induction B′ using the doubled current:
B′=μ0I′2R=μ0⋅22R
Simplifying the above expression, we find:
B′=2μ02R=μ0R
We can relate B′ to the initial magnetic induction B:
B′=2⋅μ02R=2B
Therefore, when the current is doubled, the magnetic induction at the center of the coil also doubles. Thus, the final answer is:
B′=2B
Similar Questions
Current 'i' is flowing in heaxagonal coil of side a. The magnetic induction at the centre of the coil will be
View SolutionIn the figure shown, the magnetic induction at the centre of the arc due to the current in portion AB will be

View SolutionDue to the flow of current in a circular loop of radius R, the magnetic induction produced at the centre of the loop is B. The magnetic moment of the loop is (μ0=permeability constant)
View SolutionThe magnetic induction at the point O, if the wire carries a current I, is
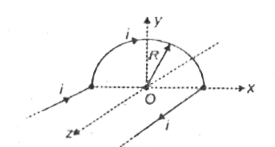
View SolutionIf the current flowing in a circular loop is in clockwise directions, then the magnetic induction will be
View SolutionA circular coil of n turns and radius r carries a current I. The magnetic field at the centre is
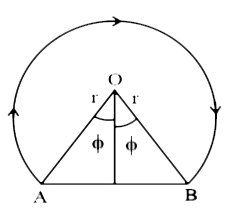
View SolutionA wire is bent in the form of a circular arc of radius r with a straight portion AB. If the current in the wire is i, then the magnetic induction at point O is
View SolutionAn electron having kinetic energy K is moving i a circular orbit of radius R perpendicular to a uniform magnetic induction. If kinetic energy is douled and magnetic induction tripled, the radius will become
View SolutionThe current I ampere is flowing in an equilateral triangle of side a the magnetic field induction at the centroid will be
View SolutionA current i is flowing in a straight conductor of length L. The magnetic induction at a point distant L4 from its centre will be-
View Solution
