Pressure of monoatomic gas in a container is 2 atm. Average KE per molecule is 2 x 10-9J. Volume of gas is 1litre. Find number of molecules of gas present in the container
32x1011
32x1010
52x1012
52x1011
To find the number of molecules of a monoatomic gas present in a container, we can use the relationship between pressure, volume, and kinetic energy. Here’s a step-by-step solution:
Step 1: Understand the Given Data
- Pressure (P) = 2 atm
- Average Kinetic Energy per molecule (KE) = 2 x 10^-9 J
- Volume (V) = 1 liter = 1 x 10^-3 m³ (since 1 liter = 10^-3 m³)
Step 2: Convert Pressure to SI Units
Pressure in SI units (P in Pascals):
P=2atm×1.013×105Pa/atm=2.026×105Pa
Step 3: Use the Relation Between Pressure, Volume, and Number of Molecules
The pressure of an ideal gas can be expressed as:
P=13N⋅m⋅v2V
Where:
- N = number of molecules
- m = mass of one molecule
- v = average speed of molecules
Step 4: Relate Kinetic Energy to Average Speed
The average kinetic energy (KE) of a molecule is given by:
KE=12mv2
From this, we can express v2:
v2=2⋅KEm
Step 5: Substitute v2 into the Pressure Equation
Substituting v2 into the pressure equation:
P=13N⋅m⋅(2⋅KEm)V
This simplifies to:
P=2N⋅KE3V
Step 6: Rearrange to Find Number of Molecules (N)
Rearranging the equation to solve for N:
N=3PV2⋅KE
Step 7: Substitute the Values
Now, substituting the values we have:
N=3⋅(2.026×105Pa)⋅(1×10−3m3)2⋅(2×10−9J)
Calculating:
N=3⋅2.026×105⋅10−34×10−9
N=6.078×1024×10−9
N=1.5195×1011
Step 8: Final Result
Thus, the number of molecules of gas present in the container is approximately:
N≈1.52×1011
- Pressure (P) = 2 atm
- Average Kinetic Energy per molecule (KE) = 2 x 10^-9 J
- Volume (V) = 1 liter = 1 x 10^-3 m³ (since 1 liter = 10^-3 m³)
Pressure in SI units (P in Pascals):
P=2atm×1.013×105Pa/atm=2.026×105Pa
The pressure of an ideal gas can be expressed as:
P=13N⋅m⋅v2V
Where:
- N = number of molecules
- m = mass of one molecule
- v = average speed of molecules
The average kinetic energy (KE) of a molecule is given by:
KE=12mv2
From this, we can express v2:
v2=2⋅KEm
Substituting v2 into the pressure equation:
P=13N⋅m⋅(2⋅KEm)V
This simplifies to:
P=2N⋅KE3V
Rearranging the equation to solve for N:
N=3PV2⋅KE
Now, substituting the values we have:
N=3⋅(2.026×105Pa)⋅(1×10−3m3)2⋅(2×10−9J)
Calculating:
N=3⋅2.026×105⋅10−34×10−9
N=6.078×1024×10−9
N=1.5195×1011
Thus, the number of molecules of gas present in the container is approximately:
N≈1.52×1011
Topper's Solved these Questions
- JEE MAIN 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2021EXERCISE - SECTION-A
80 Videos
- JEE MAIN 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2021EXERCISE - SECTION-B
40 Videos
- JEE MAINBOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINEXERCISE - All Questions
473 Videos
- JEE MAIN 2022BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2022EXERCISE - Question
492 Videos
Similar Questions
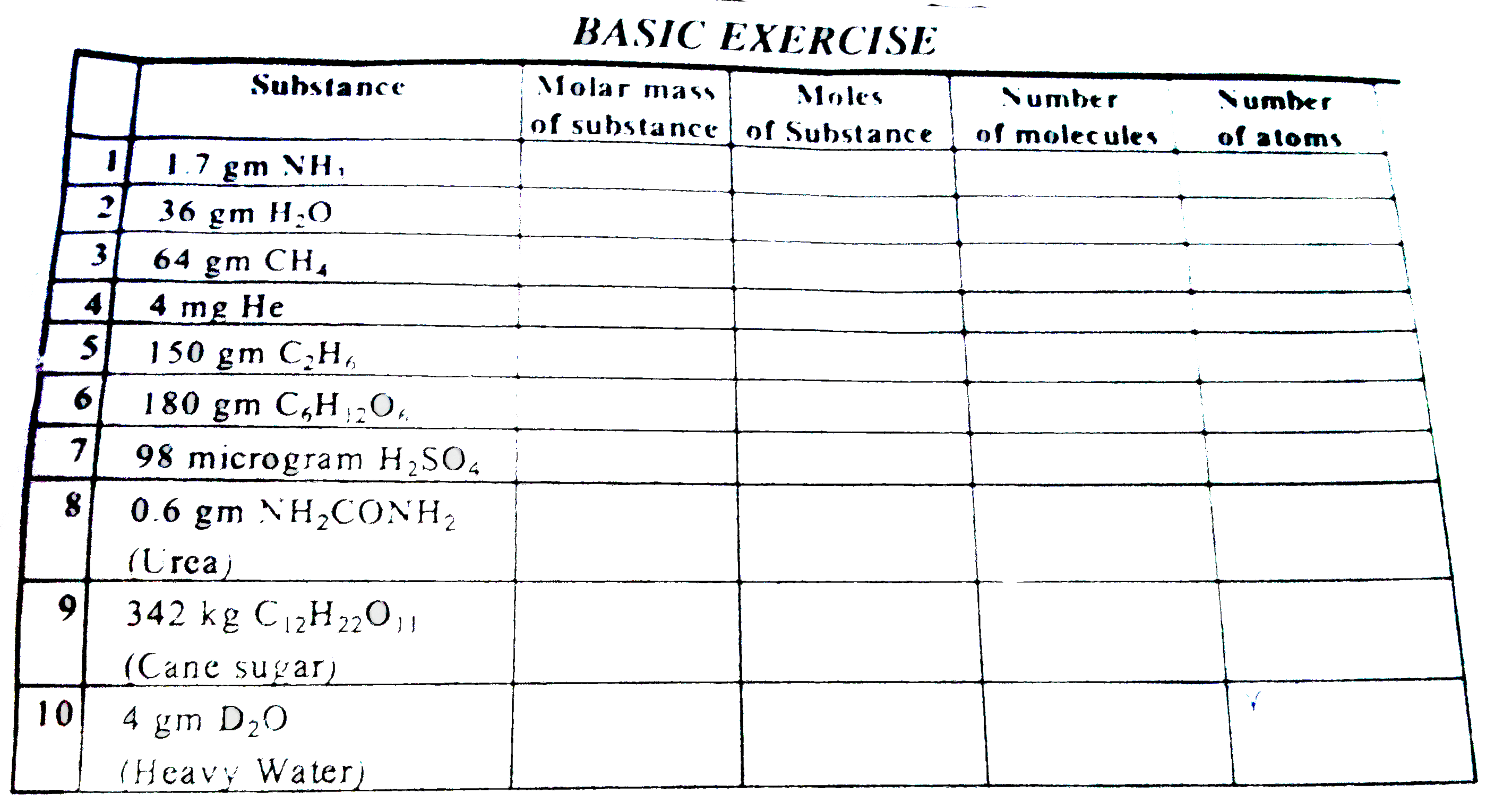
Find number of molecules in 11.35 litres SO2 gas at STP.
View SolutionThe pressure of a gas in a 100mL container is 200kPa and the. Average translational kinetic energy of each gas particle is 6×10−21J. Find the number of gas particles in the container. How many moles are there in the container ?
View Solution
Knowledge Check
A monoatomic gas is kept in a 1 litre container at pressure 1 atm. If average energy per molecule is 2x10−9J. Find number of molecules in the container.
A0.75x10−6B0.75x109C0.5x10−11D0.75x1011A container consists of O2 gas at 4 atm pressure and 400K. If the volume of the container is 8.21 litre then calculte number of oxygen atoms present in the container.
A2B1.5×NAC6.023×1023D12.04×1023If pressure and temperature of an ideal gas are doubled and volume is halved, the number of molecules of the gas
ABecome halfBBecome two timesCBecome four timesDRemain constant
Find the degrees of monoatomic, diatomic and triatomic gas molecules.
View SolutionDoes the average K.E. per molecule of the gas depend upon the mass of the molecule ?
View SolutionCalculate the mass of SO2 gas which will contain the same number of molecules as present in 4.4 g of CO2.
View SolutionA box contains x molecules of a gas. How will the pressure of the gas be effected if the number of molecules is made 2x?
View SolutionIf pressure and temperature of an ideal gas are doubled and volume is halved, the number of molecules of the gas
View Solution
