Derive an expression for electric potential at any point due to an electric dipole. Show that the potential at a point on the equitorial line of an electric dipole is zero.
Text Solution
Consider an electric dipole AB consisting of two charges -q and +q. Let P be the point where we have to calculate the electric potential and O be the centre of dipole.
Let OP=r, ∠BOP=θ and AB=2a.
Let BP=r1 and AP=r2.
The potential at point P due to +q charge is given by
V1=14πε0qr1.........(i)
The potential at point P due to -q charge is given by
V2=14πε0(−q)r2
or V2=−14πε0qr2...........(ii)
According to superposition principle the net potential at point P is given by
V=V1+V2
or V=14πεoln0qr1−14πε0qr2 [using equations (i) and (ii)]
or V=q4πε0[1r1−1r2]............(iii)
Now draw AC perpendicular to extended segment of PO and BD perpendicular to PO as shown in the figure.
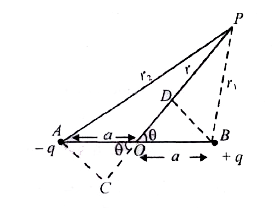
In ΔAOC, cosθ=OCOA⇒OC=OAcosθ=acosθ
In ΔBOD, cosθ=ODOB⇒OD=OBcosθ=acosθ
Now r1=BP≈PD=OP−OD=r−acosθ...........(iv)
r2=AP≈CP=OP+OC=r+acosθ .................(v)
Using equations (iv) and (v) , equation (iii) becomes
V=q4πε0[1r−acosθ−1r+acosθ]=q4πε0[r+acosθ−r+acosθr2−a2cos2θ]
or V=q4πε0[2acosθr2−a2cos2θ]=14πε0[(q×2a)cosθr2−a2cos2θ]
or V=14πε0[pcosθr2−a2cos2θ] [∴p=q×2a]..........(vi)
(i) Axial Line : When point P is on axial line , then θ=0∘.
So equation (vi) becomes
Va=14πε0=pcosθ(r2−a2cos2θ)=14πε0p(r2−a2)
(ii) Equatorial Line : When point P is on equatorial line, then θ=90∘, so equation (vi) becomes
Veq=14πε0pcos90∘(r2−a2cos290∘)=0
So electric potential on equatorial line is zero.
|
Updated on:21/07/2023
Similar Questions
Knowledge Check
- Question 1 - Select One
The electric potential at a point on the equatorial line of a electric dipole is
Adirectly proportional to the square of the distanceBindirectly proportional to the square of the distanceCdirectly proportional to the chargeDnone of the above - Question 2 - Select One
The value of electric potential at any point due to any electric dipole is
Ak.→p×→rr2Bk.→p×→rr3Ck.→p.→rr2Dk.→p.→rr3
Derive an expression for the electric potential at any point along the axial line of an electric dipole.
View SolutionDerive the expression for the electric potential at any point along the axial line of an electric dipole.
View SolutionDerive the expression for the electric potential at any point along the axial line of an electric dipole.
View SolutionDeive an expression for the electric field at any point on the equatorial line of an electric dipole.
View SolutionDerive the expression for the electric potential at any point along the axial line of an electric dipole.
View SolutionDerive an expression for the electric field at any point along the axial line of an electrical dipole.
View Solution