Show that electric field everywhere is normal to the equipotential surface .
We know, potential difference between any two points M and N
= − line integral of electric field
i.e., dV=−∫NM¯¯¯E.¯¯¯dl.
Now, for an equipotential surface, dV= 0
∴∫NM¯¯¯E.¯¯¯dl=0
Or Edlcosθ=0
This is possible when θ=90∘[∴cos90∘=0]
Hence →E⊥→dl .
i.e., electric field is directed perpendicular to the surface .
Similar Questions
On the basis of your understanding of the following paragraph and the related studied concepts.
An equipotential surface is a surface with a constant value of electric potential at all points on the surface. The component of electric field parallel to an equipotential surface is zero, as the potential does not change in this direction. Thus, the electric field is perpendicular to the equipotential surface at each point of the surface. Further, say the direction of electric field electrical potential gradually fall with distance.
Draw equipotential surfaces around an isolated positive point charge + q. Mark direction of electric field →E also.View SolutionOn the basis of your understanding of the following paragraph and the related studied concepts.
An equipotential surface is a surface with a constant value of electric potential at all points on the surface. The component of electric field parallel to an equipotential surface is zero, as the potential does not change in this direction. Thus, the electric field is perpendicular to the equipotential surface at each point of the surface. Further, say the direction of electric field electrical potential gradually fall with distance.
Four equipotential surfaces are shown in the adjoining figure. Find the magnitude and direaction of the electric field.
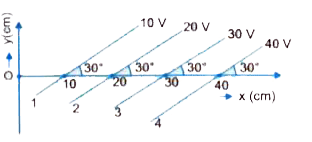 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
The electric field lines coming out of an equipotential surface are
Aperpendicular to the surfaceBparallel to the surfaceCin all directionsDzero - Question 2 - Select One
The angle between the equipotential surface and the electric field (or line of force) at any point on the equipotential surface is
A90∘ alwaysB0∘ alwaysC0∘ to 90∘D0∘ to 180∘
On the basis of your understanding of the following paragraph and the related studied concepts.
An equipotential surface is a surface with a constant value of electric potential at all points on the surface. The component of electric field parallel to an equipotential surface is zero, as the potential does not change in this direction. Thus, the electric field is perpendicular to the equipotential surface at each point of the surface. Further, say the direction of electric field electrical potential gradually fall with distance.
If a charge of 2 nC is taken from equipotential surface number 2 to 3, what is the amount of work done ?View SolutionThe angle between the equipotential surface and the electric field (or line of force) at any point on the equipotential surface is
View SolutionAssertion(A): Electric field is always normal to equipotential surfaces and along the direction of decreasing order of potential
Reason(R): Negative gradient of electric potential is electric field.View SolutionCan electric field exist longitudinal to an equipotential surface ? Give reason .
View SolutionWhy are electric field lines perpendicular at a point on an equipotential surface of a conductor ?
View SolutionWhy are electric field lines perpendicular at a point on an equipotential surface of a conductor ?
View Solution

