Consider the following statements in respect of histogram.
I. The algebraic sum of deviations of a set of n values from its atithmetic mean is zero.
II In the case of frequency distribution, mode is the value of variable which corresponds to maximam frequency Which of the above statement (s) is/are correct?
Only I
Only II
Both I and II
Neither I nor 2
The correct Answer is:C
To solve the question regarding the correctness of the statements about histograms, we will analyze each statement step by step.
Step 1: Analyze the first statement
Statement I: "The algebraic sum of deviations of a set of n values from its arithmetic mean is zero."
1. Understanding the mean: The arithmetic mean (denoted as ¯x) of a set of n observations x1,x2,...,xn is calculated as:
¯x=∑ni=1xin
2. Calculating the deviations: The deviation of each observation from the mean is given by xi−¯x.
3. Sum of deviations: The algebraic sum of these deviations is:
n∑i=1(xi−¯x)=n∑i=1xi−n∑i=1¯x
Since ¯x is a constant, we can write:
n∑i=1¯x=n⋅¯x
Therefore, the sum of deviations becomes:
n∑i=1xi−n⋅¯x
4. Substituting the mean: From the definition of the mean, we know that n∑i=1xi=n⋅¯x. Thus:
n∑i=1(xi−¯x)=n⋅¯x−n⋅¯x=0
Conclusion for Statement I: The first statement is correct.
Step 2: Analyze the second statement
Statement II: "In the case of frequency distribution, mode is the value of variable which corresponds to maximum frequency."
1. Understanding mode: The mode is defined as the value that appears most frequently in a data set. In a frequency distribution, it is the value (or class interval) that has the highest frequency.
2. Definition confirmation: The statement correctly describes the mode in the context of frequency distributions.
Conclusion for Statement II: The second statement is also correct.
Final Conclusion
Both statements I and II are correct. Therefore, the correct answer is that both statements are true.
Summary of the Solution
- Statement I is correct because the algebraic sum of deviations from the mean is zero.
- Statement II is correct because the mode is defined as the value corresponding to the maximum frequency in a frequency distribution.
Final Answer
Both statements are correct.
---
Statement I: "The algebraic sum of deviations of a set of n values from its arithmetic mean is zero."
¯x=∑ni=1xin
n∑i=1(xi−¯x)=n∑i=1xi−n∑i=1¯x
Since ¯x is a constant, we can write:
n∑i=1¯x=n⋅¯x
Therefore, the sum of deviations becomes:
n∑i=1xi−n⋅¯x
n∑i=1(xi−¯x)=n⋅¯x−n⋅¯x=0
Statement II: "In the case of frequency distribution, mode is the value of variable which corresponds to maximum frequency."
Both statements I and II are correct. Therefore, the correct answer is that both statements are true.
- Statement I is correct because the algebraic sum of deviations from the mean is zero.
- Statement II is correct because the mode is defined as the value corresponding to the maximum frequency in a frequency distribution.
Both statements are correct.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
Consider the following statements:
1. The algebraic sum of the deviations of a set of n value from its arithmetic mean is zero.
2. In the case of frequency distribution, mode is the value of variable which corresponds to maximum frequency.
Which of the statements above given is/are correct?A1 onlyB2 onlyCBoth 1 and 2DNeither 1 nor 2 - Question 2 - Select One
Consider the following statements:
1.The algebraic sum of deviations of a set of values from their arithmetic mean is always zero.
2 Arithmetic mean > median > mode for a symmetric distribution
Which of the above statements are corrects?AA) 1onlyBB) 2onlyCC) Both 1 and 2DD) Neither I nor 2 - Question 3 - Select One
Consider the following statements:
1. The algebraic sum of deviations of a set of values from their arithmetic mean is always zero.
2. Arithmetic mean gt Median gt Mode for a symmetric distribution.
Which of the above statements is/are correct?A1 onlyB2 onlyCBoth 1 and 2DNeither 1 nor 2
The age distribution of 40 children is as follows:
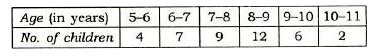
Consider the following statements in respect of the above frequency distribution:
1. The median of the age distribution is 7 years.
2. 70% of the children are in the age group 6-9 years.
3. The modal age of the children is 8 years.
Which of the above statements are correct?View SolutionConsider the following statements in respect of histogram :
1. Histogram is an equivalent graphical representation of the frequency distribution.
2. Histogram is suitable for continuous random variables, where the total frequency of an interval is evenly distributed over the interval.
What of the statements given above is/are correct?View SolutionConsider the following statements in respect of histogram :
1. The histogram is a suitable representation of a frequency distribution of a continuous variable.
2. The area included under the whole histogram is the total frequency.
Which of the above statements is/are correct?View SolutionConsider the following statements:
1. Every composite number is a natural number
2. Every whole number is a natural number
Which of the statement given above is/are correct ?View SolutionConsider the following statement:
1. A continuous random variable can take all values in an interval.
2. A random variable which takes a finite number of values is necessarily discrete.
3. Construction of a frequency distribution is based on data which are discrete.
Which of the above statements are correct ?View Solution

