ABC is a triangle , D, E, F are points in the sides BC, CA, AB respectively dividing them in the ratio 1:4,3:2 and 3:7 respectively. The point P divides AB in the ratio 1:3, then
(→AD+→BE+→CF):→CP =
1:1
2:5
5:2
none
The correct Answer is:B
Topper's Solved these Questions
- ADDITION AND MULTIPLICATION OF VECTORS BOOK - ML KHANNACHAPTER - ADDITION AND MULTIPLICATION OF VECTORS EXERCISE - Problem Set (1) (TRUE AND FALSE )
3 Videos
- ADDITION AND MULTIPLICATION OF VECTORS BOOK - ML KHANNACHAPTER - ADDITION AND MULTIPLICATION OF VECTORS EXERCISE - Problem Set (1) (FILL IN THE BLANKS )
3 Videos
- AREA OF CURVESBOOK - ML KHANNACHAPTER - AREA OF CURVESEXERCISE - SELF ASSESSEMENT TEST
16 Videos
Similar Questions
The points D,E,F divide BC,CAandAB of the triangle ABC in the ratio 1:4,3:2and3:7 respectively and the point divides AB in the ratio 1:3, then (¯¯¯¯¯¯AD+¯¯¯¯¯¯BE+¯¯¯¯¯¯CF):¯¯¯¯¯¯CK is equal to
View SolutionPoints L, M, N divide the sides BC, CA, AB of ABC in the ratio 1:4, 3:2, 3:7 respectively. Prove thatAL + BM + CŃ is a vector parallel to CK where K divides AB in the ratio 1: 3.
View Solution
Knowledge Check
In a triangle ABC, D, E, F are the mid-points of the sides BC, CA and AB respectively , the vector →AD is equal to
A→BE+→CFB→BE−→CFC→CF−→BED−→BE−→CFABC is a triangle and D,E,F are the mid-points of the sides BC,CA, AB respectively . The ratio of the areas of ΔABCandΔDEF is :
A4:1B5:1C3:1Dcan't be determindABC is a triangle and D,E,F are the mid-points of the sides BC,CA, AB respectively . The ratio of the areas of ΔABCandΔDEF is :
A4:1B5:1C3:1Dcan't be determined
Points L, M, N divide the sides BC, CA, AB of ABC in the ratio 1:4, 3:2, 3:7 respectively. Prove thatAL + BM + CŃ is a vector parallel to CK where K divides AB in the ratio 1: 3.
View SolutionABC is a triangle in which D, E and F are the mid-points of the sides AC, BC and AB respectively. What is the ratio of the area of the shaded to the unshaded region in the triangle?
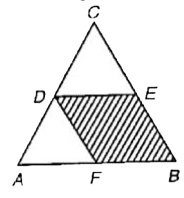
View SolutionIn △ABC, if the points P, Q, R divides the sides BC, CA, AB in the ratio 1:4, 3:2, 3:7 respectively and the point S divides side AB in the ratio 1:3, then (¯¯¯¯¯¯AP+¯¯¯¯¯¯BQ+¯¯¯¯¯¯CR):(¯¯¯¯¯CS)=
View SolutionIn a ΔABC,D,E,F are the mid -points of the sides BC, CA and AB respectively, the vector AD is equal to
View SolutionIn ΔABC,L,M,N are points on BC,CA,AB respectively, dividing them in the ratio 1:2,2:3,3:5, if the point K divides AB in the ratio 5:3, then ∣∣¯¯¯¯¯¯AL+¯¯¯¯¯¯¯BM+¯¯¯¯¯¯CN∣∣∣∣¯¯¯¯¯¯CK∣∣=
View Solution
