The number of geometrical isomers possible in triamminetrinitrocobalt (III) is X and in trioxalatohromate (III) is Y. Then the value of X + Y is _________ .
The correct Answer is:2
To solve the problem, we need to determine the number of geometrical isomers for two complexes: triamminetrinitrocobalt(III) and trioxalatohromate(III).
1. Identify the Complexes:
- The first complex is triamminetrinitrocobalt(III), which can be represented as (Co(NH₃)₃(NO₃)₃).
- The second complex is trioxalatohromate(III), represented as (Cr(C₂O₄)₃).
2. Determine the Number of Geometrical Isomers for (Co(NH₃)₃(NO₃)₃):
- In this complex, cobalt is surrounded by three ammonia (NH₃) ligands and three nitrate (NO₃) ligands.
- The ligands can arrange themselves in two ways:
- Cis: where similar ligands (NH₃) are adjacent to each other.
- Trans: where similar ligands are opposite each other.
- For this complex, we can have two geometrical isomers:
- Cis isomer: All three NH₃ ligands are on one side, and all three NO₃ ligands are on the opposite side.
- Trans isomer: The NH₃ ligands are arranged such that one NH₃ is opposite to another NH₃, and similarly for the NO₃ ligands.
- Therefore, the number of geometrical isomers (X) for (Co(NH₃)₃(NO₃)₃) is 2.
3. Determine the Number of Geometrical Isomers for (Cr(C₂O₄)₃):
- In this complex, chromium is surrounded by three bidentate oxalate (C₂O₄) ligands.
- Since oxalate is a bidentate ligand, it binds through two coordination sites, creating a situation where the arrangement does not allow for cis or trans isomers.
- Bidentate ligands do not produce geometrical isomers because they occupy two positions at once, leading to a symmetrical arrangement.
- Therefore, the number of geometrical isomers (Y) for (Cr(C₂O₄)₃) is 0.
4. Calculate X + Y:
- Now, we can sum the values of X and Y:
- X = 2 (from (Co(NH₃)₃(NO₃)₃))
- Y = 0 (from (Cr(C₂O₄)₃))
- Therefore, X + Y = 2 + 0 = 2.
Final Answer:
The value of X + Y is 2.
- The first complex is triamminetrinitrocobalt(III), which can be represented as (Co(NH₃)₃(NO₃)₃).
- The second complex is trioxalatohromate(III), represented as (Cr(C₂O₄)₃).
- In this complex, cobalt is surrounded by three ammonia (NH₃) ligands and three nitrate (NO₃) ligands.
- The ligands can arrange themselves in two ways:
- Cis: where similar ligands (NH₃) are adjacent to each other.
- Trans: where similar ligands are opposite each other.
- For this complex, we can have two geometrical isomers:
- Cis isomer: All three NH₃ ligands are on one side, and all three NO₃ ligands are on the opposite side.
- Trans isomer: The NH₃ ligands are arranged such that one NH₃ is opposite to another NH₃, and similarly for the NO₃ ligands.
- Therefore, the number of geometrical isomers (X) for (Co(NH₃)₃(NO₃)₃) is 2.
- In this complex, chromium is surrounded by three bidentate oxalate (C₂O₄) ligands.
- Since oxalate is a bidentate ligand, it binds through two coordination sites, creating a situation where the arrangement does not allow for cis or trans isomers.
- Bidentate ligands do not produce geometrical isomers because they occupy two positions at once, leading to a symmetrical arrangement.
- Therefore, the number of geometrical isomers (Y) for (Cr(C₂O₄)₃) is 0.
- Now, we can sum the values of X and Y:
- X = 2 (from (Co(NH₃)₃(NO₃)₃))
- Y = 0 (from (Cr(C₂O₄)₃))
- Therefore, X + Y = 2 + 0 = 2.
The value of X + Y is 2.
Topper's Solved these Questions
- JEE MAINS 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2021EXERCISE - Chemistry Section A
40 Videos
- JEE MAINS 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2021EXERCISE - Chemistry Section B
20 Videos
- JEE MAINS 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2021EXERCISE - CHEMISTRY (SECTION-A)
80 Videos
- JEE MAINS 2020BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2020EXERCISE - CHEMSITRY
25 Videos
- JEE MAINS 2022BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2022EXERCISE - CHEMISTRY (SECTION-B)
10 Videos
Similar Questions
Knowledge Check
Number of geometrical isomers possible for[MABCDEF] are
A6B10C15D12How many geometrical isomers are possible for
 A2B3C4D8
A2B3C4D8
How many geometrical isomers are possible for
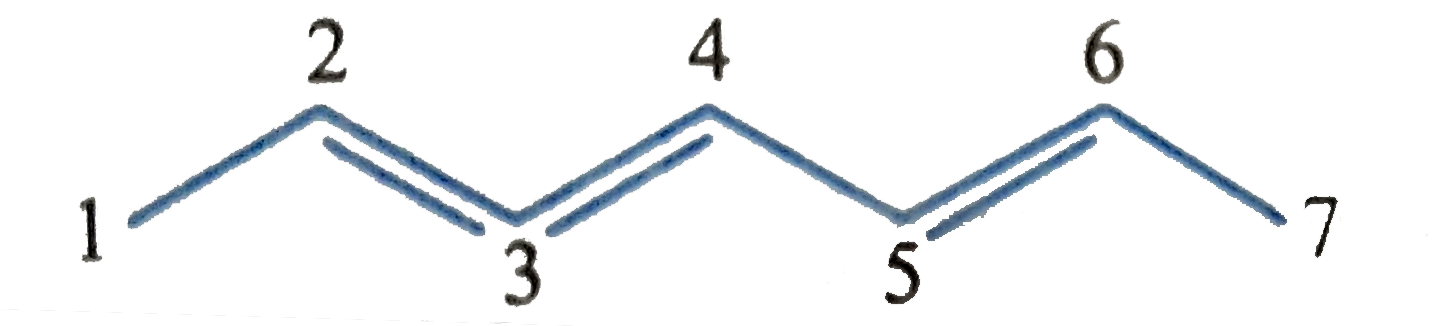
View SolutionHow many geometrical isomers are possible for
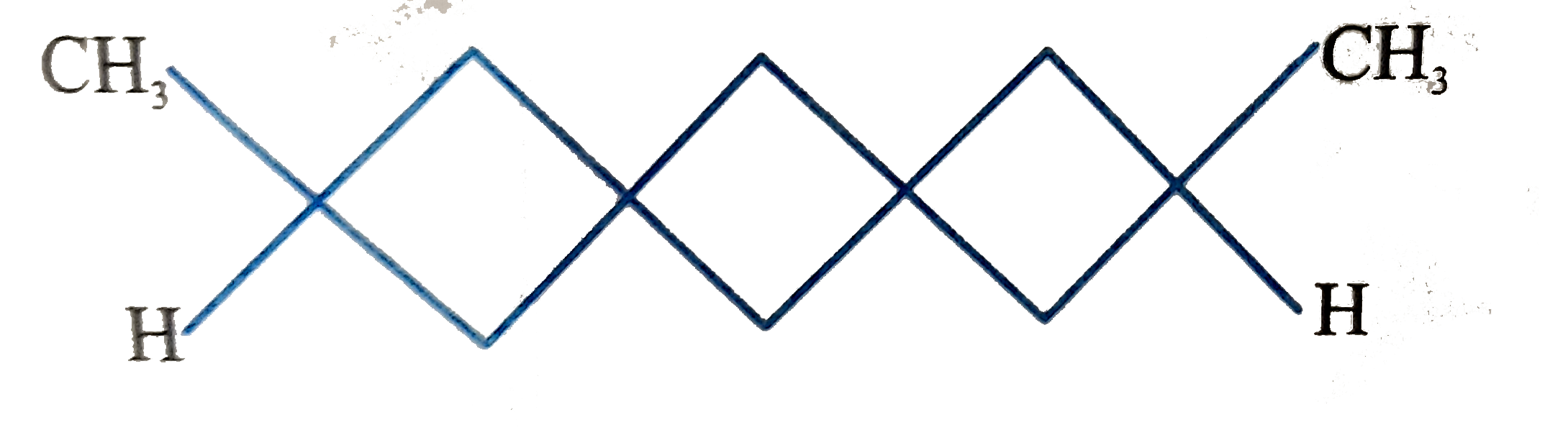
View SolutionThe total number of geometrical isomers possible in the following compound is
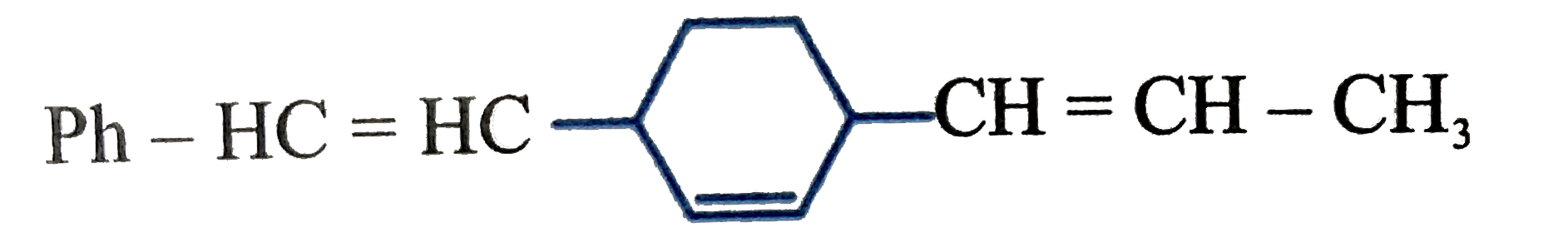
View Solution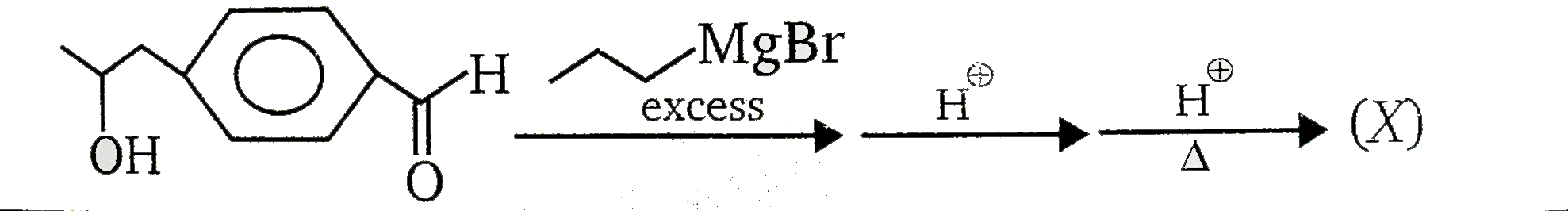
How many geometrical isomer of (X) is possible ?
View Solution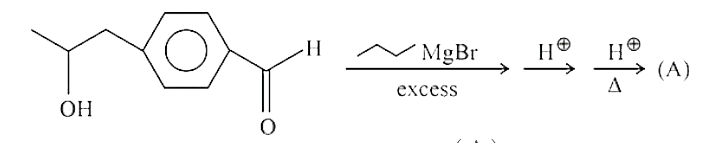
How many geometrical isomer of (A) is possible?
View SolutionThe total number of geometrical isomers are possible for the following compound is

View Solution