Question

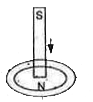
A metallic ring is held horizontal and a magnet is allowed to fall vertically through it with N-pole pointing upwards. The acceleration of magnet near the ring is a. Then-

Options

A. a = g
B. a `lt` g while approaching but a `gt` g while receding
C. `a lt g` while approaching as well as receding
D. `a gt g` while approaching but `a lt g` while receding

Answer

c

Explanation

To solve the problem of a metallic ring and a falling magnet, we can follow these steps:

Step 1: Understand the Setup
A metallic ring is held horizontally, and a magnet with its North Pole pointing upwards is allowed to fall through it.

Step 2: Apply Faraday's Law of Electromagnetic Induction
As the magnet falls, it creates a changing magnetic flux through the ring. According to Faraday's law, a changing magnetic flux induces an electromotive force (EMF) in the ring.

Step 3: Use Lenz's Law
Lenz's law states that the direction of the induced EMF will be such that it opposes the change in magnetic flux that produced it. In this case, as the North Pole of the magnet approaches the ring, the induced current in the ring will create a magnetic field that opposes the magnet's motion.

Step 4: Analyze the Motion of the Magnet
- When the magnet is approaching the ring: The induced current in the ring will create a magnetic field that opposes the approach of the magnet. This means that the effective acceleration of the magnet will be less than the gravitational acceleration (g).

- When the magnet is moving away from the ring: After the magnet passes through the ring, the induced current will now attract the magnet back towards the ring, again reducing its acceleration.

Step 5: Conclusion
In both cases (approaching and receding), the acceleration of the magnet will be less than the gravitational acceleration (g). Therefore, we conclude that the acceleration of the magnet near the ring is less than g.

Final Answer
The acceleration of the magnet near the ring is \( a < g \).

---