A light ray is incident on a plane mirror making an angle of 45∘ with respect to the surface of the mirror. The value of the angle between incident ray and reflected ray is ____∘
To solve the problem, we need to determine the angle between the incident ray and the reflected ray when a light ray strikes a plane mirror at an angle of 45 degrees with respect to the surface of the mirror.
1. Understanding the Angle of Incidence:
- The angle of incidence (I) is the angle between the incident ray and the normal (an imaginary line perpendicular to the surface of the mirror).
- Since the light ray makes an angle of 45 degrees with respect to the surface of the mirror, we can find the angle of incidence using the relationship:
Angle of incidence (I)=90∘−45∘=45∘
2. Applying the Law of Reflection:
- According to the law of reflection, the angle of incidence is equal to the angle of reflection (R). Therefore:
Angle of reflection (R)=Angle of incidence (I)=45∘
3. Calculating the Angle Between the Incident Ray and the Reflected Ray:
- The angle between the incident ray and the reflected ray is the sum of the angle of incidence and the angle of reflection:
Angle between incident ray and reflected ray=I+R=45∘+45∘=90∘
4. Final Answer:
- Thus, the angle between the incident ray and the reflected ray is:
Angle=90∘
Final Answer:
The value of the angle between the incident ray and the reflected ray is 90 degrees.
---
- The angle of incidence (I) is the angle between the incident ray and the normal (an imaginary line perpendicular to the surface of the mirror).
- Since the light ray makes an angle of 45 degrees with respect to the surface of the mirror, we can find the angle of incidence using the relationship:
Angle of incidence (I)=90∘−45∘=45∘
- According to the law of reflection, the angle of incidence is equal to the angle of reflection (R). Therefore:
Angle of reflection (R)=Angle of incidence (I)=45∘
- The angle between the incident ray and the reflected ray is the sum of the angle of incidence and the angle of reflection:
Angle between incident ray and reflected ray=I+R=45∘+45∘=90∘
- Thus, the angle between the incident ray and the reflected ray is:
Angle=90∘
The value of the angle between the incident ray and the reflected ray is 90 degrees.
Topper's Solved these Questions
- ELECTRICITY BOOK - BEIITIANSCHAPTER - ELECTRICITY EXERCISE - IIT JEE Worksheet V. Matrix Matching
2 Videos
- ELECTRICITY BOOK - BEIITIANSCHAPTER - ELECTRICITY EXERCISE - IIT JEE Worksheet III. Paragraph Type
2 Videos
- ENERGY AND WORK BOOK - BEIITIANSCHAPTER - ENERGY AND WORK EXERCISE - IIT JEE WORKSHEET
34 Videos
Similar Questions
A light ray is incident on a plane mirror making an angle of 90∘ with the mirror's surface. The angle of reflection of the light is _______ .
View SolutionA light ray is incident on a plane mirror making an angle of 90∘ with the mirror's surface. The angle of reflection of the light is _______ .
View Solution
Knowledge Check
A light ray is incident normally on a plane mirror. The angle of reflection will be
A120∘B90∘C45∘D0∘plane mirror makes an angle of 30∘ with horizontal. If a vertical ray strikes the mirror, find the angle between mirror and reflected ray
A30∘B45∘C60∘D90∘An incident ray strikes a plane mirror at an angle of 15∘ with the mirror. The angle between the incident ray and reflected ray is
A15∘B30∘C150∘Dnone of these
A ray of light is incident on a plane mirror at an angle of 40∘ with respect to surface normal. When it gets reflected from the mirror, it undergoes a deviation of
View SolutionIf a light ray incident on a plane mirror makes an angle 30∘ with it, find the angle of incidence and angle of reflection.
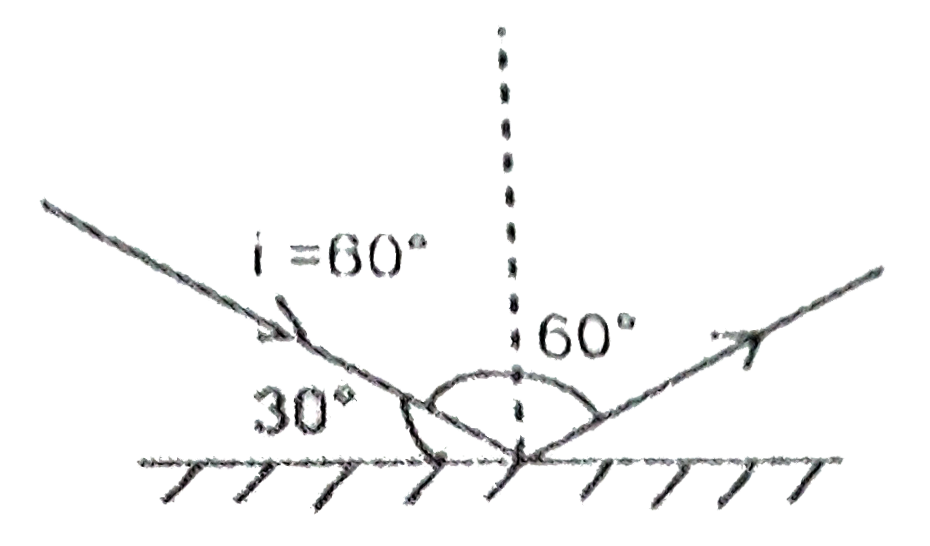
View SolutionIf a ray of light incident on a plane mirror is such that it makes an angle of 30∘ with the mirror, then the angle made by the reflected ray with the mirror is
View SolutionA ray of light is incident on a plane mirror, making an angle 'q' with the surface of the mirror. Arrange the following steps in sequential order to calculate the angle between the incident light ray and the reflected light ray.
(A) Draw a normal line on the plane mirror at the point of incidence of the light ray.
(B) Note down the angle between the plane mirror and the incident light ray.
(C) The sum of the angle of incidence and the angle between the plane mirror and the incident ray is 90∘.Determine the angle of incidence.
(D) From the laws of reflection, the angle of incidence is equal to the angle of reflection. Then the sum of the angle of incidence and the angle of reflection gives the angle between the incident light ray and the reflected light ray.
View SolutionA ray of light is incident on a plane mirror, making an angle 'q' with the surface of the mirror. Arrange the following steps in sequential order to calculate the angle between the incident light ray and the reflected light ray.
(A) Draw a normal line on the plane mirror at the point of incidence of the light ray.
(B) Note down the angle between the plane mirror and the incident light ray.
(C) The sum of the angle of incidence and the angle between the plane mirror and the incident ray is 90∘.Determine the angle of incidence.
(D) From the laws of reflection, the angle of incidence is equal to the angle of reflection. Then the sum of the angle of incidence and the angle of reflection gives the angle between the incident light ray and the reflected light ray.
View Solution
