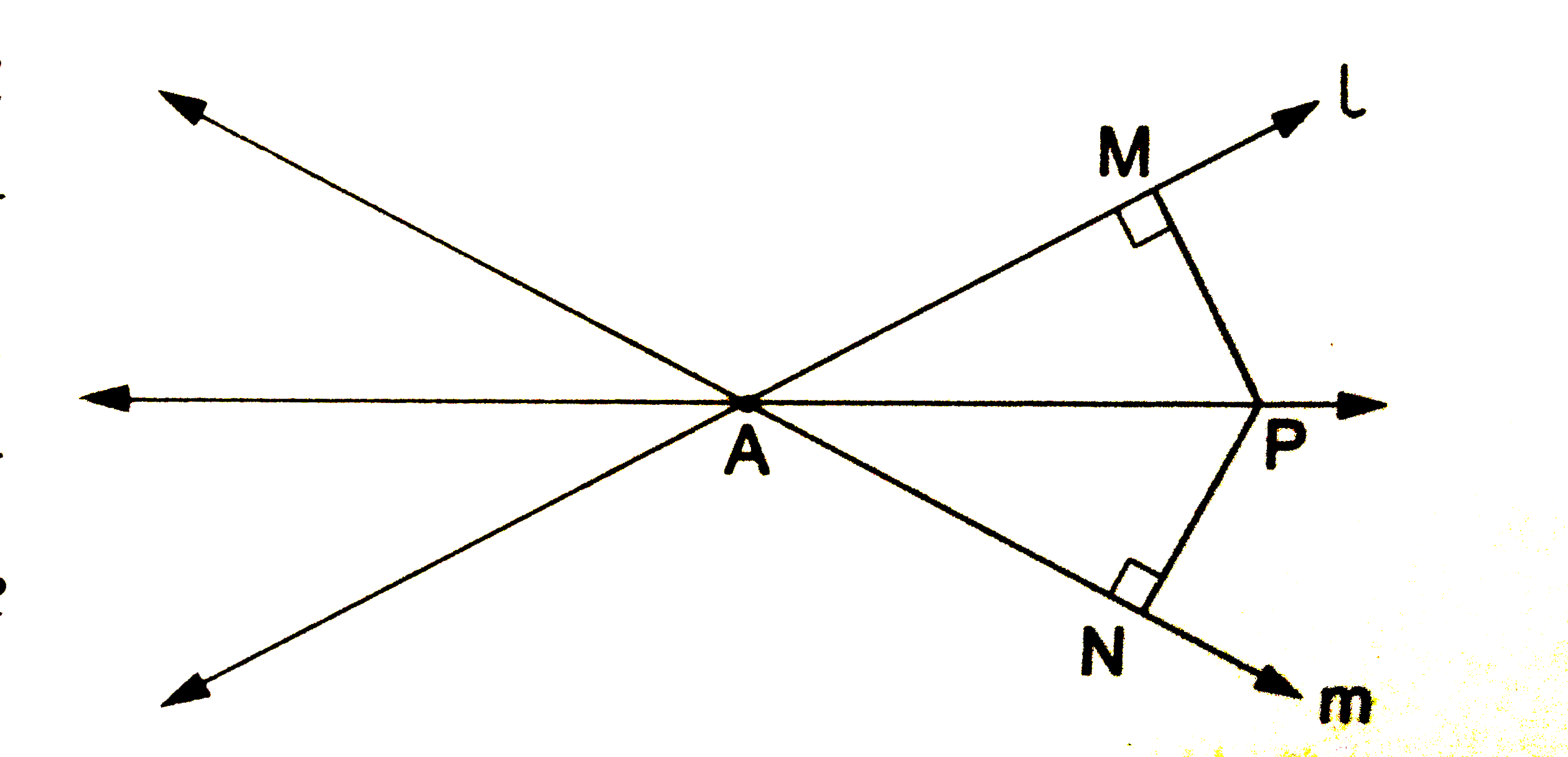
In figure P is a point equidistant from the lines l and m intersecting at point A. Show that the line n (along AP) bisects the angle between l and m.
In figure P is a point equidistant from the lines l and m intersecting at point A. Show that the line n (along AP) bisects the angle between l and m.

Video Solution
Doubtnut Promotions Banner
|
Answer

Step by step video & image solution for In figure P is a point equidistant from the lines l and m intersecting at point A. Show that the line n (along AP) bisects the angle between l and m. by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.

Updated on:21/07/2023
Class 9MATHSTRIANGLES

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • The loucs of a point which is a equidistant from two non-intersecting lines l and m is a _____

    Astraight line parallel to the line l
    BStraight line parallel to the line m
    CStraight line parallel to the line l and m and midway between them
    Dstraight line that intersects both the line l and m