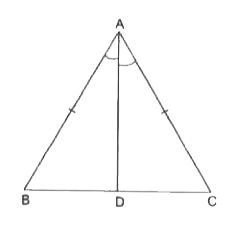
If two isosceles triangles have a common base, prove that the line segment joining their vertices bisects the common base at right angles.
Step by step video & image solution for If two isosceles triangles have a common base, prove that the line segment joining their vertices bisects the common base at right angles. by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
- TRIANGLESBook:MTG IIT JEE FOUNDATIONChapter:TRIANGLESExercise:EXERCISE (Subjective Problems) ( Integer/Numerical Value Type)
Explore 5 Videos
- TRIANGLESBook:MTG IIT JEE FOUNDATIONChapter:TRIANGLESExercise:OLYMPAID/ HOTS CORNER
Explore 10 Videos
- TRIANGLESBook:MTG IIT JEE FOUNDATIONChapter:TRIANGLESExercise:EXERCISE (Subjective Problems) ( Short Answer Type)
Explore 10 Videos
- STATISTICSBook:MTG IIT JEE FOUNDATIONChapter:STATISTICSExercise:Olympiad/HOTS Corner
Explore 20 Videos
Similar Questions
If two isosceles triangles have a common base, prove that the line joining their vertices bisects them at right angles.
View SolutionWhich two triangles have ∠B as common?

View SolutionProve that the bisector of the vertical angle of an isosceles triangle bisects the base at right angles.
View SolutionAxis the line segment joining the centres of two bases is called the axis of the cylinder.
View SolutionShow that the bisector of vertical angle of an isosceles triangle bisects the base at right angles.
View SolutionThe equation of the line bisecting the line segment joining the points (a,b) and (a',b') at right angle, is
View SolutionIf in an isosceles triangle, each of the base angles is 40°, then the triangle is
View SolutionFind the equation of the plane which bisects the line segment joining the points A(2,3,4)andB(4,5,8) at right angles.
View SolutionThe altitude from the vertex bisect the base in an isosceles triangle
View SolutionA square is incribed in an isoceles right triangle, so that the square and the triangle have one angle common. Show that the vertex of the sqare opposite the vertex of the common angle bisects the hypotenuse.
View Solution