Find the area of the given triangle.
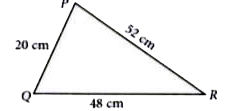
The correct Answer is:480cm2
Step by step video, text & image solution for Find the area of the given triangle. by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
- FOOTSTEPS TOWARDS CBSE BOARDBOOK - MTG IIT JEE FOUNDATIONCHAPTER - FOOTSTEPS TOWARDS CBSE BOARDEXERCISE - PART-B (SECTION-IV)
4 Videos
- FOOTSTEPS TOWARDS CBSE BOARDBOOK - MTG IIT JEE FOUNDATIONCHAPTER - FOOTSTEPS TOWARDS CBSE BOARDEXERCISE - PART-A (SECTION-II)
16 Videos
- FOOTSTEPS TOWARDS (JEE MAIN)BOOK - MTG IIT JEE FOUNDATIONCHAPTER - FOOTSTEPS TOWARDS (JEE MAIN)EXERCISE - Section B (Numerical Value Type Questions)
10 Videos
- HERON'S FORMULABOOK - MTG IIT JEE FOUNDATIONCHAPTER - HERON'S FORMULAEXERCISE - OLYMPIAD/HOTS CORNER
7 Videos
Similar Questions
Find the area of the triangle formed by joining the mid–points of the sides of the triangle whose vertices are (0, –1), (2, 1) and (0, 3). Find the ratio of this area to the area of the given triangle.
View SolutionFind the area of the triangle formed by joining the mid-points of the sides of the triangle, whose vertices are (2, - 4), (6, 2) and (- 4, 6). Find the ratio of this area to the area of the given triangle.
View Solution
Knowledge Check
The sides of a triangle are 21, 20 and 13 cm. Find the area of the larger triangle into which the given triangle is divided by the perpendicular upon the longest side from the opposite vertex.
A72cm2B96cm2C168cm2D144cm2Find the area of the triangle whose vertices are given below (−3,−4),(−2,−7),(−1,−9)
A2sq.unitsB1sq.unitsC12sq.unitsD14sq.units
Find the area of the triangle formed by joining the mid-points of the sides of the triangle whose vertices are (0,−1),(2,1)and(0,3). Find the ratio of this area to the area of the given triangle.
View SolutionIf the two legs of a right-angled triangle are 3cm and 4cm,find the area of the triangle. Find the length of the base of a triangle
View SolutionCorresponding sides of two similar triangles are in the ratio 1:3 . If the area of the smaller triangle in 40 cm2 , find the area of the larger triangle.
View SolutionThe corresonding sides of two similar triangle are in the ratio 2:3. If the area of the smaller trianle is 48cm2, find the area of the larger triangle.
View SolutionLet Δ be the area of a triangle. Find the area of a triangle whose each side is twice the side of the given triangle.
View SolutionVertices BandC of ABC lie along the line x+22=y−11=z−04 . Find the area of the triangle given that A has coordinates (1,−1,2) and line segment BC has length 5.
View Solution
