A cricket ball of mass 500 g is moving with speed of 36kmh−1 . It is reflected back with the same speed . The impulse (in kg ms−1 ) applied on it is ______
The correct Answer is:10
To solve the problem, we need to calculate the impulse applied to a cricket ball of mass 500 g that is moving at a speed of 36 km/h and is reflected back with the same speed.
1. Convert Mass from grams to kilograms:
Mass (m)=500g=5001000kg=0.5kg
2. Convert Speed from km/h to m/s:
Speed (u)=36km/h=36×518m/s=10m/s
3. Determine Initial and Final Velocities:
- Initial velocity (u) = +10 m/s (moving in the positive direction)
- Final velocity (v) = -10 m/s (reflected back in the opposite direction)
4. Calculate Change in Momentum:
- Initial momentum (P_i) = m × u = 0.5 kg × 10 m/s = 5 kg·m/s
- Final momentum (P_f) = m × v = 0.5 kg × (-10 m/s) = -5 kg·m/s
- Change in momentum (ΔP) = P_f - P_i = -5 kg·m/s - 5 kg·m/s = -10 kg·m/s
5. Calculate Impulse:
- Impulse (I) is equal to the change in momentum:
I=ΔP=−10kg⋅m/s
Final Answer:
The impulse applied on the cricket ball is -10 kg·m/s.
---
Mass (m)=500g=5001000kg=0.5kg
Speed (u)=36km/h=36×518m/s=10m/s
- Initial velocity (u) = +10 m/s (moving in the positive direction)
- Final velocity (v) = -10 m/s (reflected back in the opposite direction)
- Initial momentum (P_i) = m × u = 0.5 kg × 10 m/s = 5 kg·m/s
- Final momentum (P_f) = m × v = 0.5 kg × (-10 m/s) = -5 kg·m/s
- Change in momentum (ΔP) = P_f - P_i = -5 kg·m/s - 5 kg·m/s = -10 kg·m/s
- Impulse (I) is equal to the change in momentum:
I=ΔP=−10kg⋅m/s
The impulse applied on the cricket ball is -10 kg·m/s.
Topper's Solved these Questions
Similar Questions
A body is moving with a speed of 36 kmph. Its speed=____m/s.
View SolutionAn empty truck of mass 1000 kg is moving at a speed of 36 km h−1. It is loaded with 500 kg material on its way and again moves with the same speed. Will the momentum of the truck remain the same after loading? If not, find the momentum of the truck after loaded.
View Solution
Knowledge Check
A cricket ball of 0.5kg moving with a velocity of 100ms−1The wavelength associtated with in motion is
A1/100cmB66×10−34mC1.32×10−35mD6.6×10−−26mA cricket ball of mass 150 g is moving with a speed of 12ms−1 and is hit by a bat so that the ball is turned back with velocity of 20ms−1 if duration of contact between bat and ball is 0.01 s then:
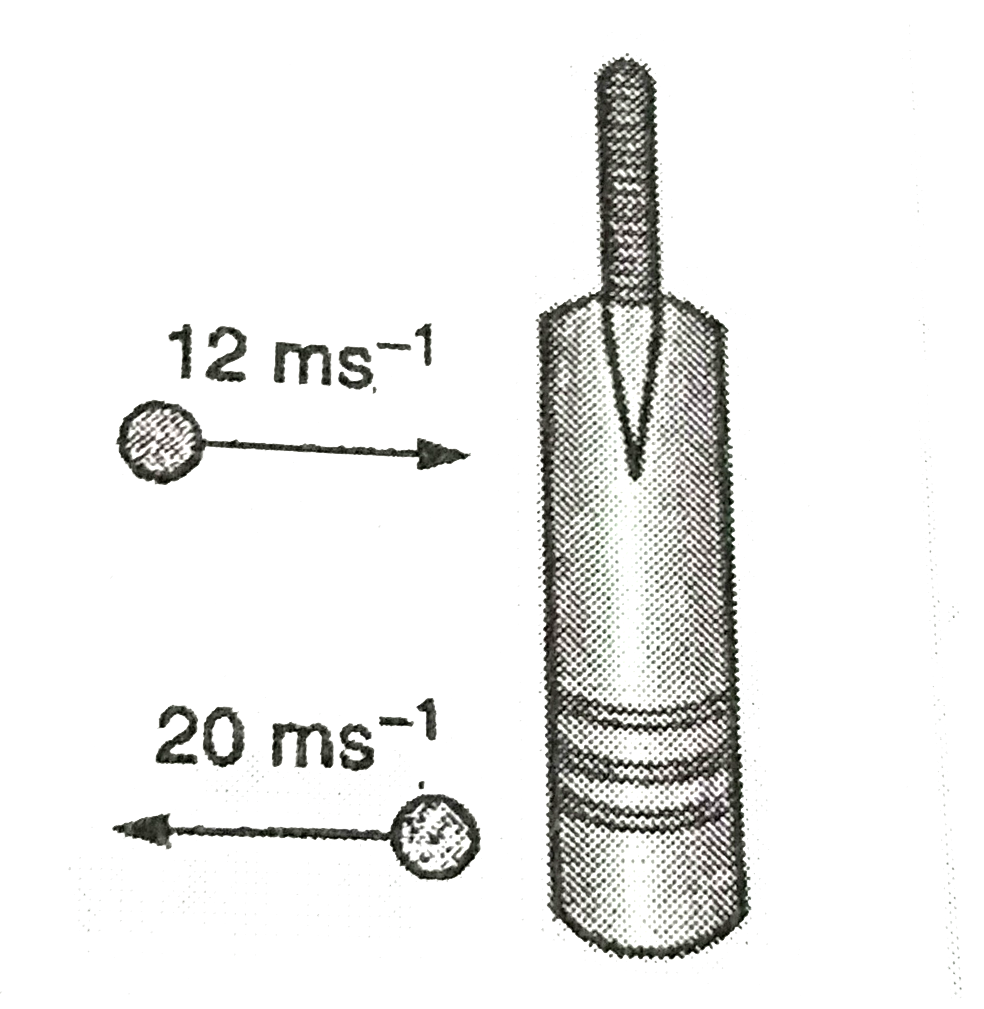 Aimpulse of force is 4.8 NsBaverage force exerted by bat is 480 NCretarding of the ball is 3200ms−2Dchange in momentum of the ball is 1.2kgms−1
Aimpulse of force is 4.8 NsBaverage force exerted by bat is 480 NCretarding of the ball is 3200ms−2Dchange in momentum of the ball is 1.2kgms−1Two billiard ball A and B each of mass 50g and moving in opposite direction with speed of 5ms−1 each, collide and rebound with the same speed. The impulse imparted to each ball is
A0.25kgms−1B0.5kgms−1C0.8kgms−1D0.125kgms−1
Convert a speed of 36km/h into m/s.
View SolutionA bullet of mass 50g moving with a speed of 500ms−1 is brought to rest in 0.01s Find the impulse and the average force .
View SolutionA ball of mass 150 g moving with an acceleration 20 m/s2 is hit by a force, which acts on it for 0.1 s. The impulsive force is
View SolutionA ball of mass 'm' strikes a rigid wall with speed 'u' and rebounds with the same speed. The impulse imparted to the ball by the wall is
View SolutionA ball of mass m strikes a rigid walJ with speed u and rebounds with the same speed. The impulse imparted to the ball by the wall is
View Solution
