A load suspended from a spring while its other end is on a hook fixed to a wall.
Step-by-Step Solution:
1. Identify the Components:
- In this scenario, we have two main components: a load and a spring that is fixed to a wall via a hook.
2. Understand the Setup:
- The load is suspended from the spring, meaning the load is hanging down due to gravity. The other end of the spring is attached to a hook on the wall.
3. Identify the Forces:
- The load exerts a downward force on the spring due to its weight (force of gravity). This force can be represented as F=mg, where m is the mass of the load and g is the acceleration due to gravity.
4. Effect of the Force on the Spring:
- The downward force applied by the load causes the spring to stretch. This is a result of the spring's elasticity. According to Hooke's Law, the force exerted by the spring is proportional to the amount it is stretched (or compressed), which can be expressed as Fs=kx, where k is the spring constant and x is the displacement from its original position.
5. Conclusion:
- The load applies a force on the spring, causing it to change shape (stretch). The spring, in turn, exerts an equal and opposite force back on the load, which is why the load remains suspended.
Final Answer:
- The load applies a downward force on the spring, resulting in a change in the shape of the spring (stretching).
---
- In this scenario, we have two main components: a load and a spring that is fixed to a wall via a hook.
- The load is suspended from the spring, meaning the load is hanging down due to gravity. The other end of the spring is attached to a hook on the wall.
- The load exerts a downward force on the spring due to its weight (force of gravity). This force can be represented as F=mg, where m is the mass of the load and g is the acceleration due to gravity.
- The downward force applied by the load causes the spring to stretch. This is a result of the spring's elasticity. According to Hooke's Law, the force exerted by the spring is proportional to the amount it is stretched (or compressed), which can be expressed as Fs=kx, where k is the spring constant and x is the displacement from its original position.
- The load applies a force on the spring, causing it to change shape (stretch). The spring, in turn, exerts an equal and opposite force back on the load, which is why the load remains suspended.
- The load applies a downward force on the spring, resulting in a change in the shape of the spring (stretching).
Topper's Solved these Questions
- FORCE AND PRESSURE BOOK - MTG IIT JEE FOUNDATIONCHAPTER - FORCE AND PRESSURE EXERCISE - EXERCISE (MULTIPLE CHOICE QUESTIONS LEVEL-I)
30 Videos
- FORCE AND PRESSURE BOOK - MTG IIT JEE FOUNDATIONCHAPTER - FORCE AND PRESSURE EXERCISE - EXERCISE (MULTIPLE CHOICE QUESTIONS LEVEL-2)
22 Videos
- FORCE AND PRESSURE BOOK - MTG IIT JEE FOUNDATIONCHAPTER - FORCE AND PRESSURE EXERCISE - SOLVED EXAMPLES
14 Videos
- FOOTSTEPS TOWARDS NEETBOOK - MTG IIT JEE FOUNDATIONCHAPTER - FOOTSTEPS TOWARDS NEETEXERCISE - MCQ
45 Videos
- FRICTION BOOK - MTG IIT JEE FOUNDATIONCHAPTER - FRICTION EXERCISE - OLYMPIAD/HOTS CORNER
15 Videos
Similar Questions
In the following situations identify the agent exerting the force and the object on which it acts. State the effect of the force in each case
A load suspended from spring while its other end is on a hook fixed to a wall
View SolutionA straight rod of light L and mass m is pivoted freely from a point of the roof. Its lower end is connected to an ideal spring of spring constant K. The other end of the spring is connected to the wall as shown in the figure. Find the frequency of small oscillation of the system.
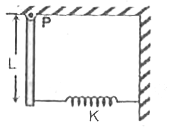
View Solution
Knowledge Check
Figure shows a magnet suspended at the lower end of a spring while its length lies along the axis of a fixed circular conducting coil. The magnet is made to oscillate,
Deflection in the galvanometer is
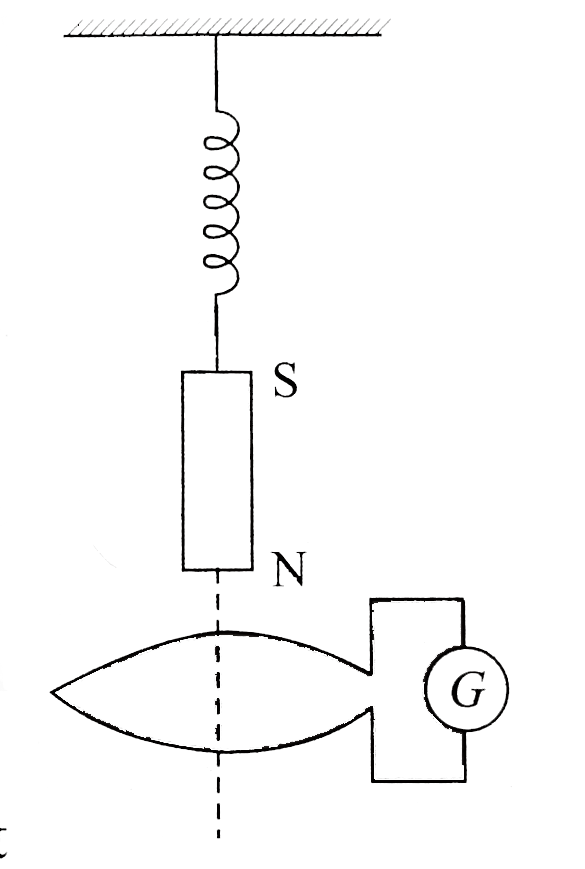 Aminimum when the magnet is at mean positionBMaximum when the magnet is at mean positionCzero when the mgnet is at mean positionDmaximum when the magnet is at exteme position
Aminimum when the magnet is at mean positionBMaximum when the magnet is at mean positionCzero when the mgnet is at mean positionDmaximum when the magnet is at exteme positionThe time period of a mass suspended from a spring is T if the spring is cut in to equal part and the same mass is suspended from one of the pert then the time period will be
ATBT2C2TDT4A metal ball suspended from the hook of a spring balance is kept immesed in a liquid other than water. On increassing the temperature of this liquid, the reading in the spring balance.
AincreasesBDecreasesCRemains sameDMay increase or decreases.
When a mass M is suspended from a spring, its period of oscillation is 2 second. If a mass 4 M is suspended from the same spring, then its period of oscillation will be
View SolutionWhen a load of 80 N is suspended from a string, its length is 101 mm. If a load of 100 N is suspended, its length is 102 mm. If a load of 160 N is suspended form it, then the length of the string is (Assume the area of cross-section unchanged)
View SolutionA body is attached to a spring whose other end is fixed. If the spring is elongated by x, its potential energy is U=5x2, where x is in metre and U is in joule. U-x graph is
View SolutionAn object weights W newton on earth. It is suspended from the lower end of a spring balance whose upper end is fixed to the ceiling of a space capsule in a stable orbit around the earth. The reading of the spring balance will be
View SolutionA block of mass m is attached to one end of a mass less spring of spring constant k. the other end of spring is fixed to a wall the block can move on a horizontal rough surface. The coefficient of friction between the block and the surface is μ then the compession of the spring for which maximum extension of the spring becomes half of maximum compression is .
View Solution
