Derive electric field intensity due to uniformly charged ring or circular loop.
Text Solution
Consider a uniformly charged thin ring of radius a. Let λ be the linear charge density of the ring.
Let P be the point on the axis of the ring at a distance r from the centr of the ring.
Consider a small element of length dl on the ring, distant CP=√r2+a2 from point P.
Charge on the element.
dq=λdl
∴ the electric field intensity due to this small element at point P is,
dE=14πɛ0dqCP2
Since. dq=λdl
∴ dE=14πɛ0λdlCP2
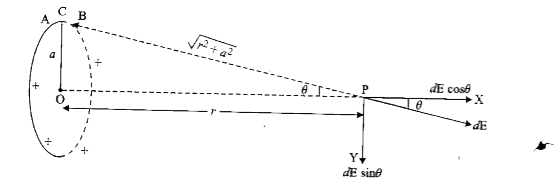
In right angled ΔAOP,CP2=r2+a2
∴ dE=14πɛ0λdl(r2+a2) ...(1)
where dE makes an angle θ with the axis of the ring.
Resolve dE into two component
(i) dEcosθ parallel to the axis of the ring.
(ii) dEsinθ perpendicular to the axis of ring.
Horizontal component dEcosθ are added up because they are in the same direction. dEsinθ component of electric field is cancelled out as they are equal and opposite in direction.
∴ the net electric field intensity is
E=∑dEcosθ=∫whole ringdEcosθ
∴ from equation (1)
E=∫whole ring14πɛ0λdl(r2+a2)cosθ ...(2)
From ΔAOP
cosθ=rCP=r(r2+a2)1/2
from eq., (2)
E=∫wholeringdl=circumference of ring=2=πa
E=λr4πɛ0(r2+a2)3/2×2πa
=(λ2πa)r4πɛ0(r2+a2)3/2
We know that,λ(2πa)=q
E=qr4πɛ0(r2+a2)3/2
Direction of electric field intensity due to ring is along the axis the of ring.
(i) When x >>>> a then r2+a2≈r2
∴ E=14πɛ0qr(r2)3/2
E=14πɛ0qrr3
E=14πɛ0qrr2along OP
(ii) When x=0 (i.e., P lies at the center of the ring)
∴ E=14πɛ00r2=0
There is no electric field at the centre of ring.
|
Updated on:21/07/2023
Similar Questions
Knowledge Check
- Question 1 - Select One
The maximum electric field intensity on the axis of a uniformly charged ring of charge q and radius R will be
A14πε0q3√3R2B14πε02q3R2C14πε02q3√3R2D14πε03q2√2R2 - Question 2 - Select One
The electric field due to a uniformly charged sphere is maximum at :
Acentre of sphereBinside the surfaceCat the surfaceDat infinite distance from the centre - Question 3 - Select One
Intensity of electric field inside a uniformly charged hollow sphere is
AzeroBnon zero constantCchange with rDinversely proportional to r
The maximum electric field intensity on the axis of a uniformly charged ring of charge q and radius R is at a distance x from the centre of the ring.The value of x is
View SolutionDerive an expression for electric intensity E due to a uniformly charged thin spherical shell at a point outside the shell.
View SolutionDerive an expression for the strength of electric field intensity at a point on the axis of a uniformly charged circular coil of radius R carrying charge Q.
View SolutionFind the electric field due to an uniformly charged arc, at the center 'c'.
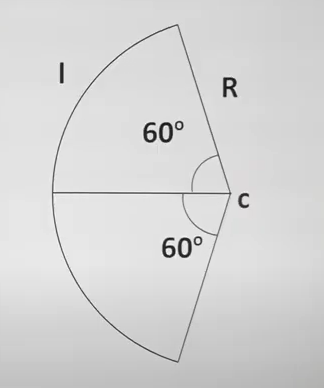 View Solution
View SolutionThe electric field at the centre of a uniformly charged ring is zero. What is the electric field and potential at the centre of a half ring if the charge on it be Q and its radius be R?
View Solution

