Using Gauss's Theorem, Derive an expression for electric field intensity due to uniformity charged hollow sphere : (b) At a point on the shell
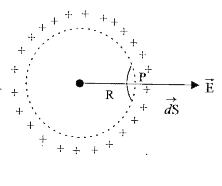
Electric field intensity at a point on the surface of shell.
Let P be the point on the surface of spherical shell such that r=R
According to Gauss theorem
∴ ∮S→E.−→dS=qɛ0
Since →E and →dS are in same direction
∴ θ=0∘
∮SEdScos0∘=qɛ0
∮SEdS=qɛ0 [∴E constant]
E∮SEdS=qɛ0
E×4πR2=qɛ0
:." "E=(sigma xx cancel(4 pi R^(2)))/(cancel(4 pi R^(2)) xx varepsilon_(0))=sigma/varepsilon_(0)" "[:.q=sigma xx 4 pi R^(2)]
E=σɛ0
Similar Questions
Derive an expression for electric field intensity at a point due to point charge.
View SolutionDerive an expression for electric field intensity at a point due to point charge.
View SolutionDerive an expression for electric intensity E due to a uniformly charged thin spherical shell at a point outside the shell.
View SolutionFind expression for electric field intensity due to a uniformly charged thin spherical shell at a point outside the shell.
View SolutionUsing Gauss’s theorem in electrostatics, deduce an expression for electric field intensity due to a charged spherical shell at a point (i) inside (ii) on its surface (iii) outside it. Graphically show the variation of electric field intensity with distance from the centre of shell.
View SolutionApply Gauss's theorem to find an expression for the electric field intensity at a point due to a point charge.
View SolutionDerive an expression for the electric field intensity at a point outside a charged conducting sphere.
View Solution(a) Using Gauss's law, derive an expression for the electric field intensity at any point outside a uniformly charged thin spherical shell of radius R and charge density σC/m2. Draw the field lines when the charge density of the sphere is (i) positive, (ii) negative.
(b) A uniformly charged conducting sphere of 2.5 m in diameter has a surface charge density of 100 μC/m2. Calculate the
(i) charge on the sphere
(ii) total electric flux passing through the sphere.
View Solution(a) Using Gauss's law, derive an expression for the electric field intensity at any point outside a uniformly charged thin spherical shell of radius R and charge density σC/m2. Draw the field lines when the charge density of the sphere is (i) positive, (ii) negative.
(b) A uniformly charged conducting sphere of 2.5 m in diameter has a surface charge density of 100 μC/m2. Calculate the
(i) charge on the sphere
(ii) total electric flux passing through the sphere.
View SolutionState Gauss's theorem in electrostatics. Apply this theorem to derive an expression for electric field intensity at a point outside a uniformly charged thin spherical shell.
View Solution
