Define drift velocity of electrons and derive its expression .
Drift velocity is defined as the average velocity with which the free electrons gets dirfted towards the positive end of the conductor under the influence of an external electric field applied .
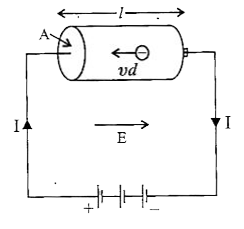
A metal conductor contaons a large number of free electrons . At room temperature these electrons move at random within the body of the conductor . The directions of motion of these free electrons are so randomly distributed that the average thermal velocity of the electrons is zero .
If →u1,→u2,→u3,...,−→un are random thermal velocities of n free electrons in the metal conductor then the average thermal velocity of electrons is ,
→u1+→u2+→u3+......+−→unn=0 .... (1)
As a result , there will be net flow of electrons or charge on one particular direction in a metal conductor ,hence no current .When some potential difference is applied across the two ends of conductor . An electric field is set up inside the conductor . The free electrons in the conductor experience a force in a direction opposite to that of electric field and are accelerated from negative end to positive end of the conductor .
If V is the potential difference applied across the ends of a conductor of length i , the magnitude of electric field set up is ,
E=Potential differencelength
E=Vl
Each free electron in the conductor experiences a force ,
→F=−e→E ..... (2)
Here the negative sign shows that the direction of force is opposite to that of electric field applied .
The acceleration of each electron is
→a=→Em
→a=−e→Em
Due to this acceleration , the free electron , apart from its thermal veocity , acquires additiona velocity component in a direction opposite to the direction of electric field . At any instant of time , the velocity acquired by electron having thermal velocity →u1 will be , →v1=→u1+→aτ1
where τ1 is the relaxation time .
Similarly , the velocities acquired by other electrons in the conductor will be ,
→v2=→u2+→aτ2
→v3=→u3+→aτ3
→vn=−→un+→aτn
According to definition , →vd=→v1+→v2+......+→vnn
→vd=(→u1+→aτ1)+(→u2+→aτ1)+.......(→u1+→aτ1)n
→vd=(→u1+→u2+.......+−→unn)+→a(τ1+τ2+.....+τn)n
→vd=(→u1+→u2+.......+−→unn)+→a(τ1+τ2+.....+τn)n
→vd=0+→aτ⇒→vd=→aτ .... (3)
where τ=τ1+τ2+....+τnn . It is called average relaxation time .
Putting the value of →a in eqn .(3) ,
→vd=−e→Eτm
vd=eEτm
The negative sign shown that →vd is opposite to the direction of →E .
Thus , →vd∝→E
Similar Questions
Knowledge Check
- Question 1 - Select One
The drift velocity of electrons is given by
Avd=emVltBvd=eVtlCvd=emtDvd=Vlmet
Define drift velocity of free electron . If relaxation time is r then write a vector relation b etween drift velocity and electric field.
View SolutionExplain the term 'drift velocity' of electrons in a conductor. Hence obtain the expression for the current through a conductor in terms of 'drift velocity'.
View SolutionExplain the term 'drift velocity' of electrons in a conductor. Hence obtain the expression for the current through a conductor in terms of drift - Temperature T velocity.
View SolutionDerive an expression for drift velocity of free electrons in a conductor in terms of relaxation time.
View SolutionDefine the term 'drift velocity' of electrons in a current carrying conductor. Obtain the relationship between the current density and the drift velocity of electrons.
View SolutionDefine the term 'drift velocity' of electrons in a current carrying conductor. Obtain the relationship between the current density and the drift velocity of electrons.
View SolutionDerive the expression for drift velocity of free electron in terms of relaxation time and electric field applied across a conductor.
View Solution
