One mole of an ideal gas at 300 K is heated at constant volume (V1 ) until its temperature is doubled, then it is expanded isothermally till it reaches the original pressure. Finally, the gas is cooled at the constant pressure till system reached to the half of original volume (V2). Determine the total work done(Vf/2) in calories: [Use in 2−0.70,K=2calK−1mol−1]Solution in Malayalam
60
40
20
10
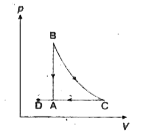
The temperature, pressure and volume conditions at each of the points A,B,C,D as show in are A(p1,V1,T1),B(2p1,V1,2T1)C(p1,2V1,2T1),D(2p1+V12,T12)
The total work done is given by Wtotal=WAB+WBC+WCD, For isochoric change (AB),WAB=0.
For isothermal expansion (BC),WBC=−1×R×2T1ln(2VV1)=−22×300×0.7=−840cal
For isobaric change (CD),WCD=0p1ΔV=−RΔV=−R[T12−2T1]=32RT1=32×2×300=900cal
Therefore , Wtotal=−840+900=60cal
Topper's Solved these Questions
- CHEMICAL THERMODYNAMICS BOOK - BRILLIANT PUBLICATIONCHAPTER - CHEMICAL THERMODYNAMICS EXERCISE - LEVEL-II (Assertion-Reason Type)
18 Videos
- CHEMICAL THERMODYNAMICS BOOK - BRILLIANT PUBLICATIONCHAPTER - CHEMICAL THERMODYNAMICS EXERCISE - LEVEL-III
52 Videos
- CHEMICAL THERMODYNAMICS BOOK - BRILLIANT PUBLICATIONCHAPTER - CHEMICAL THERMODYNAMICS EXERCISE - LEVEL-I
92 Videos
- CHEMICAL BONDING AND MOLECULAR STRUCTUREBOOK - BRILLIANT PUBLICATIONCHAPTER - CHEMICAL BONDING AND MOLECULAR STRUCTUREEXERCISE - QUESTION (LEVEL -ll)
42 Videos
- CLASSIFICATION OF ELEMENTS AND PERIODICITY IN PROPERTIESBOOK - BRILLIANT PUBLICATIONCHAPTER - CLASSIFICATION OF ELEMENTS AND PERIODICITY IN PROPERTIESEXERCISE - QUESTIONS (LEVEL-III ( Linked Comprehension Type ))
12 Videos
Similar Questions
Assertion : Heat of neutralisation of HNO3, and NaOH is almost same as that of HCl and KOH.
Reason : Both HNO3, and HCl are strong acids and NaOH and KOH are strong bases. : if both (A) and (R) are correct and (R) is the correct explanation of(A)., If both (A) and (R) are correct, but (R) is not the correct explanation of(A)., If(A) is correct, but (R) is incorrect., If both (A) and (R) are incorrect.
View Solution1.0 mole of a monoatomic ideal gas is expanded from state (1) to state (2) as shown in the figure. Calculate ti work done for the expansion of gas from state (1) to state (2) at 298 K
View SolutionIn ....... process, work is done at the expense of internal energy.
View SolutionWhich of the following species are hypervalent ?
(l) ClO−4
(ll) BF3
(lll) SO2−4
(lV) CO2−3
View SolutionThe heats of formation (ΔH∘f) of the oxides of the third period, sodium to chlorine, are in kJ mol−1
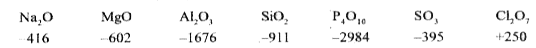
Based on these data, answer the following questions
Which oxide has maximum negative heat of formation per oxygen atom?
View SolutionAn ideal gas expands isothermally from volume V1 to V2 and is then compressed to original volume V1 adiabatically. Initialy pressure is P1 and final pressure is P3. The total work done is W. Then
View SolutionWork done by 0.1 mole of a gas at 27∘C to double its volume at constant pressure is (R=2 cal mol−1.∘K−1)
View SolutionA gas expands under constant pressure P from volume V1 toV2The work done by the gas is
View SolutionCalculate the work done by 0.1 mole of a gas at 27∘C to double its volume at constant pressure (R=2calmol−1K−1)
View SolutionOne mole of a diatomic ideal gas at 300 K is heated at constant volume until its pressure is doubled and then expanded adiabatically till its temperature falls to 300K. The work done by the gas is, if R is molar gas constant:
View SolutionAn ideal gas expands isothermally from volume V_(1) to volume V_(2) . It is then compressed to original volume V_(1) adiabatically . If p_(1),p_(2) and W represent the initial pressure , final pressure and the net work done by the gas respectively during the entrie process, then
View SolutionA cylinder contains an ideal gas at a pressure of two atmospheres, the volume being 5 litres at a temperature of 250 K. The gas is heated at constant volume to a pressure of 4 atmospheres and then at constant pressure to a temperature of 650 K. Calculate the total heat input during these processes. For the gas Cv=21Jmol−10C−1 . The gas is then cooled at constant volume to its original pressure and then at constant pressure to its original volume. Find the total heat output during these processes and the total work done by the gas in the whole cyclic process.
View SolutionA gas expands under constant pressure P from volume V_(1) to V_(2) The work done by the gas is
View SolutionAn ideal gas expands isothermally from volume V_(1) to V_(2) and is then compressed to original volume V_(1) adiabatically. Initialy pressure is P_(1) and final pressure is P_(3) . The total work done is W . Then
View Solutionn moles of a gas expands from volume V_(1) to V_(2) at constant temperature T. The work done by the gas is
View Solutionn moles of a gas expands from volume V1 to V2 at constant temperature T. The work done by the gas is
View Solution