A plane light wave of intensity I= 0.20W/ falls on a plane mirror surface with reflection coefficient . The angle of incidence is . In terms of corpuscular theory, find the magnitude of the normal pressure exerted on the surface.
The correct Answer is:C
Momentum corresponding to incident photons normal to the surface,
Since, reflection coefficient is , so the momentum of the reflected photons per second normal to surface,
Hence rate of change of momentum of the photons, from Newton.s third law,
hence pressure exerted on surface,
On substituting values, we get P= 0.06N/
Similar Questions
A plane light wave of intensity I=0.20Wcm−2 falls on a plane mirror surface with reflection coefficient ρ=0.8. The angle of incidence is 45∘. In terms of corpuscular theory, find the magnitude of the normal pressure exerted on that surface.
View SolutionA plane light wave of intensity I=0.20Wcm−2 falls on a plane mirror surface with reflection coefficient ρ=0.8. The angle of incidence is 45∘. In terms of corpuscular theory, find the magnitude of the normal pressure exerted on that surface.
View Solution
Knowledge Check
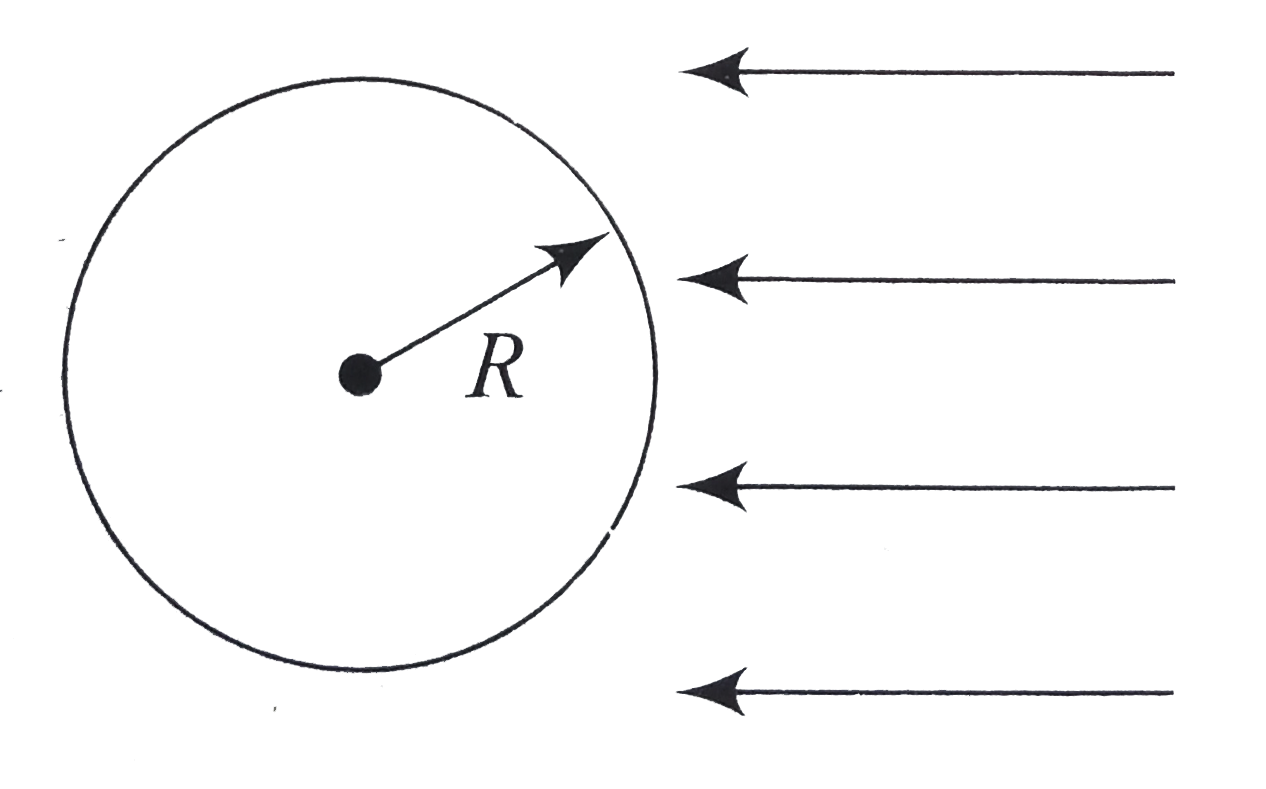
A plane wave of intensity I=0.70Wcm−2 illuminates a sphere with ideal mirror surface. The radius of sphere is R=5.0cm. From the standpoint of photon theory, find the force that light exerts on the sphere.A0.8μNB0.2μNC0.5μND1.2μNIf the intensity of the incident radiowave of 1watt/m2 is reflected by the surface, find the pressure exerted on the surface.
A7×10−9N/m2B8×10−9N/m2C6×10−10N/m2D10−19N/m2Light with an energy flux of 18W/cm2 falls on a non-reflecting surface at normal incidence. The pressure exerted on the surface is
A2N/m2B2×10−4N/m2C6N/m2D6×10−4N/m2
A plane light wave of intensity I=0.20W/cm2 falls on a plane mirror surface with reflection coefficient ρ=0.8. The angle of incidence is 45∘. In terms of the corpuscular theory find the magnitude of the normal pressure extered by light on that surface.
View SolutionA short light pulse of enegry E=7.5J falls in the form of a narrow and almost parallel beam on a mirror plate whose reflection coefficient is ρ=0.60. The angle of incidence is 30∘. In terms of the corpuscular theory find the momentum transfered to the plate.
View SolutionA palne light wave of intensity I=0.70W/cm2 illuminates a sphere with ideal mirror surface. The radius of the sphere is R=5.0cm. From the standpoint of the corpuscular theory find the force that light exerts on the sphere.
View SolutionIf the intensity of the incident radio wave of 1watt/m2 is reflected by the surface, find the pressure exerted on the surface.
View Solution
A plane wave of intensity I=0.70Wcm−2 illuminates a sphere with ideal mirror surface. The radius of sphere is R=5.0cm. From the standpoint of photon theory, find the force that light exerts on the sphere.
View Solution
