If which of the following is true?
, for as well as for
, for as well as for
, for as well as for
None of these
The correct Answer is:D
To solve the problem, we need to analyze the function f(x)=(x−a)2(x−b)(x−c)(x−d)(x−e) given the conditions a<b<c<d<e.
1. Understanding the Function: The function f(x) consists of a squared term (x−a)2 and four linear factors (x−b),(x−c),(x−d),(x−e). The squared term will always be non-negative (i.e., ≥0) for any real value of x.
2. Identifying Roots: The roots of the function occur at x=a, x=b, x=c, x=d, and x=e. The squared term at x=a means that f(x) will touch the x-axis at x=a but will not cross it.
3. Behavior of the Function:
- For x<a: All terms (x−b),(x−c),(x−d),(x−e) are negative, thus f(x) is positive (since (x−a)2 is positive and the product of four negative numbers is positive).
- For a<x<b: (x−a)2 is positive, (x−b) is negative, (x−c),(x−d),(x−e) are also negative. Thus, f(x) is positive (positive times three negatives).
- For b<x<c: (x−a)2 is positive, (x−b) is positive, (x−c) is negative, (x−d),(x−e) are negative. Thus, f(x) is negative (positive times one positive and three negatives).
- For c<x<d: (x−a)2 is positive, (x−b) is positive, (x−c) is positive, (x−d) is negative, (x−e) is negative. Thus, f(x) is negative (positive times three positives and one negative).
- For d<x<e: (x−a)2 is positive, (x−b) is positive, (x−c) is positive, (x−d) is positive, (x−e) is negative. Thus, f(x) is positive (positive times four positives and one negative).
- For x>e: All terms are positive, hence f(x) is positive.
4. Conclusion: From the analysis, we find that:
- f(x) is negative when x is between b and c and also when x is between c and d.
- Therefore, the correct option is that f(x) is negative when x is between b and c and also between d and e.
Final Answer:
The correct statement is: f(x) is negative when x is between b and c and also when x is between d and e.
- For x<a: All terms (x−b),(x−c),(x−d),(x−e) are negative, thus f(x) is positive (since (x−a)2 is positive and the product of four negative numbers is positive).
- For a<x<b: (x−a)2 is positive, (x−b) is negative, (x−c),(x−d),(x−e) are also negative. Thus, f(x) is positive (positive times three negatives).
- For b<x<c: (x−a)2 is positive, (x−b) is positive, (x−c) is negative, (x−d),(x−e) are negative. Thus, f(x) is negative (positive times one positive and three negatives).
- For c<x<d: (x−a)2 is positive, (x−b) is positive, (x−c) is positive, (x−d) is negative, (x−e) is negative. Thus, f(x) is negative (positive times three positives and one negative).
- For d<x<e: (x−a)2 is positive, (x−b) is positive, (x−c) is positive, (x−d) is positive, (x−e) is negative. Thus, f(x) is positive (positive times four positives and one negative).
- For x>e: All terms are positive, hence f(x) is positive.
- f(x) is negative when x is between b and c and also when x is between c and d.
- Therefore, the correct option is that f(x) is negative when x is between b and c and also between d and e.
The correct statement is: f(x) is negative when x is between b and c and also when x is between d and e.
Topper's Solved these Questions
- BOOK - DISHA PUBLICATIONCHAPTER - FUNCTIONSEXERCISE - Test Yourself15videos
FUNCTIONS
- BOOK - DISHA PUBLICATIONCHAPTER - FUNCTIONSEXERCISE - Practice Exercises (Standard Level)30videos
FUNCTIONS
- BOOK - DISHA PUBLICATIONCHAPTER - COORDINATE GEOMETRYEXERCISE - TEST YOURSELF15videos
COORDINATE GEOMETRY
- BOOK - DISHA PUBLICATIONCHAPTER - FundamentalsEXERCISE - Test Yourself15videos
Fundamentals
Similar Questions
Knowledge Check
- Question 1 - Select One
If a<b<c<d, then the eqution 3(x−a)(x−c)+5(x−b)(x−d)=0 has
Areal and distinct rootsBreal and equal rootsCpurely imaginary rootsDnone of these - Question 2 - Select One or More
If a<b<c<d, then the quadratic equation (x−a)(x−c)+2(x−b)(x−d)=0 has its roots
ArealBimaginaryCone root in (a,c)Dboth roots in (a,c) - Question 3 - Select One or More
Let f(x)={−3,−3≤x<0x2−3,0<x≤3andg(x)=|f(x)|+f(|x|), then which of the following is true ?
Aat x=0,g(x) is continuous as well as differentiableBatx=√3,g(x) is continuous but not differentiableCatx=2,g(x) is neither continuous nor differentiableDNone of these
Let f (x) be a polynomial function of degree 3 where a<b<candf(a)=f(b)=f(c). If the graph of f (x) is as shown, which of the following statements are INCORRECT ? (Where c>|a|)
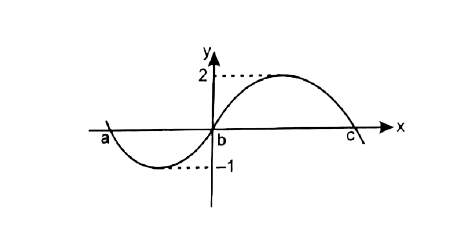 View Solution
View SolutionIf a<b<c<d, then the roots of the equation (x-a)(x-c)+2(x-b)(x-d)=0 are
View SolutionSuppose 0<b<candf(x)=x2−bc2x−(b+c),x∈R, then f(x) cannot lie in
View SolutionIf 0<x<5 and 1<y<2, then which of the following is true ?
View Solutionif a <c<b, then check the nature of roots of the equation
(a−b)2x2+2(a+b−2c)x+1=0View Solution

