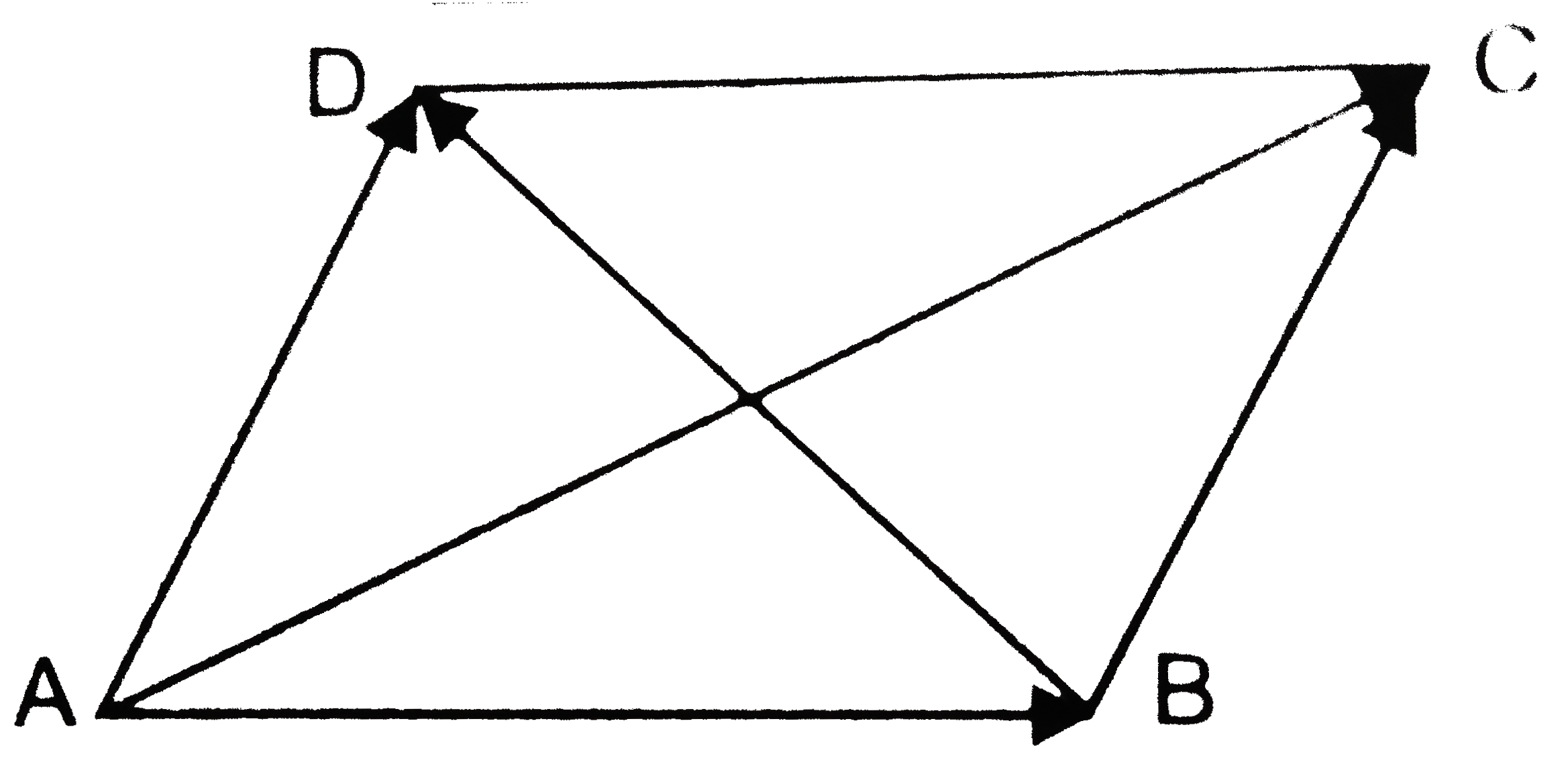
Let ABCD be a parallelogram whose diagonals intersect at P and let O be the origin. What is −−→OA+−−→OB+−−→OC+−−→OD equal to
4−−→OP
2−−→OP
−−→OP
Null vector
The correct Answer is:A
Step by step video, text & image solution for Let ABCD be a parallelogram whose diagonals intersect at P and let O be the origin. What is vec(OA) + vec(OB) + vec(OC ) + vec(OD) equal to by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Knowledge Check
Let ABCD be a parallelogram whose diagonals intersect at P and O be the origin, then OA+OB+OC+OD equals
AOPB2OPC3OPD4OPABCD is a parallelogram with AC and BD as diagonals. Then, A→C−B→D=
A4A→BB3A→BC2A→BDA→BIf ABCD is a parallelogram, then −−→AC−−−→BD=
A4−−→ABB3−−→ABC2−−→ABD−−→AB
If ABCD is a rhombus whose diagonals cut at the origin O, then proved that →OA+→OB+→OC+→OD+→O.
View SolutionABCD is a parallelogram Fig. 2 (c ) .64. ACand(BD)areitsdiagonals.Showtˆ<br> (a) vec (AC) +vec (BD) =2 vec (BC)<br>(b)vec (AC) - vec (BD) =2 vec (AB)
 .
.
View SolutionABCD is parallelogram and P is the point of intersection of its diagonals. If O is the origin of reference, show that →OA+→OB+→OC+→OD=4→OP.
View SolutionIf ABCD is a rombus whose diagonals cut at the origin O, then OA + OB + OC + OD equals to
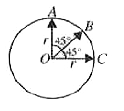
View SolutionThe resultant of the three vectors −−→OA,−−→OB and −−→OC shown in figure is
View Solution
