Derive an expression for-electric field intensity at any point on equatorial line neutral line of dipole.
Or
Two point charges - q and + q are placed at a distance '2a' apart. Calculate the electric field intensity at a point A situated at a distance r along the perpendicular bisector of the line joining the charges.

Text Solution

Verified by Experts

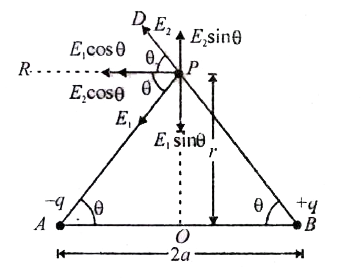
Let us take an electric dipole which consists of two point charges - q and + q separated by very small distance 2a. We wish to calculate the electric field intensity at point P, on equatorial line of dipole.
Electric field intensity at P due to - q charge placed at point A is given by
$| {\vec{E}}_{1} | = \frac{1}{4 π ɛ_{0}} \frac{q}{{(A P)}^{2}}$
$\Rightarrow | {\vec{E}}_{1} | = \frac{1}{4 π ɛ_{0}} \frac{q}{(a^{2} + r^{2})} ... ... . (i)$
The direction of E, is along PA.
Resolve $E_{1}$ into two components $E_{1} \cos θ$ along $P R and E_{1} \sin θ$ along PO. Electric field intensity at P, due to +q charge placed at B is given by :
$| {\vec{E}}_{2} | = \frac{1}{4 π ɛ_{0}} \frac{q}{{(B P)}^{2}} = \frac{1}{4 π ɛ_{0}} \frac{q}{(a^{2} + r^{2})} ... ... ... (i i)$
The direction of $E_{2}$ is along PD.
Resolve $E_{2}$ into two components. i.e., $E_{2} \sin θ$ along PF and $E_{2}, \cos θ$ along PR. The components $E_{1} \sin θ$ along PO and $E_{2}, \sin θ$ along PF are equal and opposite and hence they cancel each other.
Hence, net electric field intensitv at P is given by
$E = E_{1} \cos θ + E_{2} \cos θ$
Substitute the values of $| {\vec{E}}_{1} | and | {\vec{E}}_{2} |$ from equations $(i) and (i i)$ , we have
$E = \frac{1}{4 π ɛ_{0}} \frac{q}{(a^{2} + r^{2})} \cos θ + \frac{1}{4 π ɛ_{0}} \frac{q}{(a^{2} + r^{2})} \cos θ$
$\Rightarrow E = 2 [\frac{1}{4 π ɛ_{0}} . \frac{q}{(a^{2} + r^{2})} \cos θ] ... ... . (i i i)$
$\Rightarrow From Δ A O P, \cos θ = \frac{O A}{A P} \Rightarrow \cos θ = \frac{a}{{(a^{2} + r^{2})}^{\frac{1}{2}}}$
Putting the value of $\cos θ$ in equation (iii), we get
$E = 2 [\frac{1}{4 π ɛ_{0}} . \frac{q 2 a}{(a^{2} + r^{2})} . \frac{a}{{(a^{2} + r^{2})}^{1 / 2}}]$
$\Rightarrow E = \frac{1}{4 π ɛ_{0}} \frac{q 2 a}{{(a^{2} + r^{2})}^{3 / 2}}$
$\Rightarrow E = \frac{1}{4 π ɛ} . \frac{p}{{(a^{2} + r^{2})}^{3 / 2}} [∴ p = q \times 2 a]$

Updated on:21/07/2023