Length of a side of a square inscribed in a circle is a√2 units. The circumference of the circle is
2πa units
πa units
4πa units
2aπ units
The correct Answer is:A
To find the circumference of a circle in which a square is inscribed, we can follow these steps:
Step 1: Understand the relationship between the square and the circle
The diagonal of the inscribed square is equal to the diameter of the circle. If the side length of the square is given as a√2, we can find the diagonal using the Pythagorean theorem.
Step 2: Calculate the diagonal of the square
The formula for the diagonal d of a square with side length a is:
d=a√2
In this case, the side length a is a√2. Therefore, the diagonal d is:
d=(a√2)√2=a⋅2=2a
Step 3: Relate the diagonal to the diameter of the circle
Since the diagonal of the square is equal to the diameter D of the circle, we have:
D=2a
Step 4: Calculate the radius of the circle
The radius R of the circle is half of the diameter:
R=D2=2a2=a
Step 5: Calculate the circumference of the circle
The formula for the circumference C of a circle is:
C=2πR
Substituting the value of the radius:
C=2πa
Conclusion
Thus, the circumference of the circle is:
Circumference=2πa
---
The diagonal of the inscribed square is equal to the diameter of the circle. If the side length of the square is given as a√2, we can find the diagonal using the Pythagorean theorem.
The formula for the diagonal d of a square with side length a is:
d=a√2
In this case, the side length a is a√2. Therefore, the diagonal d is:
d=(a√2)√2=a⋅2=2a
Since the diagonal of the square is equal to the diameter D of the circle, we have:
D=2a
The radius R of the circle is half of the diameter:
R=D2=2a2=a
The formula for the circumference C of a circle is:
C=2πR
Substituting the value of the radius:
C=2πa
Thus, the circumference of the circle is:
Circumference=2πa
Topper's Solved these Questions
- BOOK - KIRAN PUBLICATIONCHAPTER - MENSURATIONEXERCISE - TYPE -II386videos
MENSURATION
- BOOK - KIRAN PUBLICATIONCHAPTER - MENSURATIONEXERCISE - TYPE - III21videos
MENSURATION
- BOOK - KIRAN PUBLICATIONCHAPTER - LCM AND HCF EXERCISE - Test Yourself18videos
LCM AND HCF
- BOOK - KIRAN PUBLICATIONCHAPTER - MISCELLANEOUSEXERCISE - TYPE-VI15videos
MISCELLANEOUS
Similar Questions
Knowledge Check
- Question 1 - Select One
The largest possible square is inscribed in a circle of 2π units circumference. The area of the square in square units is
A4π√2B2π√2C√2D2 - Question 2 - Select One
If the side of a square is 24 cm, then the circumference of its circumscribed circle ( in cm ) is :
A24√3πB24√2πC12√2πD24π - Question 3 - Select One
The area of the largest possible square inscribed in a circle of unit radius ( in square unit ) is :
A2BπC(2√2)πD(4√2)π
A regular polygon of 9 sides where length of each side is 2 is inscribed in a circle. Then the radius of the circle is
View SolutionShow that the rectangle of maximum area that can be inscribed in a circle is a square.
View SolutionFind the area of largest possible square inscribed in a circle of unit radius.
View SolutionIf a square is inscribed in a circle, find the ratio of the areas of the circle and the square.
View Solution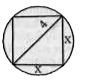
A square of side x is inscribed in a circle of radius 4 cm. Then x isView Solution

