Find the point where the straight line 2x−3y=12 cuts x-axis and y -axis Also find the length intercepted by the line between the axes.
The correct Answer is:(0,−4)
Step by step video, text & image solution for Find the point where the straight line 2x - 3y = 12 cuts x-axis and y -axis Also find the length intercepted by the line between the axes. by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Topper's Solved these Questions
- BOOK - LUCENT PUBLICATIONCHAPTER - GRAPHICAL SOLUTION OF LINEAR EQUATION EXERCISE - EXERCISE-3A46videos
GRAPHICAL SOLUTION OF LINEAR EQUATION
- BOOK - LUCENT PUBLICATIONCHAPTER - GRAPHICAL SOLUTION OF LINEAR EQUATION EXERCISE - EXERCISE-3B8videos
GRAPHICAL SOLUTION OF LINEAR EQUATION
- BOOK - LUCENT PUBLICATIONCHAPTER - ELEMENTARY TRIGONOMETRIC IDENTITIES EXERCISE - EXERCISE 11B37videos
ELEMENTARY TRIGONOMETRIC IDENTITIES
- BOOK - LUCENT PUBLICATIONCHAPTER - HEIGHT AND DISTANCEEXERCISE - EXERCISE-12B14videos
HEIGHT AND DISTANCE
Similar Questions
Find the points when the graph of the equation 3x+4y=12 cut the x-axis and y -axis.
View SolutionDraw the graph of line 4x+3y=24 Write the coordinates of points where this line intersects the x-axis and y-axis. Use this graph to find the area of the triangle formed by the line and the coordinates axes.
View SolutionFind the length of intercept b/w both axis by a line 3x+4y=12
View SolutionFor the straight line √3y−3x=3, find the intercepts on the x-axis and y-axis.
View SolutionFind the inclination of AC and the slope of AC and intercepts on the x-axis and y-axis by the line AC.
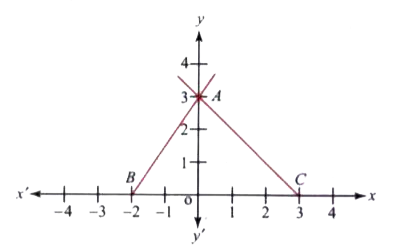
Also, find the slope of AB and the y-intercept made by the line AB.View SolutionFind the equation of the straight line through the point P(a,b) parallel to the line xa+yb=1 also find the intercepts made by it on the axes .
View SolutionReduce the equation 3x-4y+12=0 to intercepts form. Hence, find the length of the portion of the line intercepted between the axes.
View SolutionFind the equation of the straight line whose intercepts on X-axis and Y-axis are respectively twice and thrice of those by the line 3x+4y=12.
View Solution(i) Find the intercepts made by line 5x−2y=10 on both axes. Also find the length of segment between the axes made by lines.
(ii) Find the equation of a line whose X and Y intercepts are respectively 3 and 4 times of the intercepts of the line 2x+3y=6.View SolutionFind the intercepts cut off by the line 2x-3y+18=0 on the coordinate axes
View Solution

