O is the circum centre of the triangle ABC with circumradius 13 cm. Let BC = 24 cm and OD is perpendicular to BC. Then the length of OD is
3 cm
4 cm
5 cm
7 cm
The correct Answer is:C
To find the length of OD, where O is the circumcenter of triangle ABC, we can use the properties of circles and triangles. Here’s a step-by-step solution:
Step 1: Identify the Given Information
- Circumradius R=13 cm
- Length of side BC=24 cm
Step 2: Understand the Geometry
Since O is the circumcenter, it is the center of the circumcircle of triangle ABC. The line OD is perpendicular to BC and bisects BC at point D.
Step 3: Calculate the Length of BD and DC
Since D is the midpoint of BC:
BD=DC=BC2=242=12 cm
Step 4: Apply the Pythagorean Theorem
In triangle ODB, we can apply the Pythagorean theorem:
OB2=OD2+BD2
Where:
- OB=R=13 cm
- BD=12 cm
Substituting the values:
132=OD2+122
169=OD2+144
Step 5: Solve for OD2
Rearranging the equation to isolate OD2:
OD2=169−144
OD2=25
Step 6: Find the Length of OD
Taking the square root of both sides:
OD=√25=5 cm
Final Answer
The length of OD is 5 cm.
---
- Circumradius R=13 cm
- Length of side BC=24 cm
Since O is the circumcenter, it is the center of the circumcircle of triangle ABC. The line OD is perpendicular to BC and bisects BC at point D.
Since D is the midpoint of BC:
BD=DC=BC2=242=12 cm
In triangle ODB, we can apply the Pythagorean theorem:
OB2=OD2+BD2
Where:
- OB=R=13 cm
- BD=12 cm
132=OD2+122
169=OD2+144
Rearranging the equation to isolate OD2:
OD2=169−144
OD2=25
Taking the square root of both sides:
OD=√25=5 cm
The length of OD is 5 cm.
Topper's Solved these Questions
- CENTRE OF TRIANGLEBOOK - LUCENT PUBLICATIONCHAPTER - CENTRE OF TRIANGLEEXERCISE - EXERCISE-6A
59 Videos
- ALGEBRAIC IDENTITIES BOOK - LUCENT PUBLICATIONCHAPTER - ALGEBRAIC IDENTITIES EXERCISE - Exercise - 1B
28 Videos
- CIRCLE AND ITS TANGENT LINESBOOK - LUCENT PUBLICATIONCHAPTER - CIRCLE AND ITS TANGENT LINESEXERCISE - EXERCISE 8B
27 Videos
Similar Questions
M is the circumcentre of △ABC with circumradius 15 cm.Let BC = 24 cm and ML is perpendicular to BC. Then the length of ML is:
M त्रिभुज ABC का परिकेंद्र है, जिसकी परित्रिज्या 15 सेमी है। मान लीजिए कि BC = 24 सेमी है और ML, BC पर लम्ब है। तो ML की लंबाई ज्ञात कीजिए।
View SolutionIn a triangle ABC, ∠B=90∘,AB=8cm,BC=6cm. BD is perpendicular to AC, then find the length of CD, AD and BD.
View Solution
Knowledge Check
In triangle ABC,∠BAC=90∘and AD is perpendicular to BC. If AD=6cmand BD=4cm, then the length of BC is:
A10 cmB12 cmC13 cmD15 cm.
In the figure above, ABC is a triangle in which BC=24 cm and AC=13 cm. If AD is the perpendicular bisector of BC, then find the length of AD.A7 cmB12 cmC13 cmD5 cmIn the given figure, O is the centre of a circle of radius 13 cm and AB is a chord perpendicular to OD. If CD = 8 cm, then what is the length (in cm) of AB?
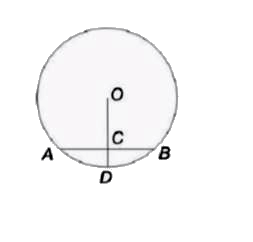 AA)6 cmBB)12 cmCC)24 cmDD)28cm
AA)6 cmBB)12 cmCC)24 cmDD)28cm

In the figure above, ABC is a triangle in which BC=24 cm and AC=13 cm. If AD is the perpendicular bisector of BC, then find the length of AD.
View Solution
In the figure above, ABC is a triangle in which BC=10 cm and AC=13 cm. If AD is the perpendicular bisector of BC, then find the length of AD.
View SolutionABC is a triangle. AB = 5cm, AC =√41cm and BC = 8cm. AD is perpendicular to BC. What is the area (in cm2) of triangle ABD?
View Solution
In the figure above, ABC is a triangle in which BC=10 cm and AC=13 cm. If AD is the perpendicular bisector of BC, then find the length of AD.
View SolutionG is the centroid of the triangle ABC, where AB, BC and CA are 7 cm, 24 cm and 25 cm respectively, then BG is:
G त्रिभुज ABC का केन्द्रक है जिसमें AB, BC और CA क्रमशः 7 सेमी, 24 सेमी और 25 सेमी हैं | BG का मान ज्ञात करें |
View Solution
