A cylindrical bucket of height 36 cm and radius 21 cm is filled with sand. The bucket is emptied on the ground and a conical heap of sand is formed, the height of the heap being 12 cm. The radius of the heap at the base is :
63 cm
53 cm
56 cm
66 cm
The correct Answer is:A
To solve the problem step by step, we will first calculate the volume of the cylindrical bucket and then equate it to the volume of the conical heap formed from the sand.
Step 1: Calculate the volume of the cylindrical bucket
The formula for the volume of a cylinder is given by:
Vcylinder=πr2h
Where:
- r = radius of the cylinder = 21 cm
- h = height of the cylinder = 36 cm
Substituting the values:
Vcylinder=π(21)2(36)
=π×441×36
=15876πcm3
Step 2: Set up the volume of the conical heap
The formula for the volume of a cone is given by:
Vcone=13πr2h
Where:
- r = radius of the cone (which we need to find)
- h = height of the cone = 12 cm
Step 3: Equate the volumes
Since the volume of the sand in the cylindrical bucket is equal to the volume of the conical heap, we have:
Vcylinder=Vcone
15876π=13πr2(12)
Step 4: Simplify the equation
We can cancel π from both sides:
15876=13r2(12)
15876=4r2
Step 5: Solve for r2
Now, we can solve for r2:
r2=158764
r2=3969
Step 6: Find r
Taking the square root of both sides gives us:
r=√3969
r=63cm
Conclusion
The radius of the heap at the base is 63cm.
---
The formula for the volume of a cylinder is given by:
Vcylinder=πr2h
Where:
- r = radius of the cylinder = 21 cm
- h = height of the cylinder = 36 cm
Vcylinder=π(21)2(36)
=π×441×36
=15876πcm3
The formula for the volume of a cone is given by:
Vcone=13πr2h
Where:
- r = radius of the cone (which we need to find)
- h = height of the cone = 12 cm
Since the volume of the sand in the cylindrical bucket is equal to the volume of the conical heap, we have:
Vcylinder=Vcone
15876π=13πr2(12)
We can cancel π from both sides:
15876=13r2(12)
15876=4r2
Now, we can solve for r2:
r2=158764
r2=3969
Taking the square root of both sides gives us:
r=√3969
r=63cm
The radius of the heap at the base is 63cm.
Topper's Solved these Questions
- BOOK - DISHA PUBLICATIONCHAPTER - MENSURATIONEXERCISE - Practice Exercises ( Foundation Level)70videos
MENSURATION
- BOOK - DISHA PUBLICATIONCHAPTER - MENSURATIONEXERCISE - Practice Exercises (STANDARD LEVEL)63videos
MENSURATION
- BOOK - DISHA PUBLICATIONCHAPTER - LOGARITHMSEXERCISE - Test Yourself15videos
LOGARITHMS
- BOOK - DISHA PUBLICATIONCHAPTER - MOCK TEST - 3EXERCISE - Multiple Choice Questions20videos
MOCK TEST - 3
Similar Questions
A cylindrical bucket, 32 cm high and with radius of base 18 cm, is filled with sand. This bucket is emptied on the ground and a conical heap of sand is formed. If the height of the conical heap is 24 cm, find the radius and slant height of the heap.
View SolutionA cylindrical bucket, 32 cm high and with radius of base 18 cm, is filled with sand. This bucket is emptied out on the ground and a conical heap of sand is formed. If the height of the conical heap is 24 cm, find the radius and slant height of the heap.
View Solution
Knowledge Check
- Question 1 - Select One
A cylindrical vessel 16 cm high and 9 cm as the radius of the base, is filled with sand. This vessel is emptied on the ground and a conical heap of sand is formed. If the height of the conical heap is 12 cm, the radius of its base is
A12 cmB18 cmC36 cmD48 cm - Question 2 - Select One
A cylindrical container of 32 cm height and 18 cm radius is filled with sand. Now all this sand is used to form a conical heap of sand. If the height of the conical heap is 24 cm, what is he radius of its base ?
Aa.12cmBb.24 cmCc.36 cmDd.48 cm
A cylindrical bucket, 32 cm high and 18 cm of radius of the base, is filled with sand. This bucket is emptied on the ground and a conical heap of sand is formed. If the height of the conical heap is 24 cm, find the radius and slant height of the heap.
View SolutionA cylindrical bucket, whose height is 27 cm and base radius is 48 cm, is filled with sand. When the bucket is emptied on the ground, a conical heap of radius 54 cm is formed. What is the height ( in cm ) of the heap ?
एक बेलनाकार (सिलिंड्रिकल) बाल्टी, जिसकी ऊंचाई 27 cm और आधार त्रिज्या 48 cm है, को रेत (सैंड) से भरा जाता है | जब बाल्टी को जमीन पर खाली किया जाता है और 54 cm त्रिज्या का एक शंकुकार ढेर बन जाता है | ढेर की ऊंचाई (cm में) कितनी है?View SolutionA girl fills a cylindrical bucket 32 cm in height and 18 cm in radius with sand .She empties the bucket on the ground and makes a conical heap of the sand .If the height of the conical heap is 24 cm find (i) its radius (ii) its slant height.
View SolutionA cylindrical bucket of diameter 28 cm and heigt 20 cm full of sand. When the sand in the bucket was poured on the ground, the sand
got converted into a shape of acone if the height of the cone was 14 cm, What was the base area of the cone?
Given :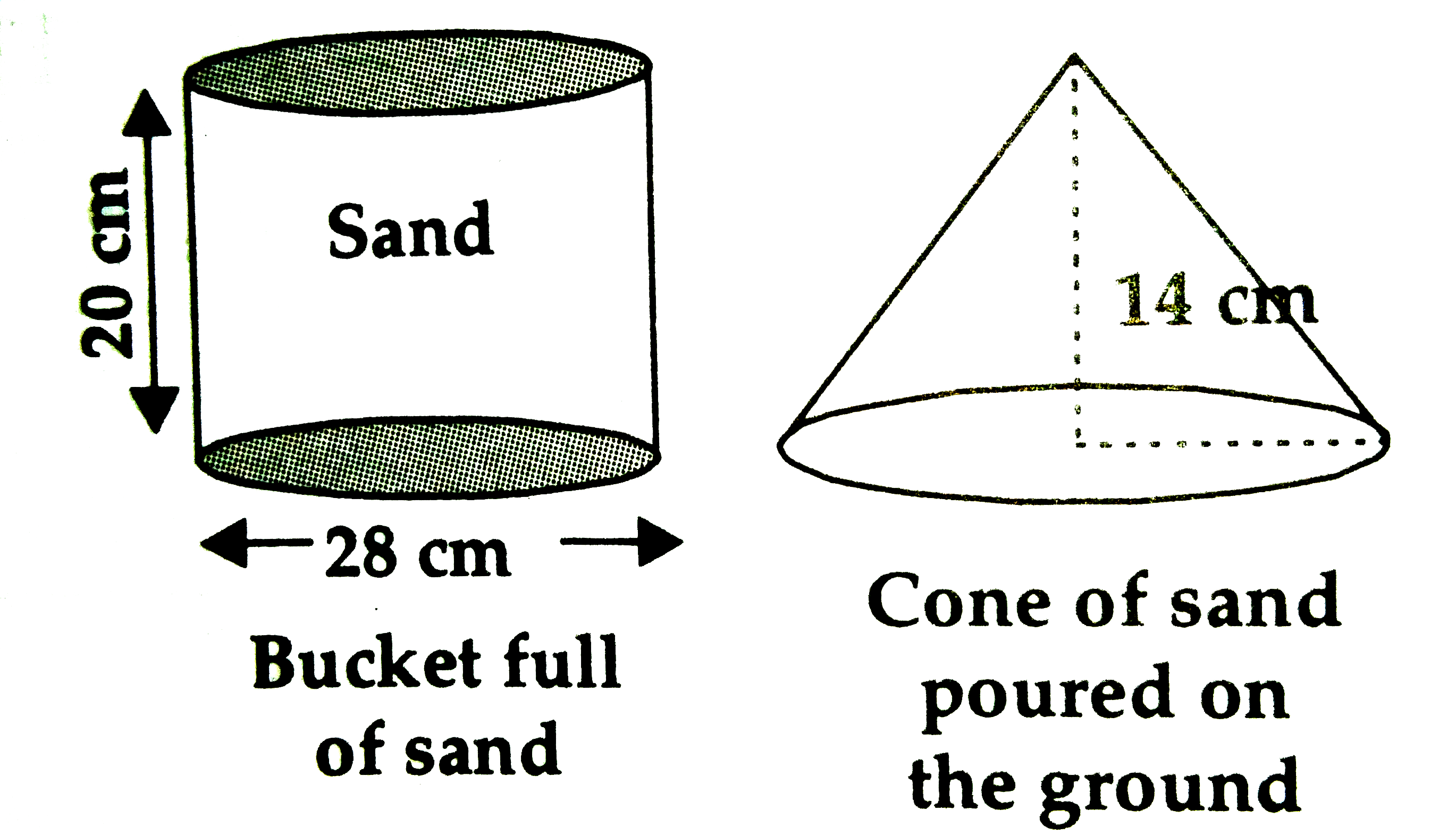
For cylinderical bucket,
r=Diameter2=14cm
h=20cm
To find: Base area of the coneView SolutionA cylinder bucket of diameter 28 cm and height 20 cm was full of sand. When the sand in the bucket was poured on the ground, the sand got converted into a shape of a cone. If the height of the cone was 14 cm , what was the base area of the cone?
View SolutionA girl empties a cylindrical bucket full of sand, of base radius 18 cm and height 32 cm on the floor to form a conical heap of sand. If the height of this conical heap is 24 cm, then find its slant height correct to one place of decimal.
View Solution

