A wire of length l is used to form a rectangle of sides 40 cm and 10 cm. The coil is placed in a uniform magnetic field of flux density 0.2 T in such a way that flux lines are perpendicular to the plane of the coil. The rectangle is changed to a square in 0.3s. Find induced emf developed in the coil.
Here, length of rectangle, L = 40 cm = 0.4m
Breadth of rectangle, B = 10 cm = 0.1m
Area of rectangle, A1=L×B=0.4×0.1
=0.04m2
Length of conductor, l=2×(L+B)
=2×(40+10)
= 100 cm = 1m
∴ In case of square, length of side
=1004=25cm=0.25m
Area of square, A2=(side)2=(0.25)2=0.0625m2
Induced emf =ΔϕΔt=B(A2−A1)0.3
=0.2(0.0625−0.04)0.3
= 0.015 V
Similar Questions
A rectangular copper coil is placed in a uniform magnetic field of induction 40 mT with its plane perpendicular to the field. The area of the coil is shrinking at a constant rate of 0.5m2s−1. The emf induced in the coil is
View SolutionA conducting wire is bent in the form of a square of side 8 cm and is placed in a region of magnetic field of 0.05 T, such that the plane of coil is perpendicular to the magnetic field. If the side of square starts decreasing at a rate of 10−3ms−1, what will be the emf induced in the coil when the area of coil is reduced to just half?
View Solution
Knowledge Check
A rectangular copper coil is placed in a uniform magnetic field of induction 40 mT with its plane perpendicular to the field. The area of the coil is shrinking at a constant rate of 0.5m2s−1. The emf induced in the coil is
A10 mVB20 mVC80 mVD40 mVA coil of area 10 cm2 has 200 turns. Magnetic field of 0.1 Wb/m2 is perpendicular to the plane of the coil. The field is reduced to zero in 0.1 s, the induced emf in the coil is
A1VB0.2VC2VD0A coil of 10 turns and area 4×10−2m2 is placed in a unifrom magnetic field of flux dencity 10−2T .If it is removed completely in 0.5s ,then the induced in the coil is
A2mVB8mVC16mVD20mV
A flexible wire bent in the form of a circle is place in a uniform magnetic field perpendicularly to the plane of the coil. The radius of the coil changes as shown in Figure. The graph of magnetude of induced emf in the coil is represented by
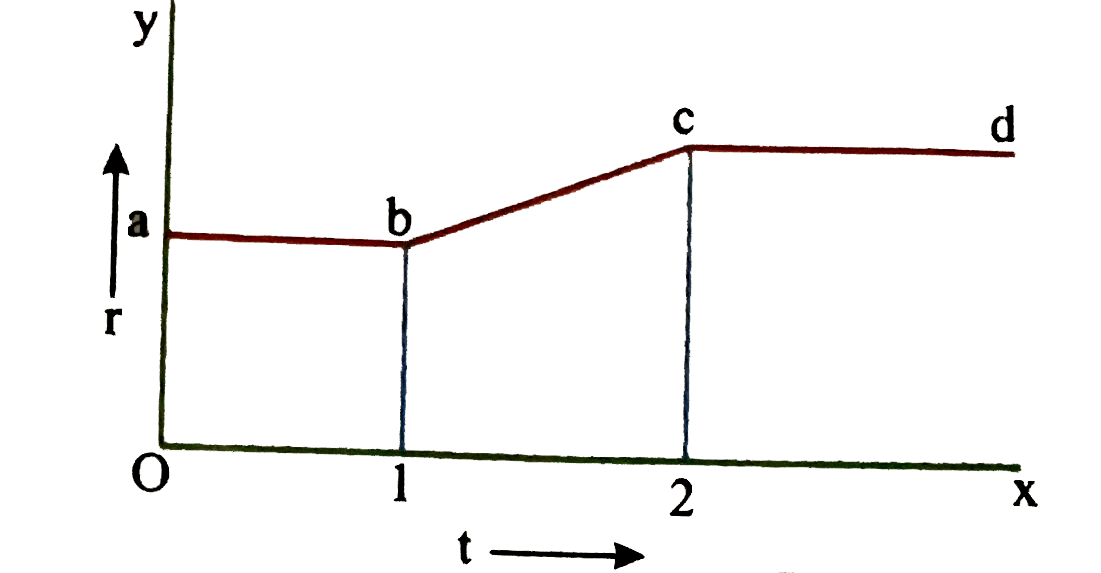
View SolutionA flexible wire bent in the form of a circle is place in a uniform magnetic field perpendicularly to the plane of the coil. The radius of the coil changes as shown in Figure. The graph of magnetude of induced emf in the coil is represented by

View SolutionA flexible wire bent in the form of a circle is placed in a uniform magnetic field perpendicular to the plane of the coil. The radius of the coil changing as shown in figure. The graph of induced emf in the coil is represented by
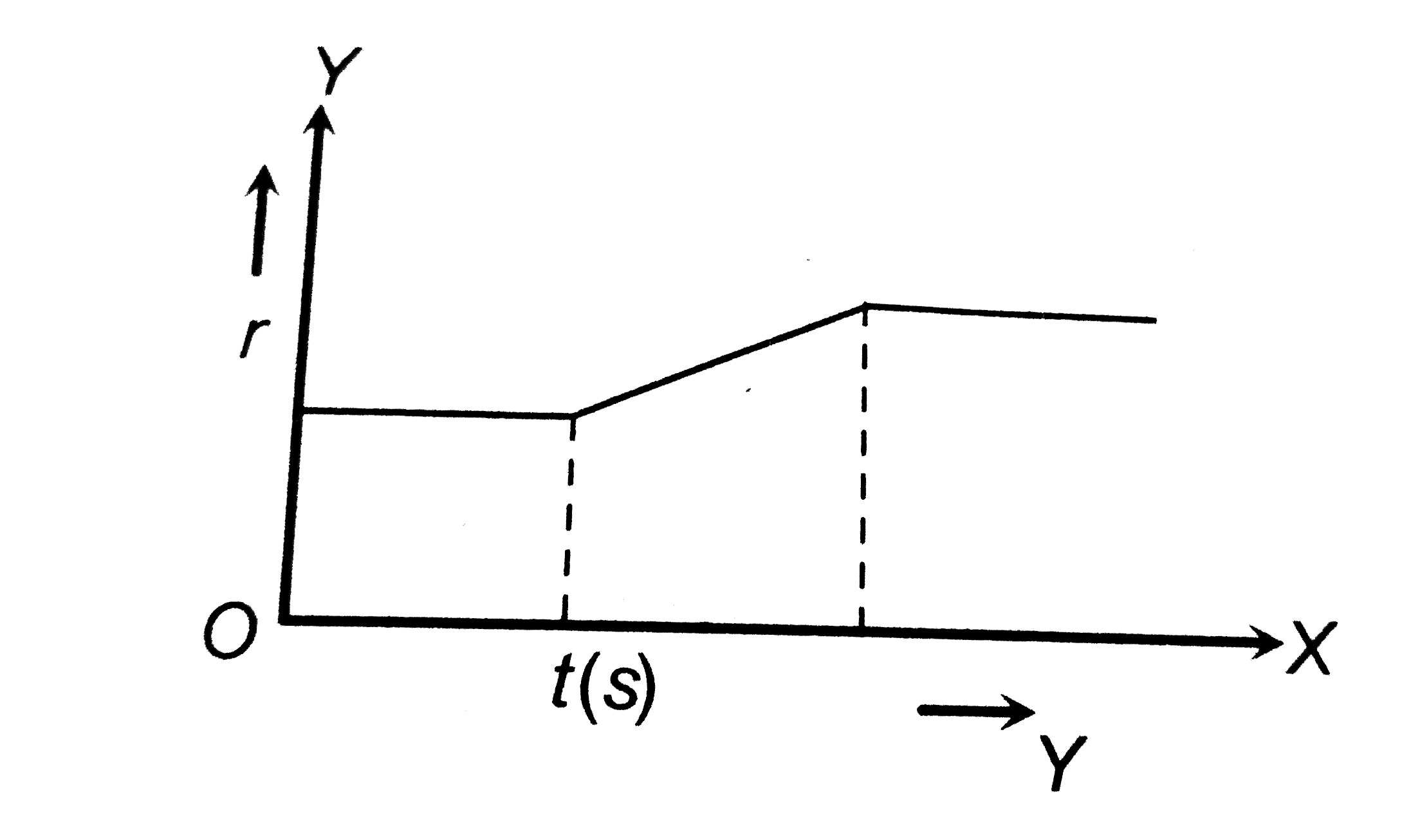
View SolutionA flexible wire bent in the form of a circle is place in a uniform magnetic field perpendicularly to the plane of the coil. The radius of the coil changes as shown in Figure. The graph of magnetude of induced emf in the coil is represented by

View SolutionThe magnetic flux linked with the coil changes from 0.1 Wb to 0.04 Wb in 3 s. the emf induced in the coil is
View Solution
