Which of the following represents Gay Lussac's law ?
I.PT= constant
II. P1T2=P2T1
III.P1V1=P2V2
Choose the correct option .
I,II and III
II and III
I and III
I and II
The correct Answer is:D
To determine which of the given options represents Gay-Lussac's law, let's analyze each option step by step.
Step 1: Understand Gay-Lussac's Law
Gay-Lussac's law states that the pressure of a gas is directly proportional to its temperature when the volume is held constant. Mathematically, this can be expressed as:
P∝T
or
PT=constant
Step 2: Analyze Each Option
1. Option I: PT=constant
- This directly represents Gay-Lussac's law, as it indicates that the ratio of pressure to temperature remains constant.
2. Option II: P1T2=P2T1
- This equation is a rearrangement of the relationship derived from Gay-Lussac's law. It implies that if the pressure and temperature of a gas change, their product remains constant, which is consistent with Gay-Lussac's law.
3. Option III: P1V1=P2V2
- This equation represents Boyle's law, which states that the pressure of a gas is inversely proportional to its volume when temperature is held constant. This does not represent Gay-Lussac's law.
Step 3: Conclusion
From the analysis:
- Option I and Option II are correct representations of Gay-Lussac's law.
- Option III is incorrect.
Thus, the correct options are I and II.
Final Answer
The correct options that represent Gay-Lussac's law are I and II.
---
Gay-Lussac's law states that the pressure of a gas is directly proportional to its temperature when the volume is held constant. Mathematically, this can be expressed as:
P∝T
or
PT=constant
1. Option I: PT=constant
- This directly represents Gay-Lussac's law, as it indicates that the ratio of pressure to temperature remains constant.
- This equation is a rearrangement of the relationship derived from Gay-Lussac's law. It implies that if the pressure and temperature of a gas change, their product remains constant, which is consistent with Gay-Lussac's law.
- This equation represents Boyle's law, which states that the pressure of a gas is inversely proportional to its volume when temperature is held constant. This does not represent Gay-Lussac's law.
From the analysis:
- Option I and Option II are correct representations of Gay-Lussac's law.
- Option III is incorrect.
The correct options that represent Gay-Lussac's law are I and II.
Topper's Solved these Questions
Similar Questions
Examine the following plots and predict whether in (i) P1<P2andT1>T2 , in (ii) T1=T2<T3, in (iii) V1>V2,in(iv)P1>P2orP2>P1
_E01_267_Q01.png) View Solution
View SolutionFor an ideal monoatomic gas, an illustration of three different paths A,(B+C) and (D+E) from an initial state P1,V1,T1 to a final state P2,V2,T1 is shown in the given figure .
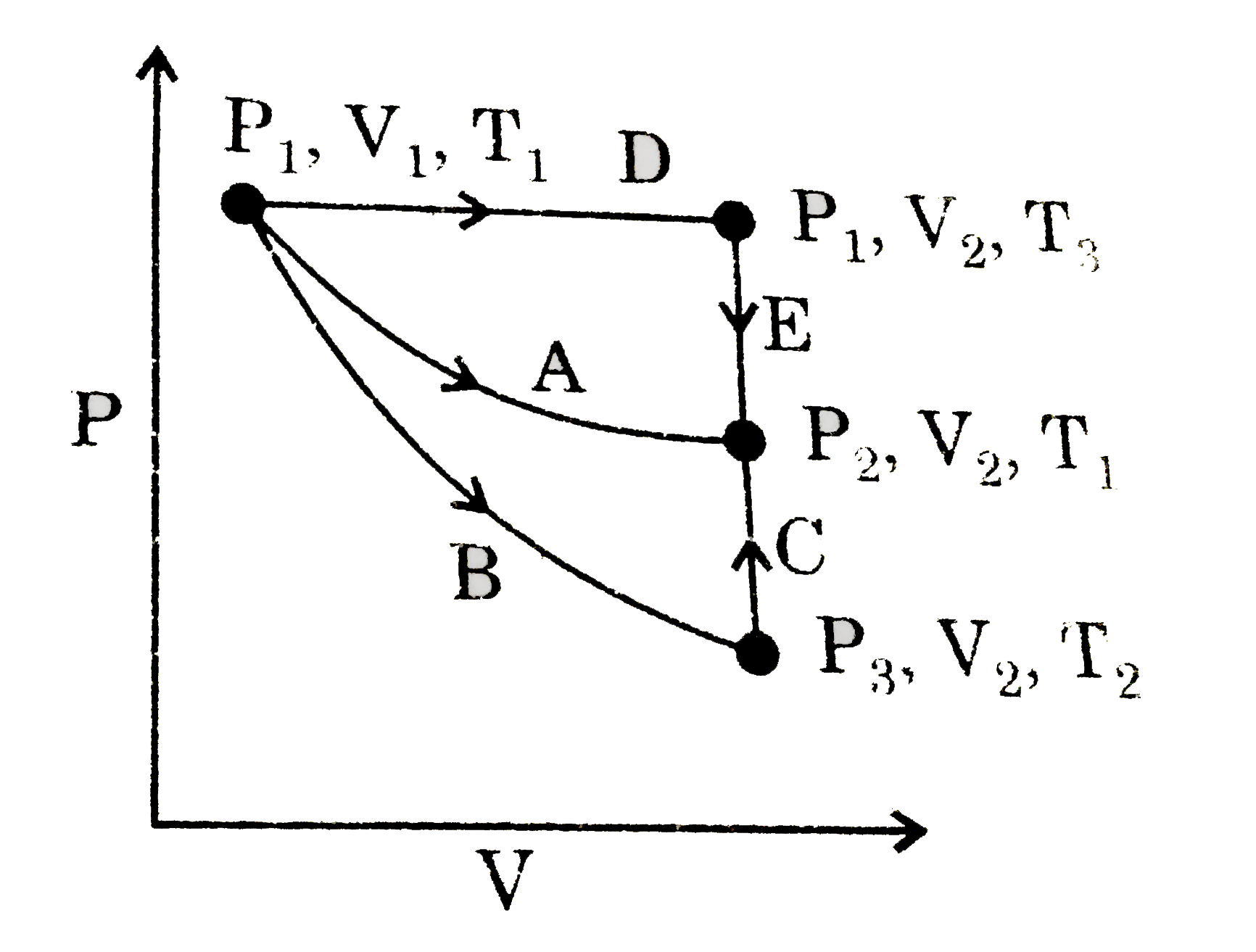
Path A represents a reversible isothermal from P1V1 to P2,V2, path (B+C) represent a reversible adiabatic expansion (B) from P1,V1,T1 to P3,V2,T2 followed by reversible heating of the gas at constant volume (C) from P3,V2,T2 to P2,V2,T1. Path (D+E) represents a reversible expansion at constant pressure P1(D) from P1,V1,T1 to P1,V2,T3 followed by a reversible cooling at constant volume V2(E) form P1,V2,T3 to P2,V2,T1
What is ΔS for (D+E)?View Solution
Knowledge Check
- Question 1 - Select One
P1V1T1=P2V2T2 is -
ABoyle's LawBCombined gas lawCCharles LawDNone of these - Question 2 - Select One
For an ideal gas, an illustration of the different paths, A (B+C) and (D+E) from an intial state P1,V1,T1 to a final state P2,V2,T1 as shown in the given figure.
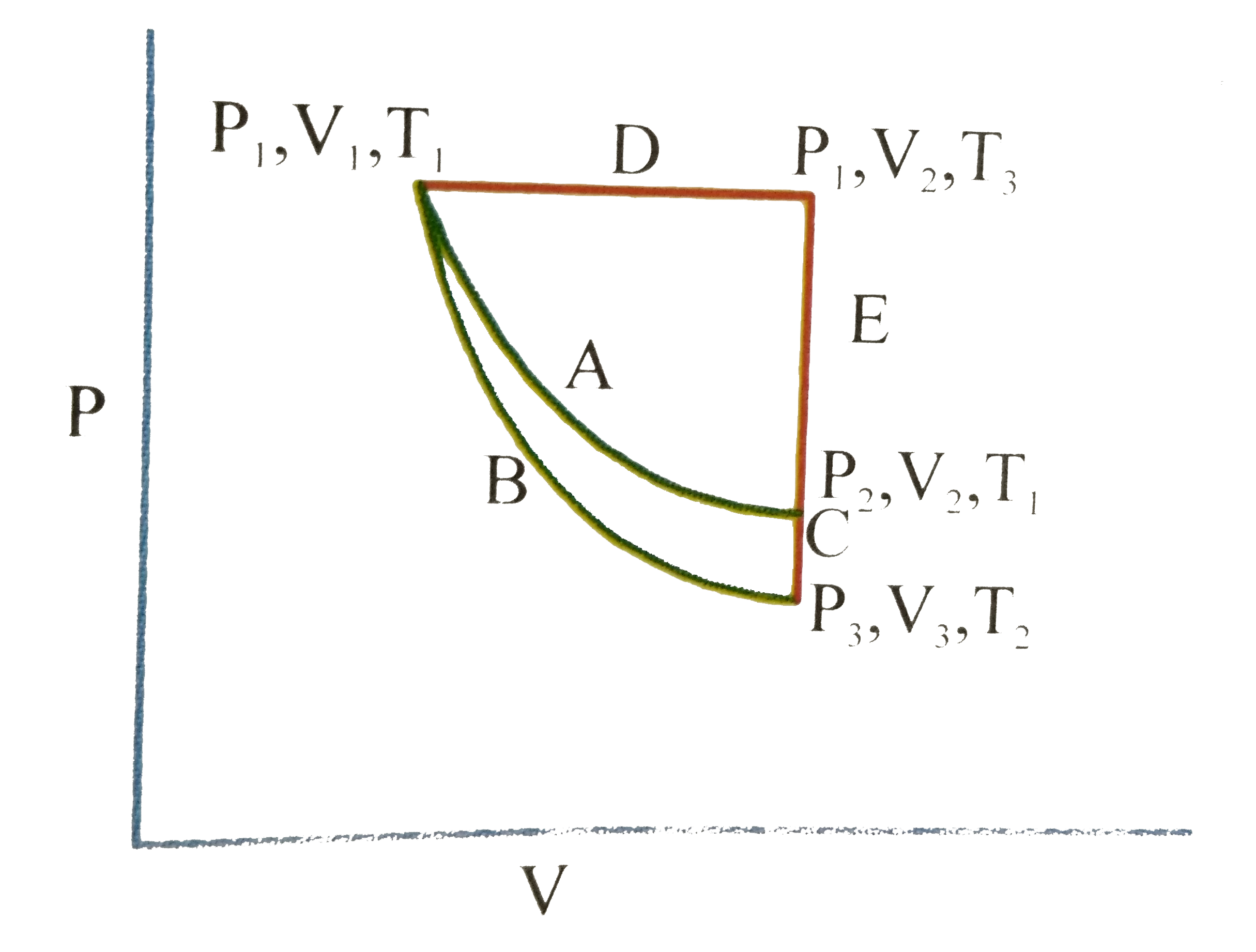
Path (A) represents a reversible isothernal expanison from P1V1 to P2V2. Path (B+C) represents a reversible adiabatic expansion (B) from P1,V1,T1 to P3,V2,T2 followed by reversible heating the gas at constant volume (C) from P3,V2,T2 to P2,V2T1 to P1,V2,T3 followed by reversible cooling at a constant volume V2 (E) from P1,V2,T3 to P2,V2,T1
What is ΔS for path (D+E) ?AzeroBT2∫T1CV(T)TdTC−nRln.V2V1DnRln.V2V1 - Question 3 - Select One
For an ideal monoatomic gas, an illustration of three different paths A,(B+C) and (D+E) from an initial state P1,V1,T1 to a final state P2,V2,T1 is shown in the given figure .
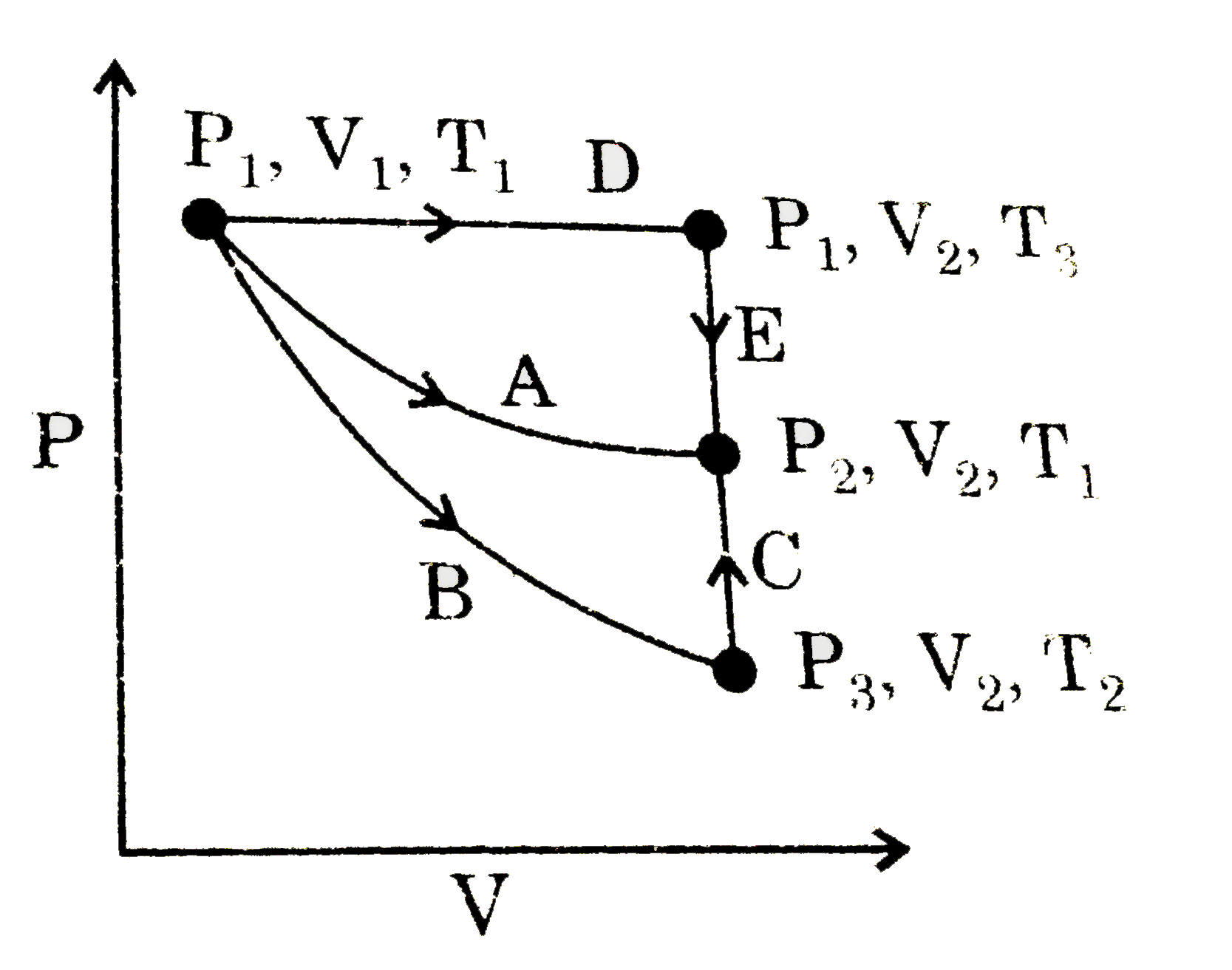
Path A represents a reversible isothermal from P1V1 to P2,V2, path (B+C) represent a reversible adiabatic expansion (B) from P1,V1,T1 to P3,V2,T2 followed by reversible heating of the gas at constant volume (C) from P3,V2,T2 to P2,V2,T1. Path (D+E) represents a reversible expansion at constant pressure P1(D) from P1,V1,T1 to P1,V2,T3 followed by a reversible cooling at constant volume V2(E) form P1,V2,T3 to P2,V2,T1
What is qrev for path (A)?AzeroB−nR InV2V1C−nRT1 InV2V1DnRT1 InV2V1
For an ideal monoatomic gas, an illustration of three different paths A,(B+C) and (D+E) from an initial state P1,V1,T1 to a final state P2,V2,T1 is shown in the given figure .
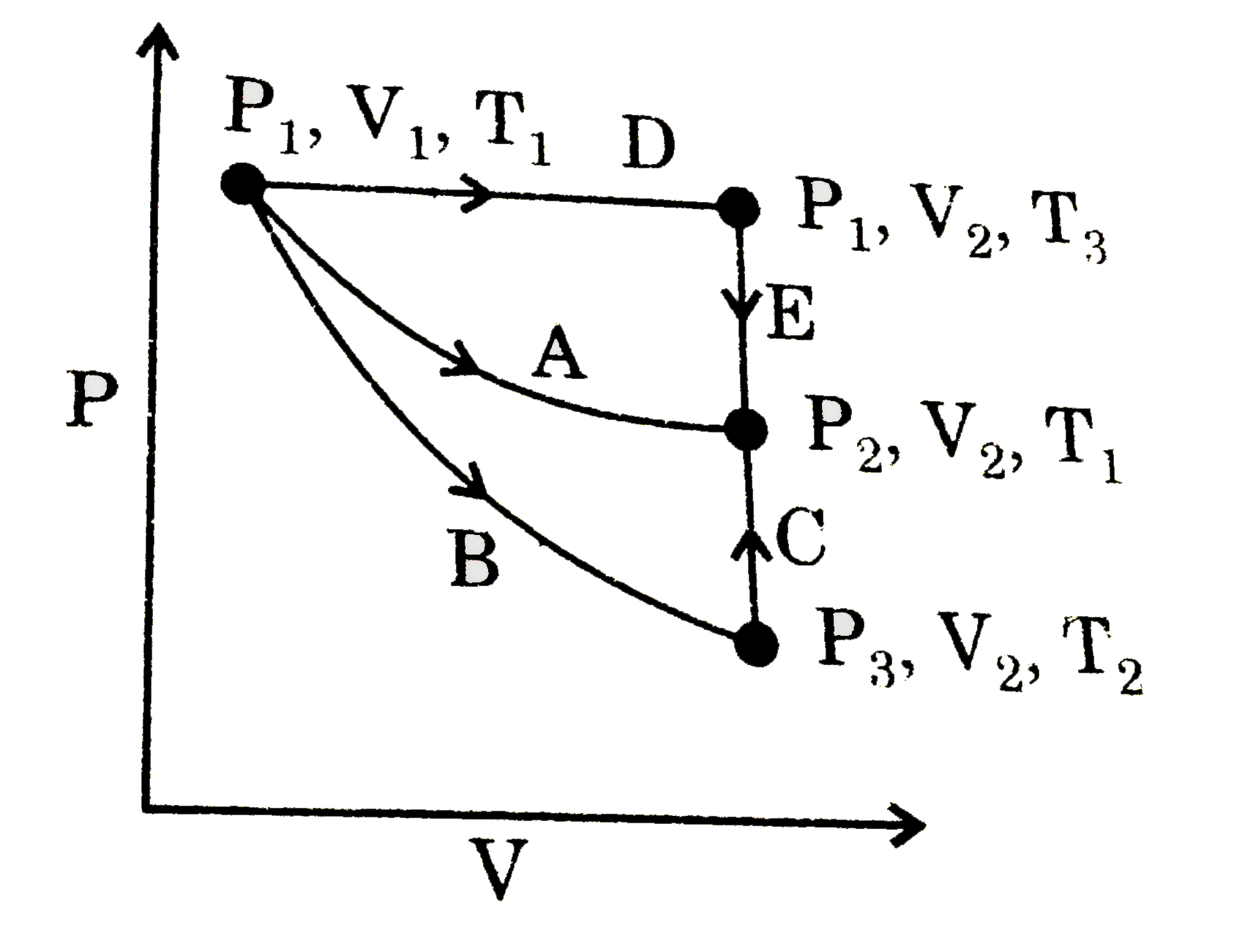
Path A represents a reversible isothermal from P1V1 to P2,V2, path (B+C) represent a reversible adiabatic expansion (B) from P1,V1,T1 to P3,V2,T2 followed by reversible heating of the gas at constant volume (C) from P3,V2,T2 to P2,V2,T1. Path (D+E) represents a reversible expansion at constant pressure P1(D) from P1,V1,T1 to P1,V2,T3 followed by a reversible cooling at constant volume V2(E) form P1,V2,T3 to P2,V2,T1
What is ΔS for path (A)?View SolutionFor an ideal gas, an illustratio of three different paths A(B+C) and (D+E) from an initial state P1,V1,T1 to a final state P2,V2,T1 is shown in the given figure.
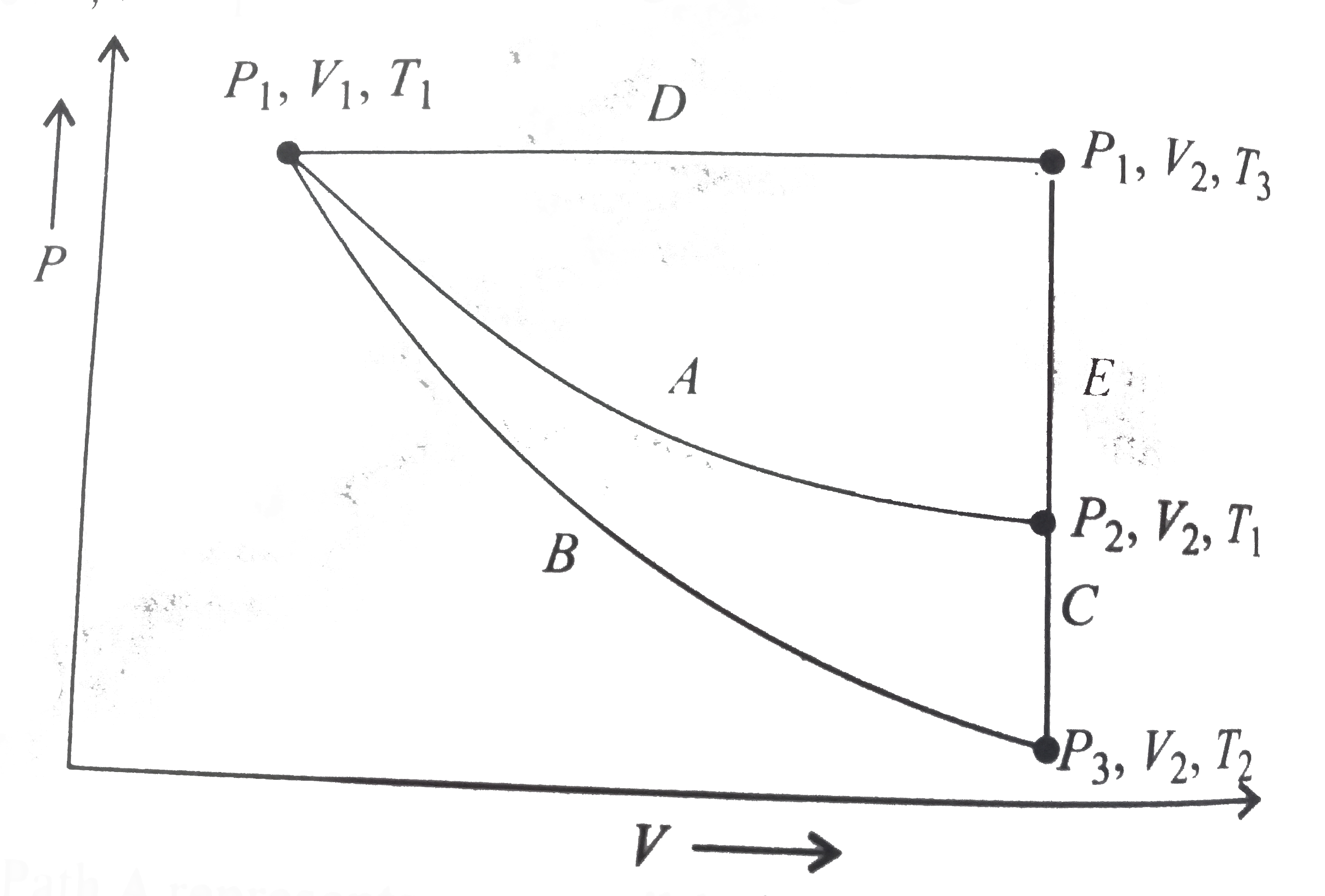
Path Arepresents a reversible isothermal expansion form P1,V1 to P2,V2, Path (B+C) represents a reversible adiabatic expansion (B) from P1,V1,T1→P3,V2,T2 followed by reversible heating the gas at constant volume (C)from P3,V2,T2 to P2,V2,T1. Path (D+E) represents a reversible expansion at constant pressure P1(D) from P1,V1,T1 to P1,V2,T3 followed by a reversible cooling at constant volume V2(E) from P1,V2,T3→P2,V2,T1.
What is qrev, for path (A)?View SolutionFor an ideal gas, an illustratio of three different paths A(B+C) and (D+E) from an initial state P1,V1,T1 to a final state P2,V2,T1 is shown in the given figure.
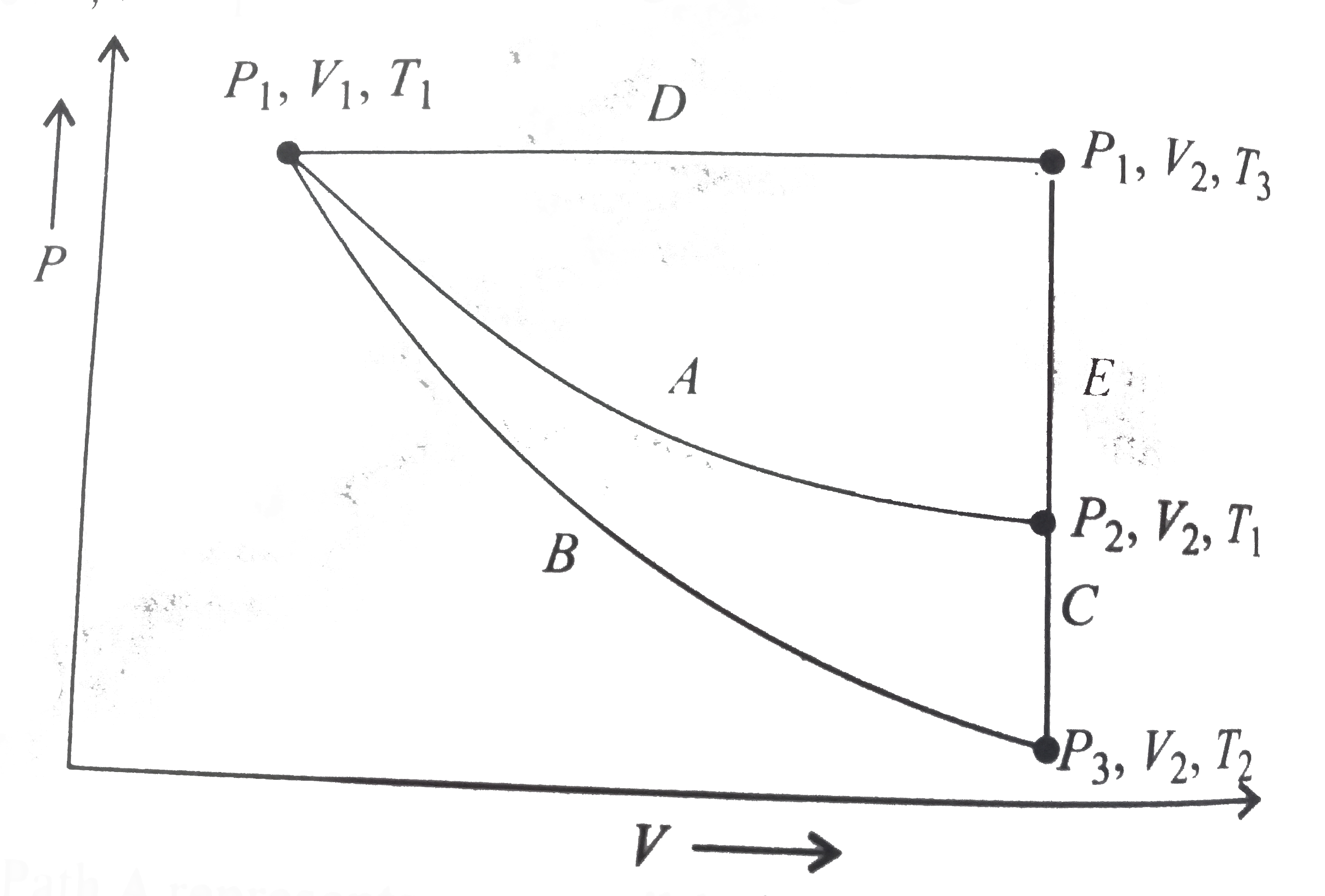
Path Arepresents a reversible isothermal expansion form P1,V1 to P2,V2, Path (B+C) represents a reversible adiabatic expansion (B) from P1,V1,T1→P3,V2,T2 followed by reversible heating the gas at constant volume (C)from P3,V2,T2 to P2,V2,T1. Path (D+E) represents a reversible expansion at constant pressure P1(D) from P1,V1,T1 to P1,V2,T3 followed by a reversible cooling at constant volume V2(E) from P1,V2,T3→P2,V2,T1.
What is qrev, for path (D+E)?View SolutionFor an ideal gas, an illustratio of three different paths A(B+C) and (D+E) from an initial state P1,V1,T1 to a final state P2,V2,T1 is shown in the given figure.
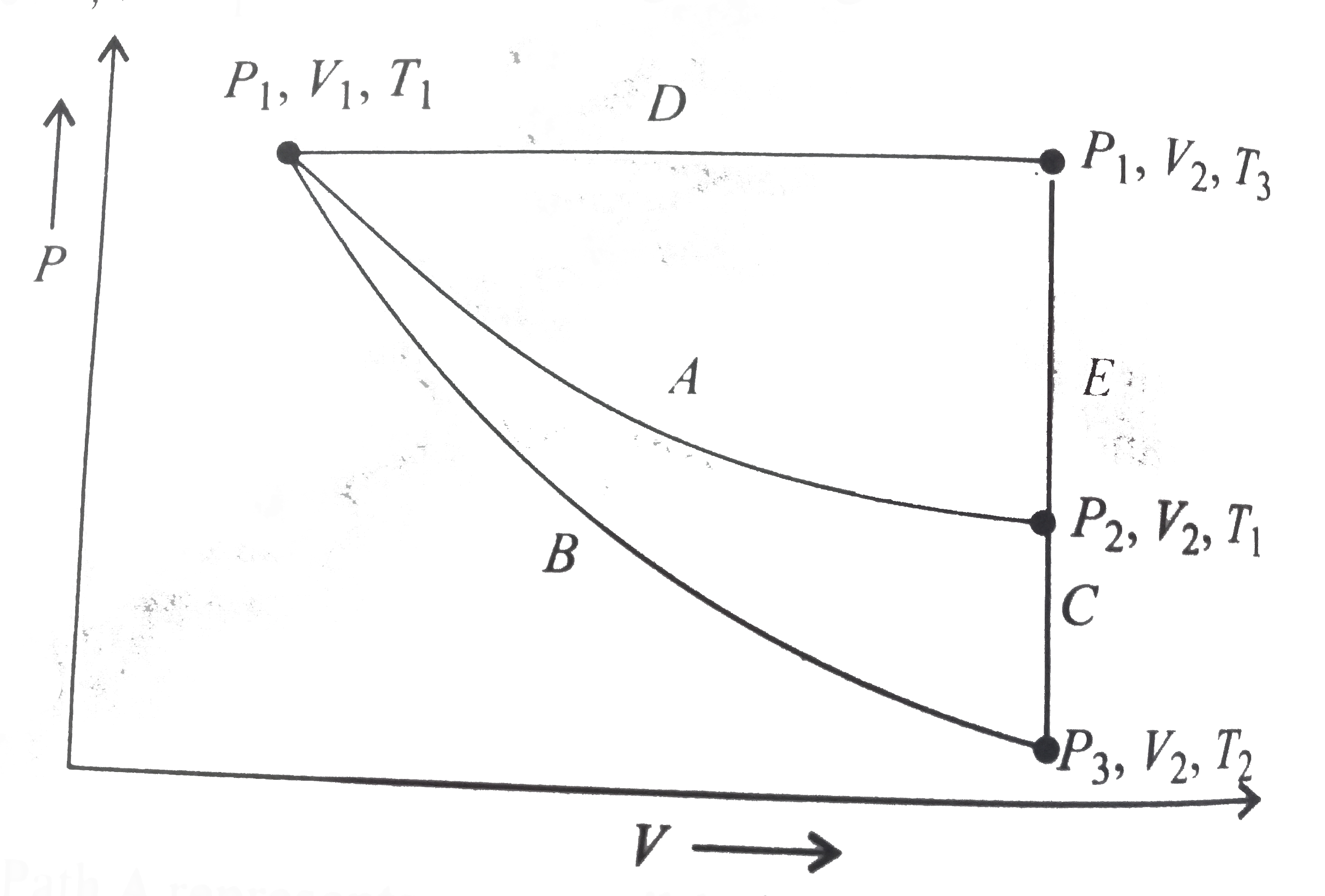
Path Arepresents a reversible isothermal expansion form P1,V1 to P2,V2, Path (B+C) represents a reversible adiabatic expansion (B) from P1,V1,T1→P3,V2,T2 followed by reversible heating the gas at constant volume (C)from P3,V2,T2 to P2,V2,T1. Path (D+E) represents a reversible expansion at constant pressure P1(D) from P1,V1,T1 to P1,V2,T3 followed by a reversible cooling at constant volume V2(E) from P1,V2,T3→P2,V2,T1.
What is ΔS for path A?View SolutionWhich of the following relationships between partial pressure , volume and temperature is correct ?
(i) P=nRTV
(ii) Ptotal=P1+P2+P3
(iii) Ptotal=(n1+n2+n3)RTVView Solution

