A uniform wire of resistance 12Ωis cut into three pieces in the ratio 1 : 2 : 3 and the three pieces are connected to form a triangle. A cell of emf 8 V and internal resistance1Ωis connected across the highest of the three resistors. Calculate the current through each part of the circuit.Solution in Punjabi
Step by step video & image solution for A uniform wire of resistance 12 Omega is cut into three pieces in the ratio 1 : 2 : 3 and the three pieces are connected to form a triangle. A cell of emf 8 V and internal resistance 1 Omega is connected across the highest of the three resistors. Calculate the current through each part of the circuit. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- ELECTRIC CURRENT. RESISTANCE AND E.M.FBOOK - MODERN PUBLICATIONCHAPTER - ELECTRIC CURRENT. RESISTANCE AND E.M.FEXERCISE - EXERCISE
64 Videos
- DISPERSIONBOOK - MODERN PUBLICATIONCHAPTER - DISPERSIONEXERCISE - EXERCISE
54 Videos
- ELECTRIC FIELDBOOK - MODERN PUBLICATIONCHAPTER - ELECTRIC FIELDEXERCISE - EXERCISE
95 Videos
Similar Questions
What is drift velocity of electrons? How do you explain the flow of current in a conductor based on this?
View SolutionFor a prism prove that
A+δ=i+e
View SolutionIn defining electric field due to a point charge, the test charge has to be vanishingly small. How this condition can be justified, when we know that charge less than the on an electron or a proton is not possible?
View SolutionA uniform wire of resistance 9Ω is cut into 3 equal parts. They are connected in form of equilateral triangle ABC. A cell of e.m.f. 2V and negligible internal resistance is connected across B and C. Potential difference across AB is
View SolutionA uniform wire of resistance 12 Omega is cut into three pieces in the ratio 1 : 2 : 3 and the three pieces are connected to form a triangle. A cell of emf 8 V and internal resistance 1 Omega is connected across the highest of the three resistors. Calculate the current through each part of the circuit.
View SolutionThree identical, each of emf 2 V and internal resistance 0.2Ω are connected in series to an external resistor of 7.4Ω. Calculate the current in the circuit.
View SolutionThree identical cells each of emf 2 V and unknown internal resistance are connected in parallel. This combination is connected to a 5 Omega resistor. If the terminal voltage across the cells is 1.5 V, what is the internal resistance of each cell ?
View SolutionA battery of emf 3 V and internal resistance 1Ω is connected in parallel with a cell of emf 1.5 V and internal resistance 0.5Ω with their like poles together. The combination is used to pass a current through a resistor of resistance of 5Ω. Using Kirchhoffs circuital laws, find the current through the 5Ω resistor.
View SolutionA series combination of three resistances 1Ω,2Ω and 3Ω is connected with a cell of emf 1.5 V and of negligible internal resistance. What is the terminal potential difference across the third resistance?
View SolutionAn electric cell of emf 10 V and of internal resistance 1Ω is connected in series with the parallel combination of three resistances of 3Ω,5Ω and 8Ω.Calculate the currents through the three resistances.
View SolutionA parallel combination of three resistances of 6Ω,12Ωand12Ω is connected in series with an electric cell of emf 1.5 V and internal resistance2Ω. Find out the currents through the three resistances.
View SolutionThree resistances of 4Ω,5Ω and 12Ω are connected in parallel.A battery of emf 10 v and internal resistance 0.5Ω. Is connected with that combination .Find out the currents through this battery and through each of this three resistances.
View SolutionThree resistors 1Omega, 2 Omega and 3 Omega are connected to form a triangle. Across 3Omega a 3 V battery is connected. The current through 3Omega resistor is
View SolutionA wire of uniform cross section of resistance 16Ω is divided into four pieces in the ratio of 1 : 2 : 3 : 4 and ll the four resistros are connected in the form of a square . Calculate the current drawn from the battery, if a battery of emf 10 V and internal resistance 0.5Ω is connected across the resistor with least resistance.
View SolutionThree identical cells of emf 2 V and internal resistances 0.20Ω are connected in series. The combination is further connected to an external resistor of 6Ω . Calculate the current through 6Ω resistor.
View SolutionA uniform wire of resistance 12Ω is cut into three pieces so that the ratio of the resistances R1:R2:R3=1:2:3 and the three pieces are connected to form a triangle across which a cell of emf 8V and internal resistance 1Ω is connected as shown.
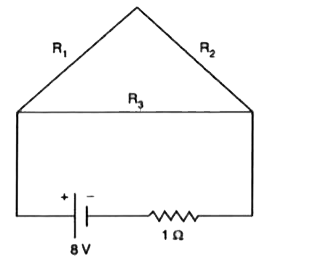
Calculate the current through each part of the circuit.
View Solution